Group Theory and Symmetry, Part IV: Great or Grand, We've Got GOT

In the last installment about group theory (1), I introduced character tables as the simplest representation of the behavior of the symmetry operations of a group. All well and good, but how are they used?

Reducing a Reducible Representation

It is not unusual in applying group theory to come across a set of characters in a particular point group that do not belong to one of the irreducible representations. One such set of characters in a system with C_2v symmetry is shown in Figure 1, where we are using "Γ" as the general symbol for a reducible representation. How do we know that this representation is reducible? Easy, we just look at the character table for C_2v (Figure 2) and note that this set of numbers is not listed in the character table. Because it's not an irreducible representation, it must be a reducible one. (NB: Any representation in any point group will either be irreducible or reducible. If it is not, then it has been constructed incorrectly.)

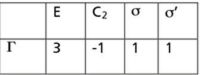
Figure 1: Set of characters with C2v symmetry.

We need to separate this reducible representation into its irreducible components. This is the equivalent of denoting any point in three-dimensional space as so many x, so many y, and so many z. Only in this case, our "basis" is not composed of x, y, and z; it's composed of the irreducible representations of the point group. In this case, we would write the appropriate combination as

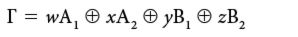
where w, x, y, and z represent the number of each irreducible representation in the reducible one, and the symbol "⊕" means "direct sum." A direct sum is the sum of the characters of each symmetry element to generate a new set of characters. Characters from different symmetry elements are never combined. Separating a reducible representation into its irreducible counterparts is called, naturally, reducing the reducible representation.

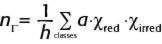
We might be able to reduce it by inspection; that is, figure it out by looking at Figure 2 and maybe do a little bit of trial and error. That may work for low-magnitude reducible representations, but it's much more difficult for larger ones. What we really need is a systematic way to reduce a representation.

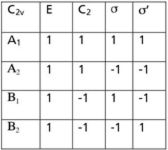
Figure 2: Character table for the C2v point group.

Enter the GOT

The standard way to reduce a reducible representation is to use the Great Orthogonality Theorem, sometimes also known as the Grand Orthogonality Theorem but in either case abbreviated the GOT (2): where n_Γ is the number of times a certain irreducible representation appears in a reducible one; h is the number of symmetry elements in the point group (known as the order of the group); a is the number of symmetry elements in each column of the point group, which are known as classes; χ_red is the character of the reducible representation, and χ_irred is the character of that irreducible representation. This expression is evaluated for each irreducible representation in the character table, and the n_Γ values are always integers ranging between zero and the highest character in the reducible representation (usually the character for E, the identity element). If it's not an integer, an error has been made. The GOT is one form of the Schur orthogonality relations, which are some general orthogonality relationships for mathematical groups and are named after Issai Schur, an Eastern European mathematician who specialized in mathematical groups. (I say "Eastern European" because he was born in what is now Belarus, grew up in what is now Latvia, and was educated and worked in Germany.)

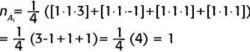
Let us see how the GOT works by reducing the reducible representation above. To determine how many times A₁ appears in our reducible representation, we combine the characters of A₁ with the characters from the reducible representation, multiply by the number of symmetry elements in each class (which, from Figure 2, is just 1 for each symmetry element), and sum over all classes of symmetry elements before dividing by the order of the group, which is 4:

The numbers inside the square brackets are, respectively, the number of elements in each class (each column of the C_2v point group only has one symmetry element), the character of each class for A₁, and the respective character in each class from Γ. Determining the n_Γ for the other three irreducible representations in Γ:

So, this irreducible representation reduces into a combination of 1 A₁, B₁, and 1 B₂ irreducible representations. We write this as this direct sum:

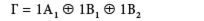
We have reduced this reducible representation into its irreducible equivalent.

Applications of the GOT

There are several applications of the GOT in the chemical sciences (2,3). Here I will focus on two: the symmetry descriptions of molecular vibrations and the partial determination of the value of an integral.

A molecule with N atoms has 3N possible net motions of its nuclei (each nucleus can move in three dimensions, hence the 3N factor). Of these 3N motions, three are the translations of the molecule as a whole and three are the rotations of the molecule about its center of mass (two, if the molecule is linear). The remaining 3N – 6 (3N – 5 if linear) motions are movements of the atoms of the molecule that do not move the center of mass of the molecule. They are called vibrations.

The random possible motions of the vibrations of a molecule can be broken down (dare I say, reduced) into fundamental motions called the normal modes of vibration. It's the normal modes of vibration that are measured when a vibrational spectrum is measured. The normal modes of vibration represent the most basic description of the vibrations of a polyatomic molecule. (Individual atoms don't have vibrations; in the solid state, the motions of the particles with respect to other particles, whether they be atoms or molecules, are called phonon modes and are considered separately from the vibrations.)

When the symmetry of a molecule is determined, the nuclear motions of the atoms that make up the vibration are ignored; however, each vibration works to distort the apparent symmetry of the molecule. On average, however, the molecule still has the symmetry as determined by non-moving nuclei. The possible motions of the molecule, however, can be used to generate a set of characters that describe the 3N motions under that symmetry point group. This character set can be reduced using the GOT. Of the set of irreducible representations, three of them correspond to the translations, and three (or two, if linear) correspond to the rotations. The remaining 3N – 6 (or 3N – 5) irreducible representations stand for the vibrations. Each vibration of the molecule can be labeled with an irreducible representation of the point group.

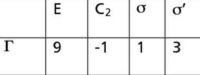
Figure 3: Reducible character set for a C2v molecule that has three atoms.

There are various standard recipes for generating the character set for the motions of any given molecule (see references 2–4). In most cases, the set of characters is reducible but is difficult to reduce by inspection (except maybe for two- or three-atom molecules). Thus, the GOT must be used to reduce it and to remove the translational and rotational parts.

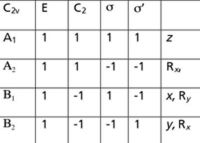
Figure 4: An expanded character table for the C2v point group.

For example, a C_2v molecule that has three atoms (like H₂O) gets the reducible character set shown in Figure 3. Can you reduce this by inspection? Likely not (no offense intended!). However, using the GOT, we can determine that this reducible representation reduces to:

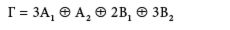
To remove the translational and rotational motions, we need additional information. This information is usually found in an expanded character table, shown in Figure 4. The "R" entries list the irreducible representations that are assigned to rotations in C_2v, while the x, y, and z entries list the irreducible representations that are assigned to the translations in C_2v. Thus, an A₂, a B₁, and a B₂ must be removed from Γ to account for the rotations, and an A₁, a B₁, and a B₂ irreducible representation must be removed to account for the translations. What is left over are the irreducible representations that must be assigned to the normal modes of vibration. We have

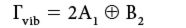
Thus, the three normal modes of vibration are assigned two A₁ labels and one B₂ label. For small molecules it is easy to assign these labels by inspection, as the characters of each irreducible representation represent eigenvalues of the motion vectors as the symmetry operation is applied the individual vibration. For larger molecules, a more detailed approach is necessary to assign labels to the vibrational modes; the details of doing this are beyond our scope but can be found in some classic books (5).

The second use of the GOT comes with evaluating transition moments. In spectroscopy, a transition moment M is defined as

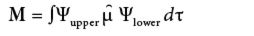
where Ψ_upper is the wavefunction of the upper state, Ψ_lower is the wavefunction of the lower state, and µ is the dipole moment operator. In a system of a given symmetry, the two wavefunctions and the operator can be labeled by irreducible representations, just like vibrations are. The overall set of characters for the integrand is the product of the irreducible representation's characters of the three functions in the integrand:

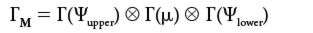
The "⊕" symbol now represents the direct product in which the characters from each symmetry element are multiplied by each other (rather than added, as we found in the direct sum). The multiplication of characters generates a new character set that is the representation of the integrand as a whole.

A transition moment is a number. It may be zero, it may be nonzero. However, for it to be nonzero, Γ_M must be or contain (by reduction by the GOT) the all-symmetric irreducible representation of that point group. The all-symmetric irreducible representation is the one that contains all ones as characters; it is typically labeled A₁ but may be labeled as "A-something else" in some character tables. It is always listed first in a character table.

Why is this so? Because if the transition moment is nonzero, it has some finite value, and it is not a vector. A numerical value must be invariant to any possible symmetry operation, which means its eigenvalue for any symmetry operation must be 1. This means that, if you are taking the character as an eigenvalue, the symmetry of the integrand must include the all-symmetric irreducible representation as part of its character set. Sometimes you can tell by inspection, but in other cases you have to use the GOT to reduce the reducible representation, just like we did for the vibrations above. If A₁ (or however the all-symmetric irreducible representation) is NOT part of Γ_M, then the integral must equal to zero. Only if A₁ is part of Γ_M can the integral be nonzero (however, the symmetry considerations give no hint about how nonzero the value will be).

These irreducible representation-based conclusions are the basis for selection rules. In cases where A₁ is not present, the spectroscopic transition is predicted to not occur and is considered forbidden. If A₁ is present, then the spectroscopic transition is allowed. Knowing even approximate wavefunctions allows us to determine what selection rules will be, typically in terms of the change of the relevant quantum number.

I hope you have enjoyed our multi-part foray into symmetry and group theory. It is a fascinating subject, one that all spectroscopists should be familiar with. Hopefully this series has inspired you to look further into a field of mathematics that is very important in spectroscopy.

David W. Ball is a professor of chemistry at Cleveland State University in Ohio. Many of his "Baseline" columns have been reprinted in book form by SPIE Press as The Basics of Spectroscopy, available through the SPIE Web Bookstore at www.spie.org. His book Field Guide to Spectroscopy was published in May 2006 and is available from SPIE Press. He can be reached at d.ball@csuohio.edu; website: academic.csuohio.edu/ball

David W. Ball

References

(1) D.W. Ball, Spectroscopy24 (4), 16–21 (2010).

(2) R.L. Carter, Molecular Symmetry and Group Theory (John Wiley & Sons, New York, 1998).

(3) F.A. Cotton. Chemical Applications of Group Theory, 3d ed. (Wiley-Interscience, New York, 1990).

(4) D.W. Ball. Physical Chemistry (Cengage Publishing Company, Belmont, California, 2002).

(5) E.B. Wilson, J.C. Decius, and P.C. Cross, Molecular Vibrations (Dover Publications, Mineola New York, 1980).