Maxwell's Equations, Part VI

This is the sixth installment in a series devoted to explaining Maxwell's equations, the four mathematical statements upon which the classical theory of electromagnetic fields — and light — is based. Previous installments can be found on Spectroscopy's website (www.spectroscopyonline.com/The+Baseline+Column). Maxwell's equations are expressed in the language of vector calculus, so a significant part of some previous columns have been devoted to explaining vector calculus, not spectroscopy. In the last installment, we were developing the vector calculus tools to express Maxwell's equations. An understanding of the math makes it easier to understand the equations — that's why we're spending so much time on math, rather than spectroscopy. Apologies if this frightens or dismays you, but that's the way it goes. Once again, the numbering of the figures is intentional; it's meant to provide continuity throughout this entire series.

Where We Left Off

In our last installment, we left off with an expression for the infinitesimal work per unit area in a rectangular field in the (x,y) plane. The overall vector field is given by the equation

and we were assuming a closed path in just the aforementioned plane. We found that the infinitesimal work was

Thus, the closed loop in the (x,y) plane actually related to a vector in the z direction. The integral over the closed path is also referred to as the circulation of the vector field.

Now we are ready to generalize our result and consider how it applies to an equation — specifically, one of Maxwell's equations.

Introducing the Curl

For a vector function F = F_x i + F_y j + F_z k, I will hereby define the function

as the one-dimensional curl of F. I designate it "one dimensional", possibly improperly, because the result is a vector in one dimension, in this case the z dimension. The analysis we performed in the earlier section — defining a closed path in a single plane and taking the limit of the path integral — can be performed for the (x,z) and (y,z) planes. When we do that, we get the following analogous results:

The combination of all three expressions gives us a general expression for the curl ofF:

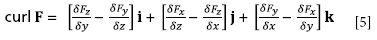
This expression allows us to determine

for any vector function F in any plane.

But what does the curl of a vector function mean? One way of thinking about it is that it is a variation in the vector function F that causes a rotational effect in a perpendicular axis. (Indeed, "curl F" is sometimes still designated "rot F," and a vector function whose curl equals zero [see Figure 38] is termed "irrotational.") Furthermore, a vector function with a nonzero curl can be thought of as curving around a particular axis, with that axis being normal to the plane of the curve. Thus, the rotating water in Figure 37 of our previous installment has a nonzero curl, while the linearly flowing water in Figure 38 has a zero curl. You may want to refer to the previous installment of this column to refresh your memory of what they look like (1).

A mnemonic (that is, a memory aid) for the general expression for curl F takes advantage of the structure of a 3 × 3 determinant:

Understand that curl F is not a determinant; a determinant is a number that is a characteristic of a square matrix of numerical values. However, the expression for curl F can be constructed by performing the same operations on the expressions as one would do with numbers to determine the value of a 3 × 3 determinant: constructing the diagonals and adding the right-downward diagonals and subtracting the left-upwards diagonals. If you have forgotten how, Figure 39 shows how to determine the expression for the curl.

Figure 39

The determinantal form of the curl can be expressed in terms of the del operator, ∇. Recall from part III of this series (2) that the del operator is

Also recall from vector calculus that the cross product of two vectors A ≡ i A _x + j A _y + k A _z and B defined analogously is written A × B and is given by the expression

By comparing this expression to the determinantal form of the curl, it should be easy to see that the curl of a vector function F can be written as

Like the fact that curl is not technically a determinant, it is technically not a cross product, as del is an operator, not a vector. The parallels, however, make it easy to gloss over this technicality and use the "del cross F" symbolism to represent the curl of a vector function.

Because the work integral over a closed path through an electrostatic field E is zero, it is a short, logical step to state that therefore

This is one more property of an electrostatic field: The field is not rotating about any point in space. Rather, an electrostatic field is purely radial, with all field "lines" going from the point in space straight to the electric charge.

Faraday's Law

An electrostatic field caused by a charged particle is thought of as beginning at a positive charge and ending at a negative charge. Because overall matter is electrically neutral, then every electric field emanating from a positive charge eventually ends at a negative charge. This is illustrated in Figure 40, top diagram.

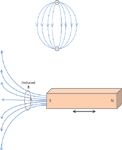
Figure 40

However, when a changing magnetic field creates a current in a conductor, this current is the product of an induced electric field. In the case of a bar-type magnet, the magnetic field is axially symmetric about the length of the bar, so the induced electric field is axially symmetric as well: that is, it is circular. This is illustrated in Figure 40, bottom diagram.

The induced, circular electric field caused by a moving magnet causes charges to move in that circle. The circulation of the induced electric field vector can be constructed from our definition of "circulation" above; it is

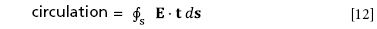
where E is the induced electric field, t is the tangent vector along the path, and s is the infinitesimal amount of path. In this case, the "circulation" is defined as the "electromotive force," or emf. What is this force doing? Why, causing charges to move, of course! As such, it is doing work, and our arguments using work in the sections above are all valid here.

What Faraday found experimentally is that a changing magnetic field induced an electric field (which then forced a current). If you imagine that a magnetic field is composed of discrete field lines, what is happening is that as the number of magnetic field lines in a given area changes with time, an electric field is induced. Figure 41 illustrates this. Consider the loop of area outlined by the black line. As the magnet is moved to the right, the number of magnetic field lines intersecting the loop changes. It is this change that induces the electric field.

Figure 41

The number of field lines per area is called the magnetic flux. In part III of this series (2), we presented how we determine the flux of a vector field. For a changing vector field F having a unit vector perpendicular (or normal) to its direction of motion n over some surface S, the flux is defined as

For our magnetic field B, this becomes

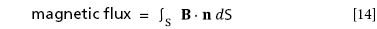
But the induced electric field is related to the change in magnetic flux with time. Thus, we are actually interested in the time derivative of the magnetic flux:

At this stage, we bring everything together by citing the experimental facts as determined by Faraday and others: The electromotive force is equal to the change in the magnetic flux over time. That is,

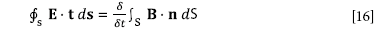
Let us divide each side of this equation by the area A of the circular path of the induced current. This area also corresponds to the surface S that the magnetic field flux is measured over, so we divide one side by A and one side by S. We get

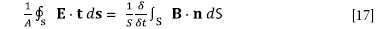
Suppose we want to consider the limit of this expression as the area of the paths shrink to zero size; that is, as A → 0. We would have

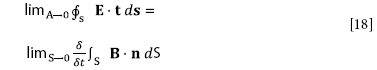
The left side is, by definition, the curl of E. What about the right side? Rather than prove it mathematically, let's consider the following argument. As the surface S goes to zero, the limit of the magnetic flux ultimately becomes one magnetic flux line. This single line will be perpendicular to the infinitesimal surface — look at the rendering of the magnetic field lines in Figure 41 if you need to convince yourself of this. Thus, the dot product B · n is simply B, and the infinite sum of infinitesimal pieces (which is what an integral is) degenerates to a single value of B. I, therefore, argue that

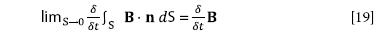
So what we now have is

We are almost done. The law of conservation of energy must be satisfied. Although it appears that we are getting an induced current from nowhere, understand that this induced current also generates a magnetic field. For the law of conservation of energy to be satisfied, the new magnetic flux must oppose the original magnetic flux (this concept is known as Lenz's law after Henrich Lenz, the Russian physicist who discovered it). To represent this mathematically, a negative sign must be included in the last equation. By convention, the minus sign is put on the right side, so our final equation is

This expression is known as Faraday's law of induction, given that Michael Faraday discovered (or rather, first announced) magnetic induction of current. It is considered the third of Maxwell's equations: a changing magnetic field induces an electromotive force, which in a conductor will promote a current.

Not meaning to minimize the importance of Maxwell's other equations, but the impact of what this equation embodies is huge. Electric motors, electrical generators, and transformers are all direct applications of a changing magnetic field being related to an electromotive force. Given the electrified nature of modern society, and the machines that make it that way, we realize that there is a huge impact of Maxwell's equations in our everyday lives.

David W. Ball is normally a professor of chemistry at Cleveland State University in Ohio. For a while, though, things will not be normal: starting in July 2011 and for the commencing academic year, David will be serving as Distinguished Visiting Professor at the United States Air Force Academy in Colorado Springs, Colorado, where he will be teaching chemistry to Air Force cadets. He still, however, has two books on spectroscopy available through SPIE Press, and just recently published two new textbooks with Flat World Knowledge. Despite his relocation, he still can be contacted at d.ball@csuohio.edu. And finally, while at USAFA he will still be working on this series, destined to become another book at an SPIE Press web page near you.

David W. Ball

References

(1) D.W. Ball, Spectroscopy 27(1), 24–33 (2012).

(2) D.W. Ball, Spectroscopy 26(9), 18–27 (2011).

(3) Other references: In writing this series, I have been strongly influenced by the following works:

• H. M. Schey, Div, Grad, Curl, and All That: An Informal Text on Vector Calculus (W.W. Norton and Company, New York, New York, 2005). This is a wonderful text for someone needing the fundamentals of vector calculus. It is engaging and light-hearted, two adjectives that you would swear would never be used in a description of vector calculus!

• D. Fleisch, A Student's Guide to Maxwell's Equations (Cambridge University Press, Cambridge, UK, 2008). This very approachable book takes the tactic of parsing each equation and explaining what each part means; very useful in understanding the fundamentals of Maxwell's equations.