What Modeling Reveals About the Properties of an Inductively Coupled Plasma
To get better performance from inductively coupled plasma (ICP)-based methods, it is informative to study the properties of the ICP under different conditions. Annemie Bogaerts and Maryam Aghaei at the University of Antwerp, Belgium, are using computational modeling to examine how various properties of the ICP, such as gas flow path lines and velocity, temperature changes, and ionization effects, are affected by numerous factors, such as the gas flow rates of injector and auxiliary gas, applied power, and even the very presence of an MS sampler. They have also applied their models to study particle transport through the ICP. Using their developed model, it is now possible to predict optimum conditions for specific analyses. Bogaerts and Aghaei spoke to us about this work.
Pages 52–59
To get better performance from inductively coupled plasma (ICP)-based methods, it is informative to study the properties of the ICP under different conditions. Annemie Bogaerts and Maryam Aghaei at the University of Antwerp, Belgium, are using computational modeling to examine how various properties of the ICP, such as gas flow path lines and velocity, temperature changes, and ionization effects, are affected by numerous factors, such as the gas flow rates of injector and auxiliary gas, applied power, and even the very presence of a mass spectrometry (MS) sampler. They have also applied their models to study particle transport through the ICP. Using their developed model, it is now possible to predict optimum conditions for specific analyses. Bogaerts and Aghaei spoke to us about this work.
You carried out a computational study to examine the impact on an inductively coupled plasma that arises from inserting the metal cone interface of a mass spectrometer sampler (1). You have also studied the effect of sampling cone position and sampler orifice diameter (2). Can you briefly explain how your model works?
The ICP-MS model is based on solving partial differential equations for the gas flow dynamics, coupled with the energy conservation equation and Maxwell equations. The power coupling into the ICP is a source term in the energy conservation equation, whereas the emitted radiation is treated as a loss term. The electromagnetic fields, the amount of ionization, and the material parameters as a function of temperature and plasma properties also are calculated. Typical calculation results from the model include the gas flow behavior, as well as the plasma temperature and electron density profiles.
What did your study show about how the gas flow path lines and velocity in an ICP are affected by the presence of an MS sampler, as well as its positioning and orifice diameter?
The calculation results indicate that the plasma velocity increases dramatically when flowing through the sampling orifice, as a result of the sudden pressure drop (Figure 1). Moreover, the flow coming from the central inlet, instead of maintaining a straight line trajectory, adopts a curved flow profile because of the entrainment forces acting upon it. Nevertheless, the whole carrier-gas flow is able to pass through the interface cone as a consequence of flow acceleration to the orifice of the sampler. However, the auxiliary and cooling gas flows are not affected by the acceleration toward the orifice, and they adopt a curved line trajectory to the open sides of the torch at the end sides of the interface cone. This modification of path lines affects the temperature and electron density profiles. Moreover, by changing the sampler orifice diameter, we can control whether only the carrier gas or also the auxiliary gas can exit through the sampler. However, the flow velocity at the sampler position is found to be independent of the sampler orifice diameter.
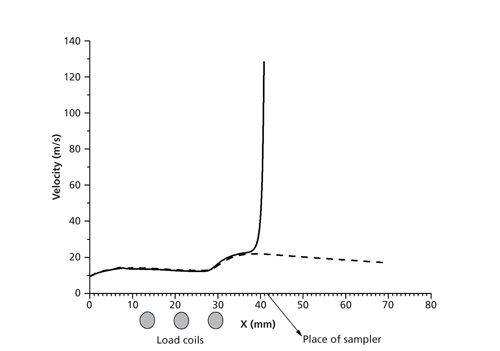
Figure 1: Axial distribution of gas flow velocity in the case with (solid line) and without (dashed line) sampler, on the central axis of the plasma torch. The load coils and the place of the sampler are also indicated for clarity. (Adapted with permission from the Royal Society of Chemistry, reference 1).
What was the effect on the plasma temperature and electron density?
The sampler cools the gas flowing into the MS, which results in a reduction of the total number of ions that can be measured. The effect of the sampler is especially important in the downstream region from the plasma, but not so important in the most intense plasma region (the coil region). The changes induced by the sampling cone are very sensitive to the distance from the sampler. The general behavior is a decrease in the central region and a rise in the outer areas for both the temperature and the electron density profiles, close to the sampler. Further away from the sampler, both temperature and electron density are higher than in the case without a sampler, also in the central region (Figure 2). Finally, as the distance of the sampler from the load coil increases, the plasma temperature at the place of the sampler decreases slightly, which also has consequences for the ion generation and transport through the sampling cone.
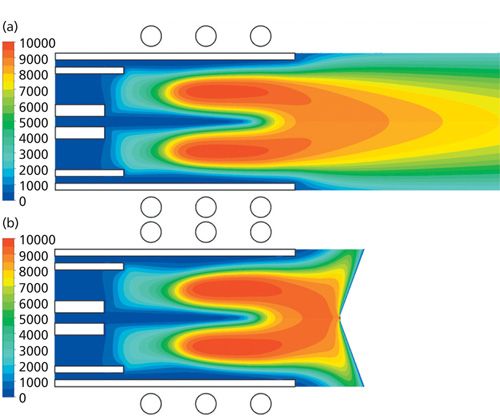
Figure 2: Two-dimensional (2D) temperature profile (K), (a) without and (b) with sampler. (Adapted with permission from the Royal Society of Chemistry, reference 1).
What insights do these results give us about ICP-MS methods?
These calculations yield information about the plasma very close to the sampler, a region that is not or barely accessible for measurements and yet is nevertheless the most important plasma region where the measured data are sampled from, as it is the connection point between the ICP and the mass spectrometer. Thus, an increase of the sampler temperature might yield an increased transmission of ions into the mass spectrometer.
You have also done computational studies to examine the effect of injector gas flow rate, auxiliary gas flow rate, and applied power on the plasma characteristics in an ICP connected to a mass spectrometer (3). What effects did you see from changes to the injector gas flow rate and the auxiliary gas flow rate?
Our calculations can predict the optimum range of operating and geometrical parameters for different setups. Indeed, to ensure that the injector gas entirely flows in a straight line and efficiently guides the sample toward the mass spectrometer, the injector gas flow rate should be at least 1.0 L/min for all the cases we studied (Figure 3). On the other hand, the injector gas flow rate should not exceed a maximum limit (for example, 1.4 L/min for the case studied in reference 3), to avoid a situation where the auxiliary gas cannot pass through regions in the plasma with high power coupling and cannot transfer the energy efficiently to the injector gas.
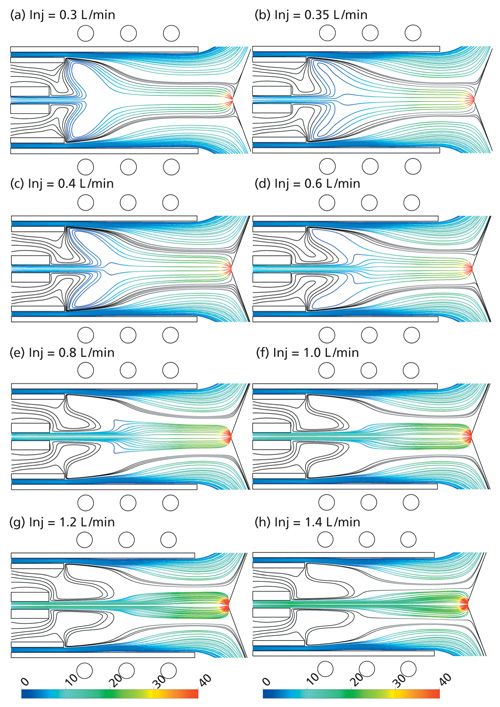
Figure 3: 2D gas flow velocity path lines originating from the central and outer inlets, colored by velocity in m/s, and from the intermediate inlet, colored in black, at different carrier gas flow rates, ranging from 0.3 to 1.4 L/min. The auxiliary gas and cooling gas flow rates are kept fixed at 0.4 and 1.2 L/min and the power is 1000 W. (Adapted with permission from reference 3.)
Furthermore, the injector gas flow rate should preferentially be higher than the auxiliary gas flow rate to prevent the auxiliary gas from passing through the sampler orifice and to ensure that only the injector gas can enter the mass spectrometer. This is necessary to optimize the detection efficiency of the sample substances, at least in the case when the ion cloud diameter is small-that is, when the analyte ions are transported only by the injector gas.
What is the impact of the level of applied power?
The applied power affects the gas path lines, and can therefore control which flow (whether only the carrier gas or also the auxiliary gas) can pass through the sampler orifice (Figure 4). Furthermore, the general effect of increasing the applied power is a rise in the plasma temperature, which results in a higher ionization in the coil region. However, it also reduces the length of the cool central channel, which is crucial for transferring the sample to the sampler (Figure 5). Moreover, at power values that are too high-for example ~1500 W, for the geometry described in reference 3-the auxiliary gas cannot pass through the high power coupling regions in the plasma anymore, and will not be able to transfer the energy efficiently to the carrier gas.
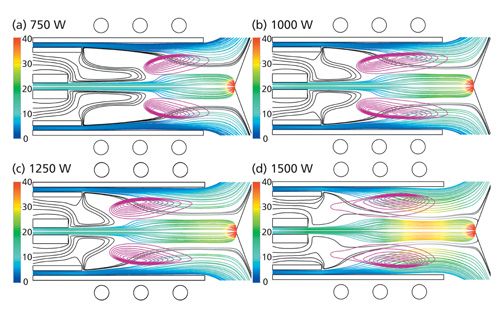
Figure 4: 2D gas flow velocity path lines originating from the central and outer inlets, colored by velocity in m/s, and from the intermediate inlet, colored in black, at (a) 750 W, (b) 1000 W, (c) 1250 W, and (d) 1500 W of applied power. The carrier, auxiliary, and cooling gas flow rates are kept fixed at 1.0, 0.4, and 1.2 L/min. (Adapted with permission from reference 3.)
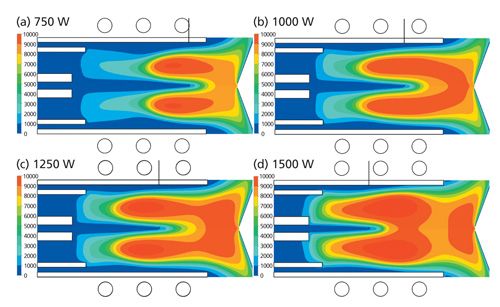
Figure 5: 2D temperature profile (K), at (a) 750, (b) 1000, (c) 1250, and (d) 1500 W of applied power. The carrier, auxiliary, and cooling gas flow rates are kept fixed at 1.0, 0.4, and 1.2 L/min. The small black lines above each plot indicate the length of the cool central channel. (Adapted with permission from reference 3.)
You also studied the effects of applied power, gas flow rates, and other parameters on the rotational motion of the gas flow in an ICP (4). What did that study show?
Using a 1.5-mm diameter injector inlet typically results in the occurrence of rotational motion only in the auxiliary-gas flow path lines and not in the carrier-gas flow path lines, when flow rates are higher than 0.9 L/min and at any applied power (see the example shown in Figure 4). However, when using a larger injector inlet diameter (2 mm), the carrier-gas flow will also exhibit some backward motion inside the torch (see Figure 6 for comparison), which is undesirable for sample transfer to the mass spectrometer. Our calculations predict that by increasing either the flow rates of the carrier gas (the most dominant factor), auxiliary gas, or cooling gas, or by raising the external power, we can control and avoid the backward motion. Moreover, using a sampler with a larger orifice diameter also results in a drop in the rotational behavior of the auxiliary gas flow. Note that, as mentioned before, there is a limit for increasing the carrier-gas flow rate, because above the limit, a lower amount of analyte will get ionized as a result of the high gas flow rate and the low temperature in the plasma.
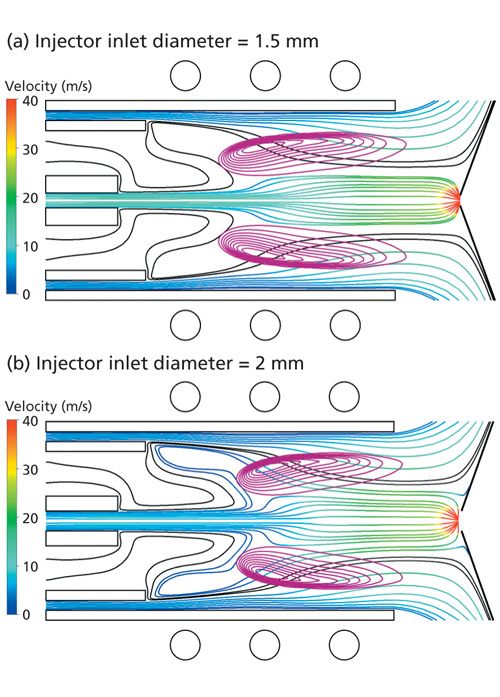
Figure 6: Effect of injector inlet diameter on gas flow velocity: (a) 1.5 mm and (b) 2.0 mm. 2D gas flow velocity path lines originating from the carrier gas and cooling gas inlets, colored by velocity (in m/s), and from the auxiliary gas inlet, colored in black. The sampler orifice diameter is 1.0 mm. The carrier gas, auxiliary gas, and cooling gas flow rates are 1.0, 0.4, and 12 L/min, respectively. The violet contours demonstrate the area of external power coupling. (Adapted with permission from the Royal Society of Chemistry, reference 4.)
Recently, you published an article about particle transport through the ICP, which includes injection of copper samples (5). Can you briefly explain how this new part of the model works?
The trajectory of each droplet is calculated by integrating the force balance acting on the droplets. Besides their transport throughout the torch, a heat and mass balance equation is applied, which relates particle temperature to the convective and diffusive heat transfer, and calculates the mass transfer to the vapor phase for the elemental droplets. The heat lost or gained by the particle as it traverses each computational cell will also appear as a source or sink of heat in the subsequent calculation of the continuous phase energy equation. We are currently also studying sample ionization. From the ionization degree and the (mass and charge) conservation equations, the number densities of electrons and of the atoms and ions of the sample material can be calculated. By integrating the number density of ions passing through the sampler over orifice width and comparing to the entering material, we can calculate the transport efficiency at different conditions.
What do the results show about particle transport?
Our calculations show that depending on the injection position, each droplet follows a different path line in the torch and sometimes it can expand in the radial direction from the central axis (see Figure 7). Our model provides us the axial and radial position at which the evaporation occurs, which depends on the droplet temperature along the torch. It also shows to what extent the vapor cloud moves in the radial direction and deviates from the central axis. In the case of on-axis injection, the droplets move in a straighter line along the torch and experience a later evaporation compared with off-axis injection. This reduces the probability of losing the sample inside the torch or the occurrence of a detection delay by preventing the droplets from early radial movement inside the torch. Therefore, the sample species can reach the sampler orifice with a higher transmission efficiency. Also, it ensures less expansion in the first vacuum stage and therefore it reduces the amount of sample that gets lost through the skimmer.
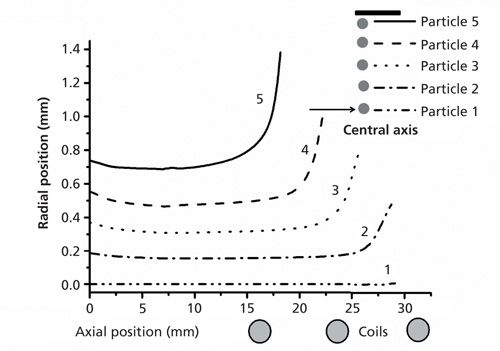
Figure 7: Effect of introduction position of the droplets on the radial position and of five different copper droplets (of 1 mm diameter), introduced with a flow rate of 100 ng/s from different initial radial positions at the central inlet. A schematic picture of the droplet positions at the central inlet as well as the load coils is also shown. (Adapted with permission from the Royal Society of Chemistry, reference 5.)
Based on the results of this work, what recommendations do you have for optimizing the operating conditions for good analytical practice in ICP-MS?
Our model allows us to track particles to determine their position, phase (liquid, vapor, or ionized), velocity, and temperature, both in the ICP torch and at the sampler orifice. More specifically, the shape and position of the ion clouds originating from the Ar flow and the inserted sample material are determined inside the torch, which can be used for emission spectrometry and mass spectrometry studies. From the calculation results, any deviation from the central axis, as well as any early or late evaporation along the torch are indicated, which are caused by non-optimal operating conditions. In fact, for any geometry, it is important to take into account the flow path lines exiting the torch, their expansion, and their interaction with the sampler cone, which are all affected by the geometrical and operating parameters. It is difficult to give general recommendations, because the behavior depends on the geometry and operating conditions. However, using our developed model, it is now possible to predict optimum conditions for specific cases, as indicated in our publications.
References
- M. Aghaei, H. Lindner, and A. Bogaerts, J. Anal. At. Spectrom. 27, 604–610 (2012).
- M. Aghaei, H. Lindner, and A. Bogaerts, J. Anal. At. Spectrom. 28, 1485 (2013).
- M. Aghaei, H. Lindner, and A. Bogaerts, Spectrochim. Acta, Part B76, 56–64 (2012).
- M. Aghaei, L. Flamigni, H. Lindner, D. Günther, and A. Bogaerts, J. Anal. At. Spectrom.29, 249 (2014).
- M. Aghaei and A. Bogaerts, J. Anal. At. Spectrom. DOI: 10.1039/c5ja00162e (2015).

Trending on Spectroscopy: The Top Content of 2024
December 30th 2024In 2024, we launched multiple content series, covered major conferences, presented two awards, and continued our monthly Analytically Speaking episodes. Below, you'll find a selection of the most popular content from Spectroscopy over the past year.
Best of the Week: Hyperspectral Imaging, ICP-MS Analysis of Geological Samples, Product Roundup
October 18th 2024Top articles published this week include an article about hyperspectral imaging in human skin research, a peer-reviewed article about analyzing geological samples using atomic spectroscopy techniques, and an equipment roundup piece about the latest products in the industry.