Fiber Optics
Getting light from one place to another is a key task in any spectroscopic method. Sometimes we send light through (mostly) empty space using mirrors or lenses, and sometimes we use things called fiber optics. This installment of "The Baseline" tackles fiber optics.

Fiber optics is the branch of optics that deals with optical fibers. Optical fibers are thin fibers of glass or plastic that are used to transmit light signals. The fibers are usually cylindrical, but for certain special purposes, they can have elliptical or rectangular cross sections. The field of fiber optics, and the optics of the fibers involved, is a fascinating application of various spectroscopic topics. Indeed, fiber optics has made its way into spectroscopy, acting as light guides in monochromators, remote detectors, light sources, and other components.
Total Internal Reflection
Optical fibers depend upon total internal reflection for transmission of light. Total internal reflection is a consequence of Snell's law for the refraction of light going from one medium to another (1). See Figure 1:
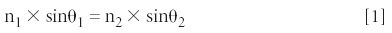
Here, n1 and n2 are the indices of refraction of the light-transparent medium, and θ1 and θ2 are the angles the light rays make with the normal to the interface surface. As illustrated in Figure 1, when light is going from a medium of higher refractive index to a medium of lower refractive index, the light's angle with respect to the normal increases.

Figure 1: Geometric description of Snell's law.
As the angle with the normal in the higher refractive index medium increases, so does the refracted angle in the lower refractive index medium. As Figure 2 shows, there comes some angle at which the refracted light becomes refracted parallel to the interface. This is the critical angle for the interface. The critical angle θc is given by the expression

At any incident angle larger than the critical angle, the light does not pass through the interface, but is instead reflected back into the original medium. This is known as total internal reflection. Essentially, optical fibers work because light is totally internally reflected down the length of the fiber.
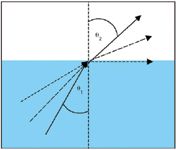
Figure 2: Snell's law requires that, at some angle, light will not enter the region of lower refractive index. At this point, the light experiences total internal reflection.
Optical Fibers
The simplest optical fiber is composed of a glass or plastic transparent core surrounded by a layer called the cladding, which must have a lower refractive index. Fibers may also be surrounded by an additional protective coating. Typically, the differences in the index of refraction of the core and cladding are small so that the critical angle is close to 90°, as measured from the normal, so that light coming in closely parallel to the interface experiences total internal reflection. As light rays get sequentially internally reflected, they ultimately propagate down the length of the fiber, emerging at the other end, as shown in Figure 3. The conical region from which light rays originate and are internally reflected internally is called the acceptance cone. Depending upon the application, acceptance cones can be designed to be large (when connecting the end of a fiber to a detector) or small (when using monochromatic light or short pulses). Large acceptance cones also allow light to travel either very near the cylindrical axis or at relatively large angles to the axis, allowing for highly variable pathlengths of the light rays. Hence, two light rays entering a fiber at the same time can take much very different times to traverse a given length of fiber, contributing to an effect called dispersion. While not an issue for continuous light, for light pulses, a large dispersion can lead to lengthening of the pulse and a loss of bandwidth.
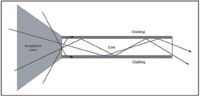
Figure 3: Ray diagram of how an optical fiber propagates light rays.
Optical fibers can be only a few wavelengths in diameter; in such cases, not only do they have a relatively narrow acceptance cone, but their behavior must be considered in terms of electromagnetic theory rather than ray-tracing. Narrow-diameter fibers – typically 8–10 μm in diameter – can be thought of as waveguides for light, much like waveguides for radio waves in magnetic resonance spectroscopy. Narrow fibers are called single-mode fibers because they will only transmit one kind of transverse mode of light wave. The exact mode does depend upon the wavelength of light used, so even single-mode fibers effectively can transmit more than one mode of light effectively.
The key to making optical fibers is to get very pure glass or plastic. Impurities are the bane of optical fibers: more light is attenuated because of absorption by impurities, rather than scattering effects. Very high purity silicon dioxide or germanium dioxide, carefully doped with additives, is made into a cylinder and surrounded by a layer of the appropriate cladding material. This construction is called the preform. This preform is heated to 1900–2200 °C to melt the end, which is drawn at a rate of 10–20 m/s to form a fiber of given diameter. The exact temperature of the preform and the speed of pulling determine the ultimate diameter of the optical fiber.
Uses
Optical fibers are used anywhere someone wants to transmit light from one spot to another, especially along paths that are not perfectly straight – one advantage that optical fibers have is flexibility. In spectroscopy, source light can be delivered to and from samples much farther than the source itself, and with samples that might not fit inside a sample chamber, for example, inside production lines to check for product purity. Fiber optics is very useful in medical imaging, where in which they are adapted into endoscopes and laparoscopes for viewing inside the body. And any electrical signal that can be converted to a light signal can be transmitted easily and more efficiently using optical fibers instead of copper or aluminum wiring.
David W. Ball is a professor of chemistry at Cleveland State University in Ohio. Many of his "Baseline" columns have been reprinted in book form by SPIE Press as The Basics of Spectroscopy, available through the SPIE Web Bookstore at www.spie.org. His new textbook, Physical Chemistry, was published in August 2002. He can be reached at d.ball@csuohio.edu his webiste is academic.csuohio.edu/ball.

High-Speed Laser MS for Precise, Prep-Free Environmental Particle Tracking
April 21st 2025Scientists at Oak Ridge National Laboratory have demonstrated that a fast, laser-based mass spectrometry method—LA-ICP-TOF-MS—can accurately detect and identify airborne environmental particles, including toxic metal particles like ruthenium, without the need for complex sample preparation. The work offers a breakthrough in rapid, high-resolution analysis of environmental pollutants.
The Fundamental Role of Advanced Hyphenated Techniques in Lithium-Ion Battery Research
December 4th 2024Spectroscopy spoke with Uwe Karst, a full professor at the University of Münster in the Institute of Inorganic and Analytical Chemistry, to discuss his research on hyphenated analytical techniques in battery research.
Mass Spectrometry for Forensic Analysis: An Interview with Glen Jackson
November 27th 2024As part of “The Future of Forensic Analysis” content series, Spectroscopy sat down with Glen P. Jackson of West Virginia University to talk about the historical development of mass spectrometry in forensic analysis.
Detecting Cancer Biomarkers in Canines: An Interview with Landulfo Silveira Jr.
November 5th 2024Spectroscopy sat down with Landulfo Silveira Jr. of Universidade Anhembi Morumbi-UAM and Center for Innovation, Technology and Education-CITÉ (São Paulo, Brazil) to talk about his team’s latest research using Raman spectroscopy to detect biomarkers of cancer in canine sera.