Those Ions Know What They Are Doing, Part I

The phrase "the ions know what they are doing" emphasizes that ions, once formed, react according to known rules and logical patterns. We can change their environment in different experiments and observe their behavior with different instruments, but they follow the rules. We depend on this underlying rationality so that, for example, a pattern of unimolecular dissociation reactions in electron ionization can be interpreted to discern ion structure, and by analogy, molecular structure. An integral part of all mass spectral interpretation, using the variety of ionization methods available, is to examine the mass spectra, deduce the rules, and discover those patterns. Accomplishing this requires an understanding of the environment in which the ions are formed, the environments in which they dissociate, and the time they reside within each. Because ions move through a beam instrument or reside for extended periods in others, the range of environments becomes broad, and the experimental options numerous.

Welcome to analysis by modern mass spectrometry (MS). Would you like ions from your sample created by electron ionization (EI), chemical ionization (CI), electrospray ionization (ESI), or matrix-assisted laser desorption–ionization (MALDI)? Perhaps you may be interested in another variant in ionization methods accessible to the modern analyst. If you have been following this column over the years, you realize that some of these ionization methods may create protonated ions from the molecule, while others may create odd-electron molecular ions. Some of the ionization methods are considered "hard" (creating ions with a great deal of internal energy), and others are "soft" (creating ions that contain less internal energy). Intuitively, we surmise that ions with more internal energy are likely to dissociate to a greater degree, at faster rates, or follow different dissociation pathways than a cool ion does. We are not surprised that the recorded mass spectra change when using different ionization methods or recording the mass spectra under different experimental conditions. Invariably, if we observe a difference, we then seek to explain it. Ions created by EI and CI have been examined for decades, and our understanding is advanced, underpinning the discussion in this column. For ESI and MALDI, despite the broad applicability of the methods, our understanding of the ionic environments in those sources has not yet advanced to an analogous level of detail.

The Fundamentals

An elementary fact about chemical reactions is that their rate depends on the temperature of the system. For many reactions, the reaction rate increases with an increase in temperature, and that dependence is shown by the classical Arrhenius rate equation. In short, the Arrhenius rate equation expresses the rate constant k of a reaction on the temperature T:

k = Ae^-E(act)/RT                                                                                     [1]

In this equation, A is the pre-exponential factor, R is the universal gas constant, and E(act) is the activation energy for the reaction. The activation energy reflects the fact that it takes a certain amount of energy to align the participants in the reaction so that the reaction can proceed. If there was no activation energy for reactions, all reactions would proceed spontaneously and irreversibly to the lowest-energy end result.

Electron Ionization

Can a simple relationship such as the Arrhenius rate equation be helpful in understanding the dissociation reactions that occur in MS? Let's consider EI first. If we know the structure of the molecule from which an ion is formed, by the Franck–Condon principle we know the structure of the ion that is formed in EI. Subject to any perturbations induced by the ionic charge, we know (from other spectroscopic methods) the bond strengths between each atom in the ion and its neighbor. So, if we know the "temperature" of the ion, we might be able to deduce something about the dissociation rate. But there is no one rate for the dissociation of this ion. The nascent molecular ion M⁺ will fragment in competing dissociation reactions, each with a different rate. The recorded EI mass spectrum represents the collection of ions resulting from these competing reactions. In the absence of a series of reactions (which complicates matters further because the individual reactions in the sequence can proceed at different rates), faster reactions will produce fragment ions of higher relative intensity in the measured mass spectrum.

So, we realize immediately that the concept of temperature requires refinement in the context of MS, the ionic environment, and the rates of dissociation reactions of ions. Temperature is a collective property of a system in which some sort of equilibrium has been achieved. That equilibrium is established by a dynamic, but predictable, exchange of energy between all of the involved partners. The concept of temperature is easily understood within the concept of an ideal gas. In the ideal gas, collisions between atoms or molecules are perfectly elastic, and there are no intermolecular attractive forces. The common teaching analogy is to describe the ideal gas behavior as billiard ball collisions. This analogy has its limitations, but it focuses attention on the fact that the energy of such a system is primarily in the form of the kinetic energy of the moving particles. Although some particles may be moving faster and some more slowly, the kinetic energy of the system as a whole is quantified through a temperature T, and the temperature is related to pressure and volume through the ideal gas law: PV = nRT. The gas that exists in the ionization source of a mass spectrometer is a collection of ions and neutral molecules; only a small fraction of the sample molecules are ionized, so in many respects we might treat the system as if it were composed completely of the un-ionized gas molecules from sample, from residual air molecules, and from any gases that we purposefully add to the source.

Chemical Ionization

The pressure in an EI source is low (about 10^-6 Torr). In a CI source, the pressure is maintained at about 1 Torr (760 Torr is approximately atmospheric pressure) with an added reagent gas — perhaps this might be a better candidate for looking at the concept of temperature. The temperature of the metal walls of the CI source is around 150–200 °C; the effective temperature of the enclosed reagent gas is probably close to that value. The CI source is often termed a "closed source," so we might even expect that an approximate thermal equilibrium can be attained within its confines. But the ions created in a CI source are not in an energy equilibrium with the neutral gas molecules, and the temperature of the gas does not control the reaction rates of the ions. In the CI source, a reagent gas (methane, isobutane, or ammonia, among many other choices) is ionized to create a reagent ion that transfers a proton to the molecule M to form (M+H)⁺. If methane is used as the CI gas, the overall reaction is

M (the molecule) + CH₅⁺ → (M+H)⁺ (the protonated molecule) + CH₄            [2]

So, what is the internal energy of the (M+H)⁺ ion that we can relate to how much dissociation occurs? The amount of internal energy in (M+H)⁺ depends on the ΔH of the proton transfer reaction shown above. The sample molecules are more basic than CH₅⁺, and the proton is transferred, along with energy. Because the overall energy of the reaction must be balanced, the amount of internal energy of the (M+H)⁺ ion is determined by the difference in proton affinities of the molecule and the reagent gas molecule. Ionization with the reagent ion formed from methane leaves more internal energy in the protonated molecule than does isobutane, and both leave more energy than ammonia. More internal energy results in more dissociation. The fragment ions that we observe in the CI mass spectrum represent the end collection of all of the in-source dissociations. In a sense, the mass spectra, with either more or less dissociation, correspond to a hotter or colder "temperature" of the ions, respectively. But this casual use of temperature-related terms to describe the extent of dissociation reactions is misleading.

Assessing the Internal Energy

The CI source, however, does produce a level of predictability in ion dissociations based on the ΔH of the proton transfer reaction. What can we conclude about the internal energies of those protonated molecules (M+H)⁺ that do not dissociate in the source, but survive to be drawn into the mass analyzer along with the fragment ions? Now, assessment of the internal energy of these ions becomes more complicated because there are several possibilities. Perhaps these ions have all of the internal energy given to them in the proton transfer reactions, but simply have not reacted during their residence time in the source. Perhaps these ions had all of that internal energy initially, but were de-excited in collisions within the source (that is, collisions that were nonelastic). Perhaps the ions have other means of releasing energy into the environment, such as radiative emission. The uncertainties in the evaluation of the ion's internal energy become greater, even as the ionic environment becomes simply the isolated ion within a vacuum without any collisions.

In most instruments, after ions leave the ion source they are in a lower-pressure, collision-free environment. Whatever dissociation reactions these ions now undergo are unimolecular reactions — there are no collisions with other molecules or ions, and there is no system "temperature." The rates of the dissociation reactions depend only on the properties of the ion itself. How do we assess the effect of internal energy on the rates of unimolecular reactions? We use statistical rate theories, which are introduced here and will be explored more fully in the next column. A theory widely used in MS is called the quasi-equilibrium theory (QET). Here is a description of its underlying assumptions and most cogent conclusions.

Quasi-Equilibrium Theory

Vekey (1) summarizes the fundamental assumptions of statistical rate theories as follows:

• Ion dissociation reactions occur much more slowly than the initial ionization process;

• ion dissociation reactions occur more slowly than any redistribution of energy among internal degrees of freedom of the ion;

• ions are in a state of internal equilibrium, but do not exchange energy with the environment; and

• ionic products are formed by a series of competing, and sometimes consecutive, dissociation reactions.

These four separable assumptions can be distilled into one maxim — the ions, once formed, redistribute internal energy (and even structure) totally within themselves until they are in their most stable state, and then (and only then) will dissociation reactions occur. The implications of the QET are thoroughly discussed by Sztaray (2).

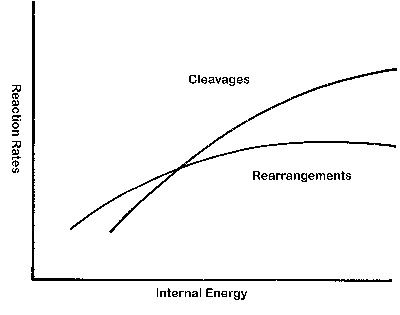
We will describe QET more fully in Part II, but we'll introduce a few of its more notable predictions now. Figure 1 shows (in general) the predicted dependence of reaction rate on ion internal energy for two distinct types of fragmentation reactions. In direct cleavages, a bond between two adjacent atoms is stretched (while the rest of the ionic structure remains about the same) until the bond ruptures. In a rearrangement, multiple atoms must move (and bond lengths change, as bonds are broken and new bonds form) in a concerted fashion to create the required ionic structure for the dissociation to proceed. For both types of reactions, the rate generally increases with energy. But, generally, a rearrangement reaction (if structurally possible) occurs faster at a lower internal energy than a direct cleavage. A rearrangement may seem less likely than a direct cleavage, but the fact that new bonds are formed in the rearranged ion provides a "return" that favors the rearrangement. In the interpretation of mass spectra, the products of rearrangement reactions have great significance. Many ions have at least the low internal energy for a fragmentation to occur, and the structural requirements are diagnostic.

Figure 1: The quasi-equilibrium theory (QET) predicts a distinctive behavior in the reaction rates of cleavages vs. rearrangements in ions as a function of their internal energy.

The test of the QET is whether its predictions match what can actually be observed in experimental mass spectra. Changes in fragment ion relative intensities as a function of ion internal energy comprise what is called a "breakdown curve." There may be substantial uncertainties in the values of both the x (internal energy) and y (ion intensity) values. A generic example of a breakdown curve is shown in Figure 2. Certain molecules have been thoroughly studied both theoretically and experimentally; these compounds are called thermometer molecules (3). Thermometer molecules are well known in MS, both in the study of the classical ionization methods and in deducing the basics in MS-MS excitation. New types of thermometer ions have been proposed to discern energetics in MALDI. The study of their dissociation reactions as a function of experimental conditions provides insight into the energetics of the ions created. Again, the terminology is misleading; the thermometer molecules do not tell us about "temperature." They do, however, provide a series of calibration points that are invariant, and provide an anchor for assessing the multitude of ionization and excitation processes in modern MS.

Figure 2: A breakdown curve plots the relative intensity of various fragment ions formed by unimolecular dissociation reactions as a function of the internal energy of the ion.

Kenneth L. Busch has read with interest the recent reanalyses of the results of the GC–MS experiments from the Viking lander on Mars. Mars has not changed in the past 40 years since those experiments were designed and the results first were received and analyzed. The samples are as they have always been; they have probably been stable for millennia. But our instruments evolve, and our understanding of their operation and the interpretation of the data they produce becomes more sophisticated. To recall A.C. Clarke, what we accomplish now must seem like magic. In 50 more years, we will be ignorant. Through it all, the ions know what they are doing. These contents are the sole responsibility of the author, who can be reached at wyvernassoc@yahoo.com, and can't balance his checkbook.

References

(1) K. Vekey, J. Mass Spectrometry 31(5), 445–463 (1996).

(2) J. Sztaray, PhD dissertation, Eotvos Lorand University, Institute of Chemistry, Budapest, Hungary, 2009.

(3) G. Luo, I. Marginean, and A. Vertes, Anal. Chem. 74, 6185–6190 (2002).