Atomic Clocks: An Application of Spectroscopy
In the previous installment of this column, the author discussed clocks as the first scientific instrument. What do clocks have to do with spectroscopy? Actually, the world's most accurate clocks, atomic clocks, are based upon a spectroscopic transition of cesium or other elements, making spectroscopy a fundamental tool in our measurements of the natural universe.

Time is one of the seven fundamental quantities in nature. I made a case in the last installment of this column (1) that mechanical devices for measuring time — clocks — might be considered the world's first scientific instruments. Clocks are ubiquitous because the measurement of time is a fundamental activity that is important to computer users, pilots, and lollygaggers alike.
The Second
Quantities of time are expressed in a variety of units that we teach our grade-schoolers, but the IUPAC-approved fundamental unit of time is the second (abbreviated "s"). Units such as minute, hour, day, and year, as well as prefix-modified units such as millisecond, nanosecond, and megasecond, are all based directly upon the second; derived units such as the newton (where 1 N = 1 kg·m2 /s) and joule (1 J = 1 N/s = 1 kg·m2 /s2 ) also are based upon the second. (Interestingly, within the SI system of units, the units for time greater than second — minute, hour, day, and so forth — are the only units not based upon powers of 10 times the basic unit. What was once referred to as the "metric system" is now properly referred to as the "SI system" of units, with "SI" coming from the French phrase "système international d'unités," or "international system of units.") Ultimately, it becomes fundamentally important to be able to determine exactly how long a second is.

David W. Ball
Originally, a second was defined as part of a minute, which was part of an hour, which was in turn defined as part of a day. Thus, 1 s was 1/(60 × 60 × 24), or 1/86,400 of a day. However, even by the 17th century, defining the day itself was difficult. Was the day based upon the position of the sun (the solar day) or the position of distant stars (the sidereal day)? At what latitude (that is, position toward the north or south) is a day measured? Over time it was recognized that measuring time accurately was a challenge.
In 1660, the Royal Society proposed that a second be determined by the half-period (that is, one swing) of a pendulum of a given length. That given length was very close to what became 1 m, suggesting a relationship between the standard unit of length and the standard unit of time. However, it was recognized that the period of a pendulum of given length varied with position on the Earth's surface. Nonetheless, the concept of a "seconds pendulum" was useful through the 18th century in France, England, and the Americas, with the length of the pendulum varying between 99.0 and 99.4 cm, depending upon location and often specified to five decimal places.
In 1956, it was finally recognized that the rotation of the Earth was too inconstant to use in the definition of a second. Instead, the revolution of the Earth about the Sun was used. In 1960, the Eleventh General Conference on Weights and Measures (Conférence générale des poids et mesures, CGPM) ratified a new definition of the unit second:
1/31,556,925.9747 of the tropical year for 1900 January 0 at 12 hours ephemeris time
"Ephemeris time" is an older time scale based upon the positions of celestial bodies. Because the length of the "tropical year" (a year as measured going from one point to the same point on the celestial ecliptic) varies over long periods of time as well as on the starting and ending points of the ecliptic, a "mean tropical year" is the average of all points over one solar year, and the imaginary tropical year starting at 1900 January 0, 1200 hours formed the basis for the second.
Spectroscopic Definition of Second
Even this definition, however, was problematic because of variations in the Earth's motion about the Sun. Enter spectroscopy.
In the late 1940s and through the 1950s, work on atomic clocks progressed. Early atomic clocks were developed at the U.S. National Bureau of Standards (now the National Institute of Standards and Technology, or NIST) and the National Physical Laboratory (NPL) in the UK. Atomic clocks essentially are masers (microwave amplification by stimulated emission of radiation) that have extremely stable outputs. They have been based upon hydrogen, rubidium, or cesium, although the cesium atomic clocks are the ones upon which the modern definition of the second is based.
In 1967, based upon the work of astronomers from NPL and the United States Naval Observatory (USNO), the second was redefined as:
The duration of 9,192,631,770 periods of the radiation corresponding to the transition between the two hyperfine levels of the ground state of the caesium-133 atom.
In 1997, this was amended with the statement:
This definition refers to a caesium atom at rest at a temperature of 0 K.
This amendment was required to compensate for thermal variations in the frequency of the transition. At this point, the way to identify a second's worth of time is to count 9,192,631,770 wavelengths of light coming from the hyperfine levels of cesium-133. This, by the way, corresponds to a wavelength of 3.2612256 cm, which lies in the microwave region of the electromagnetic spectrum, nearer to the infrared region than the radiowave region. The ability to measure a second, then, is based upon our ability to measure this particular spectroscopic transition of 133 Cs.

Figure 1
Why cesium (or, as preferred by IUPAC, caesium)? Several reasons:
- Cesium has a relatively low boiling point of 941.4 K (668.3 °C), so obtaining cesium in the vapor phase is relatively easy. In fact, an operating temperature of only 80 °C is required to generate enough Cs vapor for some atomic clocks to operate.
- Cesium is a fairly heavy atom (133 amu), meaning that its thermal velocity at any temperature is low, increasing measurement times and decreasing Doppler effects on its spectrum.
- Cesium has a single valence electron, and in some respects, the electronic spectrum of cesium can be approximated loosely as a one-electron system, like atomic hydrogen.
- Natural cesium exists as one isotope, having mass number 133. Hence, any sample of naturally occurring cesium is isotopically pure and its spectrum is uncontaminated by any isotope effect.
- Cesium has a relatively large nuclear spin I of 7/2. Nuclei of other elements have this or even higher spin (for example, 138 La has a spin of 5), but they suffer by not having the other advantages of cesium.
- Cesium has a fair-sized nuclear magnetic moment. These last two properties lead to:
- Cesium has an unusually large hyperfine splitting in its ground state, caused by the magnetic interaction of the spin from the unpaired 6s1 valence electron and the I = 7/2 nucleus.
It is this hyperfine structure that causes the energy level diagram that is involved in the definition of the second. Figure 1 shows a diagram of the electronic energy levels of the Cs atom. The interaction of the magnetic dipole moment of 133 Cs (due to its nonzero spin) and the magnetic dipole moment of the orbiting 6s1 valence electron causes the formation of new energy levels based upon the interaction of the magnetic moment vectors; the energy change is calculated by with a called the hyperfine structure constant.
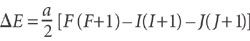
It is the transition from the ground state, labeled 6 2 S1/2 (F = 3), to the first hyperfine excited state, 6 2 S1/2 (F = 4), that is involved in the definition of the second. It is worth noting that, with such a relatively low energy, even at 80 °C there are a significant number of atoms in the F = 4 state due to thermal population.
The Atomic Clock
How does an atomic clock work? Older atomic clocks were based upon beams of cesium atoms, with the atoms moving at several hundred meters per second. The most modern atomic clock at NIST uses what is called an atomic fountain. Figure 2 shows a general schematic of the clock (3,4). Initially, a cloud of cesium vapor is introduced into a vacuum chamber. Six perpendicularly placed infrared lasers are used to capture a small cloud of cesium atoms at their intersection; the repeated rebounds of photons from all directions slow the gas-phase cesium atoms to near absolute zero (the phrase "optical molasses" is sometimes used to describe the conditions experienced by the cesium atoms). Approximately 100 million atoms are captured. At this point, atoms populate the F = 3 and F = 4 states, which also have quantized z-components of angular momentum ranging from mF = –3 to 3 for the F = 3 state, and from mF = –4 to 4 for the F = 4 state. Only the mF = 0 states are useful, so atoms not in this state must be eliminated.
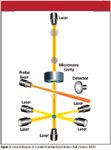
Figure 2
The atoms are then pushed up by the two vertical lasers, and then all of the lasers are turned off. The atoms drift up about 1 m under the influence of gravity, passing through a microwave cavity. A short burst from the microwave cavity drives the atoms having F = 4 and mF = 0 (using the Dirac braket notation, labeled the |4,0› state) into the |3,0› state by way of stimulated emission. Then, a burst of radiation from an 852-nm laser causes absorption from the F = 4 state to the F' = 5 state, essentially heating up the atoms and removing them thermally. The atoms of cesium are now essentially all in the |3,0› state.
The cloud of atoms then passes through a microwave cavity tuned (hopefully) to 9192631770 Hz. They absorb the microwave radiation to transfer to the |4,0› state. They are then hit again by the 852-nm laser, transfer to the F' = 5 excited state, and fluoresce back to the F = 4 state. The fluorescence is detected optically.
The process is repeated multiple times until the fluorescence output is maximized. It is at this point that the microwave cavity is tuned to the "correct" frequency.
Because the fountaining motion of the cesium atoms is slow (about 4 m/s) and the atoms themselves are cooled by the optical molasses to only a few microkelvins, the atoms interact with the microwave field for about a second. This allows for a very narrow linewidth for the measured transition, allowing for a Δv/v of less than 10-15 — a very accurate measurement. This corresponds to an accuracy of 1 s in about 31.7 million years. Modern atomic clocks are so accurate that the greatest errors are thought to arise from collisions between cesium atoms, uncertainties in the local gravitational field, and effects due to ambient blackbody radiation (that is, the fact that the clock itself is operating mostly at room temperature). New atomic clocks are being designed to minimize these effects, which might ultimately include operation of the clock in a micro- or nongravity environment (5,6).
Conclusion
The defining of the units we use to characterize the physical universe has to be among the most fundamental work a scientist can do. Spectroscopists should take some pride in knowing that the tools of their trade are instrumental in determining the value of the unit used to measure time. Interested readers are urged to consult the references and the works cited within for additional information.
Acknowledgment
Thanks to Dr. Steve Jefferts of the NIST Time and Frequency Division in Boulder, Colorado, for technical assistance.
David W. Ball is a professor of chemistry at Cleveland State University in Ohio. Many of his "Baseline" columns have been reprinted in book form by SPIE Press as The Basics of Spectroscopy, available through the SPIE Web Bookstore at www.spie.org. His most recent book, Field Guide to Spectroscopy (published in May 2006), is available from SPIE Press. He can be reached at d.ball@csuohio.edu his website is academic.csuohio.edu/ball
References
(1) D.W. Ball, Spectroscopy21(12), 87–95 (2006).
(2) J.F. Milligan, Am. J. Phys.42, 883 (1974).
(3) Available online at http://tf.nist.gov/timefreq/cesium/fountain.htm. Accessed November 1, 2006.
(4) S.R. Jefferts, J. Shirley, T.E. Parker, T.P. Heavner, D.M. Meekhof, C. Nelson, F. Levi, G. Costanzo, A. De Marchi, R. Drullinger, L. Hollberg, W.D. Lee, and F.L. Walls, Metrologia39, 321 (2002).
(5) S.A. Diddams, J.C. Bergquist, S.R. Jefferts, and C.W. Oates, Science306, 1318 (2004).
(6) S.R. Jefferts, T.P. Heavner, and E.A. Donley, Jpn. J. Appl. Phys.43, 2803 04).
LIBS Illuminates the Hidden Health Risks of Indoor Welding and Soldering
April 23rd 2025A new dual-spectroscopy approach reveals real-time pollution threats in indoor workspaces. Chinese researchers have pioneered the use of laser-induced breakdown spectroscopy (LIBS) and aerosol mass spectrometry to uncover and monitor harmful heavy metal and dust emissions from soldering and welding in real-time. These complementary tools offer a fast, accurate means to evaluate air quality threats in industrial and indoor environments—where people spend most of their time.
NIR Spectroscopy Explored as Sustainable Approach to Detecting Bovine Mastitis
April 23rd 2025A new study published in Applied Food Research demonstrates that near-infrared spectroscopy (NIRS) can effectively detect subclinical bovine mastitis in milk, offering a fast, non-invasive method to guide targeted antibiotic treatment and support sustainable dairy practices.
