Laser-Induced Breakdown Spectroscopy for Analysis of Aerosol Particles: The Path Toward Quantitative Analysis

The application of laser-induced breakdown spectroscopy (LIBS) for the analysis of aerosol systems is a challenging problem that entails a wide range of physical phenomena that are coupled to the ultimate analyte response. While the analysis of aerosol particles dates back to some of the earliest LIBS studies, the evolution of understanding of the many processes involved in transforming a solid particulate into a collection of dissociated atoms and ions necessary for atomic emission spectroscopy has largely occurred over the last decade. During this time, a number of studies have attempted to elucidate the physics of particle vaporization, dissociation and ionization, diffusion of heat and mass, and ultimately, the resulting atomic emission. This review seeks to summarize the evolution of thought with regard to LIBS-based analysis of aerosol systems and provide insight into future research directions.

The technique of laser-induced breakdown spectroscopy (LIBS) makes use of a laser-induced plasma to vaporize and dissociate a targeted material. Subsequent atomic emission from the analyte species within the laser-induced plasma forms the basis of LIBS as an analytical technique. One might trace the beginning of quantitative LIBS-based aerosol analysis to the pioneering work of Radziemski, Cremers, and colleagues (1). In their study, beryllium-rich aerosols were used for generation of calibration curves and subsequent calculation of detection limits. They demonstrated the viability of LIBS for detection and quantitative analysis of aerosol samples. In a following study, in which particle sizes were extended to the submicrometer-sized range, Radziemski and colleagues (2) generated calibration curves for three analytes — namely cadmium, lead, and zinc — which were characterized by initial linearity followed by various degrees of saturation at higher concentrations. The saturation effects were attributed to incomplete vaporization of the analyte-containing particles. Over the following two decades, the LIBS community often cited an upper size limit for complete particle vaporization of about 10 μm, some directly referring to the extensive work of Radziemski and Cremers (3,4), while others referred to such a limit as a more general guideline. Radziemski and Cremers (2) did report that the LIBS technique is useful as long as the aerosol particles are below about 10 μm in diameter and somewhat monodisperse, although no systematic study was ever undertaken to specifically quantify this issue. Only in more contemporary LIBS studies was the issue of an upper size limit for quantitative analysis of aerosol particles addressed directly (5,6), as discussed in more detail later.

An issue closely related to complete particle vaporization is the issue of the independence of the analyte response on the analyte source. In the larger analytical community, such a topic would be considered to fall under the label of matrix effects, although such terminology was not largely used in the LIBS community with regard to aerosol analysis. Semantics notwithstanding, the issue was addressed from the beginning, including in the early work by Radziemski and colleagues (2). An important finding of their study was the general agreement (within 10%) of lead atomic emission signals of comparable atomic lead concentrations when nebulizing either lead acetate, lead chloride, or lead nitrate. In addition to analysis of particle-derived analyte signals, researchers also have looked at the gas phase of the aerosol system, where the relative independence of analyte signals on the molecular source was reported in several studies (7,8). Specifically, Dudragne and colleagues (7) demonstrated that analyte signals for fluorine, chlorine, sulfur, and carbon scaled with the number of respective analyte atoms in the constituent molecules for a wide range of compounds, concluding that the parent molecules were fully dissociated in the laser-induced plasma. Tran and colleagues (8) verified that SF₆ and HF yielded identical fluorine atomic emission signals when the gas composition was adjusted to contain the same atomic fluorine concentration.

This discussion is by no means a comprehensive review of the early evolution of LIBS for aerosol analysis, but rather, is intended to set the stage for a more in-depth discussion of the physics of LIBS-based analysis of aerosol particles. However, the general concepts outlined here, namely, complete particle vaporization and independence of the LIBS signal on analyte source (that is, lack of significant matrix effects) — do provide the basis and motivation for a wide range of LIBS-based aerosol studies. In a number of applied studies, LIBS-based sensing has been implemented successfully for continuous on-line monitoring of emissions and industrial processes (9–24), for analysis of ambient air particulate matter (25–28), and for general aerosol systems and nanoparticles (29–33). LIBS also was used in conjunction with single-shot analysis to effectively sample and analyze aerosol populations using discrete particle analysis (12,25,34,35). Other studies have addressed the feasibility of LIBS for analysis of biological materials and bioaerosols (36–44). In view of the wide array of potential applications, important research issues regarding LIBS-based analysis of aerosol systems can be identified for further analysis, as discussed in the remainder of this review.

Plasma–Particle Interactions: The Limiting Cases of Finite and Infinite Rates of Relevant Transport Properties

The phrase plasma–particle interactions implies an interaction between the analyte particle and the laser-induced plasma rather than between the particle and the laser beam. This is an important distinction that arises from consideration of the laser focal volume and the resulting laser-induced plasma volume. The latter is typically several orders of magnitude greater, which directly translates to a dominance of the probability of plasma–particle interactions as compared with direct laser-particle sampling. This is verified in the context of plasma volume measurements, particle sampling rate considerations, and direct imaging studies (45–47). The plasma–particle processes also must be framed in the context of the overall plasma lifetime (~100 μs for ~100 mJ/pulse air breakdown) and the analytical time-scales (that is, detector delays and gates), which typically range from ~5 μs to 50 μs following breakdown (48).

With this framework in mind, the introductory comments speak directly to widely proposed assumptions within the LIBS community, namely, that complete dissociation of constituent species within the highly energetic laser-induced plasma results in analyte signal linearity with increased analyte concentration, and on the independence of the analyte atomic emission signal from the analyte source. From a physics point of view, these concepts can be extrapolated to the context of process rates. If the time scale for complete analyte vaporization and dissociation is much less (that is, an order of magnitude faster) than the analytical time scale, it becomes essentially instantaneous, and the atomic concentration might be expected to scale linearly with analyte mass. If the time scale for diffusion of heat through the plasma and the time scale for diffusion of analyte mass through the plasma are also much less than the analytical time scale, the analyte spatial distribution, excitation, and ensuing atomic emission should correspond to the overall plasma conditions (bulk or spatially averaged), with no localized perturbations due to the presence of a vaporizing analyte particle.

This scenario corresponds to the ideal situation, in which aerosol particle-derived analyte species interact uniformly with an unperturbed analytical laser-induced plasma. In contrast, the opposite scenario might be envisioned, in which the time scale of particle vaporization and dissociation, and the time scales for heat and mass transfer, are all very slow compared to typical analytical time scales of plasma evolution (~5–50 μs). Such a scenario will result in a nonlinear analyte response, as a significant portion of analyte atoms will remain in a nonemitting phase bound within the solid particulate. Furthermore, the local absorption of plasma energy required for particle vaporization will not be sufficiently replaced by the diffusion of heat from the larger, surrounding plasma, resulting in localized suppression of plasma temperature or electron density, which can subsequently affect the atomic emission of the analyte species that are also confined to this spatial region due to finite scales of mass transfer. Figure 1 depicts the two scenarios outlined earlier, which might be considered the two limiting cases for plasma–particle interactions pursuant to quantitative LIBS-based particle analysis for a given analytical time scale.

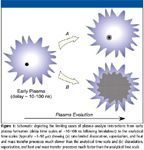
Figure 1

Clearly the roles of heat and mass transfer processes in combination with the rates of particle vaporization and dissociation are of critical importance to understanding and optimizing the analyte response for LIBS-based aerosol particle analysis. Along these lines of thought, contemporary research studies have sought to quantify the plasma–analyte interactions, and will be the focus of the remainder of this review.

Figure 2

Probing Plasma–Particle Interactions

The relative simplicity and analyte sensitivity of LIBS, combined with the ability to analyze individual particles from an aerosol system, have motivated a wide range of applications, as briefly described earlier. Figure 2 nicely illustrates the promise of LIBS for quantitative aerosol analysis, depicting a spectrum obtained from a single aerosolized B. atrophaeous spore of about 1 μm in diameter, as adopted from work of Dixon and Hahn (41). The two calcium atomic emission lines correspond to about 4 fg of calcium, thereby demonstrating the femtogram limits of detection available with LIBS for select analyte species. However, the process of transforming from a solid, micrometer-to submicrometer-sized particle to individual neutral atoms and ions involves a complex set of physical processes, as summarized in Figure 3.

Figure 3

As a starting point to understand the relevant issues, researchers have taken a more critical look at the upper particle size limits for quantitative analysis of aerosol particles. Carranza and Hahn (5) explored the vaporization and analyte response of individual silica microspheres in an aerosolized air stream. The upper size limit for complete particle vaporization was found to correspond to a silica particle diameter of 2.1 μm for a laser pulse energy of 320 mJ, as determined by the deviation from a linear mass response of the silicon atomic emission signal for progressively increasing diameters of silica microspheres. Their findings were discussed in concert with factors that may influence the vaporization dynamics of individual aerosol particles, and it was considered that the larger particles might not have sufficient plasma residence time to completely vaporize on the analytical time scale that the emission measurements were recorded, namely, 35 μs following plasma initiation.

A more recent study examined the complete vaporization of carbon-rich particles (specifically glucose particles and sodium hydrogenocarbonate particles) in a laser-induced plasma, and reported an upper size limit of 5 μm for complete vaporization (6). A larger limiting size with the carbon-rich particles as compared with the silicon particles (2.1 μm versus 5 μm) most likely reflects the marked difference in melting points and volatility when comparing the more refractory silicon particles to the more volatile carbon-rich particles.

While the exact upper size limits for quantitative analysis will always depend upon the nature of the analyte and the specific experimental configuration (for example, laser energy, wavelength, and configuration), these studies have helped to answer the important question of the limiting particle size for quantitative analysis, although additional studies are necessary to elucidate the underlying physics of this 2–5 μm size limit.

To gain a deeper knowledge of the laser-induced plasma–particle interactions as related to aerosol particle analysis, researchers have turned to spatially resolved spectral measurements and direct plasma imaging. Hohreiter and Hahn (45) were the first to directly image analyte species as they vaporized and dissociated from an individual glass microsphere, and subsequently diffused into the surrounding plasma. As shown in Figure 4, their study provided direct evidence of the actual time scales of particle vaporization and analyte diffusion, revealing that the plasma–particle interaction is initially limited to a spatial region about the particle. Analysis yielded diffusion coefficients of the calcium atoms ranging from 0.06 to 0.02 m² /s over the delay times of 2–30 μs, respectively, following plasma initiation, and a time scale of 15–20 μs for complete dissociation of the 2-μm (mean diameter) glass microspheres. In several related studies, Lithgow and Buckley (49–51) explored the influence of the spatial location on the atomic emission from aerosol particles in laser-induced plasmas. The spatially resolved spectral measurements revealed a variability in analyte signal with the location of particles within the plasma. Additionally, they proposed the use of spatially resolved measurements to maximize the particle detection efficiency.

Figure 4

The finding of spatially localized analyte species following particle vaporization with LIBS-based aerosol analysis raises the question of the importance of localized plasma perturbations due to loss of plasma energy to vaporization and dissociation processes. Previous studies revealed no changes to the overall plasma (spatially averaged bulk plasma) properties of temperature and electron density due to the presence of aerosol particles (52). However, the same study revealed a dependence of the analyte signal (carbon in the study) on the source of the analyte when comparing particulate-derived carbon to gaseous-derived carbon (namely CO₂ and CH₄). Together, such findings suggest the examination of the problem from the other point of view, namely, changes to the localized plasma properties about the particle-derived analyte species, rather than changes to the bulk plasma. Diwakar and colleagues (53) examined the issue of localized plasma perturbations by examining the analyte signal from a range of multicomponent submicron-sized aerosol particles. Analyte species examined included sodium, magnesium, and cobalt with the addition of the elements copper, zinc, or tungsten at mass ratios from 1:9 to 1:19 (analyte-to-concomitant species). Their measurements revealed a perturbation in localized plasma temperature, which was attributed to the loss of plasma energy required to vaporize and ionize the aerosol particle mass. The measurements provide direct evidence of a matrix effect for aerosol particles, as the resulting analyte signals were affected by additional particle mass, as attributed to localized plasma perturbations in the vicinity of the particle. The resulting perturbations to analyte response were minimized at longer plasma delay times (~60 μs), which is attributed to the allowance of sufficient time for complete vaporization of the analyte particles in combination with sufficient time for the analyte atoms to thoroughly diffuse throughout much of the plasma, thereby equilibrating with the overall bulk plasma conditions. The authors concluded that quantitative LIBS analysis of aerosol particles should be performed with careful attention given to the temporal plasma evolution and analytical time scales, and noted that the finite time-scales of particle dissociation and heat and mass transfer are equally important.

Much of the LIBS research has been driven by experimental studies, although numerical simulations and modeling studies can provide valuable insight into the plasma dynamics (54–71). A recent study by Dalyander and coworkers (71) specifically addressed the time scales of heat and mass transfer with laser-induced plasmas for the application of aerosol particle analysis. The model included simultaneous solution of the heat and mass transfer equations using physical models for the plasma thermal conductivity and for the mass diffusivity of the analyte species (calcium and magnesium in the study). The analysis revealed significant species gradients due to the finite scales of mass transfer, and localized temperature perturbations due to energy requirements of particle dissociation and ionization. A very useful parameter for directly comparing the time scales of heat and mass transfer is the dimensionless Lewis number, defined as the ratio of the thermal diffusivity to the mass diffusivity. Figure 5 presents the volume-averaged Lewis number from the numerical simulations for the species calcium and magnesium as a function of time following plasma initiation. The Lewis number ranges from about 4 to 1, with a Lewis number of essentially unity for plasma delay times beyond 5 μs following breakdown. The findings are in excellent agreement with the experimental results discussed earlier and indicate that the time scales of heat and mass diffusion are comparable and finite. In aggregate, such a near-unity Lewis number is indicative of a finite rate of diffusion of heat into the region of particle dissociation, and of a comparable, finite rate of diffusion of analyte species away from the particle region. Such a scenario, therefore, suggests initial suppression of local plasma temperature, as energy that is utilized for particle dissociation can only be replaced over a finite time scale.

Figure 5

Summary and Future Directions

The above narrative is intended to make two key points about the evolution of laser-induced breakdown spectroscopy for the specific application of aerosol analysis. First, a new technique can move into the analytical community with an initial set of key assumptions (for example, complete and rapid particle dissociation, or independence of the analyte signal on analyte source) that have not yet been evaluated rigorously. What often follows is a parallel path of fundamental investigations in concert with many applied studies examining the applicability and performance of the analyte scheme. Second, only through fundamental scientific studies can the underlying physics behind the key assumptions be evaluated critically, after which the optimal paths forward can be identified with the ultimate goal of a widely accepted analytical technique. One can propose that the last decade has seen LIBS-based aerosol analysis in this second phase, in which the key physical processes are being unraveled. It is also noted that beyond LIBS, plasmas are a central component of many of today's leading analytical methods for chemical analysis, microanalysis, and materials characterization, including laser-ablation inductively coupled plasma–mass spectrometry (LA-ICP-MS) and inductively coupled plasma–atomic emission spectroscopy (ICP-AES). Hence, related studies of plasma–analyte interactions in inductively coupled plasmas remain relevant to the problems studied in the context of LIBS (72,73). In all of these analytical techniques, the complex plasma–analyte interactions are directly related to the ultimate analyte response, and to the ultimate quality of the results provided by the techniques. It is envisioned that by understanding and overcoming the problems associated with the plasma–particle interface, LIBS will successfully move forward in the analytical community.

Acknowledgments

The author would like to acknowledge the significant contributions made by his current and former graduate students to the topic of LIBS-based aerosol analysis and offer his gratitude for their fine efforts: Jorge Carranza, Vincent Hohreiter, Ken Iida, P. Soupy Dalyander, Michael Asgill, Philip Jackson, Bret Windom, and Prasoon Diwakar. This work was supported in part by the National Science Foundation through grant CHE-0822469, as part of the Plasma-Analyte Interaction Working Group (PAIWG), a collaborative effort of the University of Florida, Federal Institute of Materials Research and Testing (BAM) in Berlin, and the Institute for Analytical Sciences (ISAS) in Dortmund, jointly funded by the NSF and DFG.

David W. Hahn is with the Department of Mechanical & Aerospace Engineering, Plasma Analyte Interaction Working Group, University of Florida, Gainesville, Florida.

References

(1) L.J. Radziemski, T.R. Loree, D.A. Cremers, and T.M. Hoffman, Anal. Chem. 55, 1246–1252 (1983).

(2) M. Essien, L.J. Radziemski, and J. Sneddon, J. Anal. At. Spectrom. 3, 985–988 (1988).

(3) D.K.Ottesen, J.C.F. Wang, and L.J. Radziemski, Appl. Spectrosc. 43, 967–976 (1989).

(4) S. Yalcin, D.R. Crosley, G.P. Smith, and G.W. Faris, Hazardous Waste & Hazardous Materials 13, 51–61 (1996).

(5) J.E. Carranza and D.W. Hahn, Anal. Chem. 74, 5450–5454 (2002).

(6) E. Vors and L. Salmon, Anal. Bioanal. Chem. 385, 281–286 (2006).

(7) L. Dudragne, Ph. Adam, and J. Amouroux, Appl. Spectrosc. 52, 1321–1327 (1998).

(8) M. Tran, B.W. Smith, D.W. Hahn, and J.D. Winefordner, Appl. Spectrosc. 55, 1455–1461 (2001).

(9) M. Casini et al., Laser Particle Beams 9, 633–639 (1991).

(10) W.L. Flower et al., Fuel Process. Technol. 39, 1–3 (1994).

(11) R.E. Neuhauser, U. Panne, R. Niessner, G.A. Petrucci, P. Cavalli, and N. Omenetto, Anal. Chim. Acta 346, 37–48 (1997).

(12) D.W. Hahn, W.L. Flower, and K.R. Hencken, Appl. Spectrosc. 51, 1836–1844 (1997).

(13) D.L. Monts et al., Combust. Sci. Technol. 134, 103–126 (1998).

(14) R.E. Neuhauser, U. Panne, R. Niessner, and P. Wilbring, Fresenius' J. Anal. Chem. 364, 720–726 (1999).

(15) H.S. Zhang, F.Y. Yueh, and J.P. Singh, Appl. Opt. 38, 1459–1466 (1999).

(16) M.H. Nunez, P. Cavalli, G. Petrucci, and N. Omenetto, Appl. Spectrosc. 54, 1805–1816 (2000).

(17) S.G. Buckley, H.A. Johnsen, K.R. Hencken, and D.W. Hahn, Waste Management 20, 455–462 (2000).

(18) H.S. Zhang, F.Y. Yueh, and J.P. Singh, J. Air Waste Manage. Assoc. 51, 681–687 (2001).

(19) L.G. Blevins, C.R. Shaddix, S.M. Sickafoose, and P.M. Walsh, Appl. Opt. 42, 6107–6118 (2003).

(20) K. Lombaert et al., Plasma Chem. Plasma Process. 24, 41–56 (2004).

(21) K. Iida , C.Y. Wu, and D.W. Hahn, Combust. Sci. Technol. 176, 453–480 (2004).

(22) A. Molina et al., Spectrochim. Acta, Part B 60, 1103–1114 (2005).

(23) A. Molina et al., Appl. Opt. 45, 4411–4423 (2006).

(24) R. Yoshiie et al., Powder Technol. 180, 135–139 (2008).

(25) D.W. Hahn, Appl. Phys. Lett. 72, 2960–2962 (1998).

(26) J.E. Carranza, B.T. Fisher, G.D. Yoder, and D.W. Hahn, Spectrochim. Acta, Part B56, 851–864 (2001).

(27) G.A. Lithgow, A.L. Robinson, and S.G. Buckley, Atmos. Environ. 38, 3319–3328 (2004).

(28) B. Hettinger, V. Hohreiter, M. Swingle, and D.W. Hahn, Appl. Spectrosc. 60, 237–245 (2006).

(29) D. Mukherjee, A. Rai, and M.R. Zachariah, J. Aerosol Sci. 37, 677–695 (2006).

(30) D. Mukherjee and M.D. Cheng, Appl. Spectrosc. 62, 554–562 (2008).

(31) T. Amodeo et al., Spectrochim. Acta, Part B 63, 1183–1190 (2008).

(32) D. Mukherjee and M.D. Cheng, J. Anal. At. Spectrom. 23, 119–128 (2008).

(33) K. Park, G. Cho, and J.H. Kwak, Aerosol Sci. Technol. 43, 375–386 (2009).

(34) D.W. Hahn and M.M. Lunden, Aerosol Sci. Technol. 33, 30–48 (2000).

(35) J.E. Carranza, K. Iida, and D.W. Hahn, Appl. Opt. 42, 6022–6028 (2003).

(36) S. Morel, N. Leone, P. Adam, and J. Amouroux, Appl. Opt. 42, 6184–6191 (2003).

(37) A.C. Samuals, F.C. DeLucia, K.L. McNesby, and A.W. Miziolek, Appl. Opt. 42, 6205–6209 (2003).

(38) A.R. Boyain-Goitia, D.C.S. Beddows, B.C. Griffiths, and H.H. Telle, Appl. Opt. 42, 6119–6132 (2003).

(39) J.D. Hybl, G.A. Lithgow, and S.G. Buckley, Appl. Spectrosc. 57, 1207–1215 (2003).

(40) N. Leone et al., High Temp. Mater. Process. 8, 1–22 (2004).

(41) P.B. Dixon and D.W. Hahn, Anal. Chem. 77, 631–638 (2005).

(42) J.D. Hybl et al., Appl. Opt. 45, 8806–8814 (2006).

(43) E. Gibb-Snyder et al., Appl. Spectrosc. 60, 860–870 (2006).

(44) N. Leone, G. Fath, and P. Adam, High Temp. Mater. Process. 11, 125–147 (2007).

(45) V. Hohreiter and D.W. Hahn, Anal. Chem. 78, 1509–1514 (2006).

(46) J.E. Carranza and D.W. Hahn, J. Anal. At. Spectrom. 17, 1534–1539 (2002).

(47) V. Hohreiter, A. Ball, and D.W. Hahn, J. Anal. At. Spectrom. 19, 1289–1294 (2004).

(48) B.T. Fisher, H.A. Johnsen, S.G. Buckley, and D.W. Hahn, Appl. Spectrosc. 55, 1312–1319 (2001).

(49) G.A. Lithgow and S.G. Buckley, Spectrochim. Acta, Part B 60, 1060–1069 (2005).

(50) G.A. Lithgow and S.G. Buckley, Appl. Phys. Lett. 87, 011501 (2005).

(51) S. Simpson, G.A. Lithgow, and S.G. Buckley, Spectrochim. Acta, Part B 62, 1460–1465 (2007).

(52) V. Hohreiter and D.W. Hahn, Anal. Chem. 77, 1118–1124 (2005).

(53) P.K. Diwakar, P.B. Jackson, and D.W. Hahn, Spectrochim. Acta, Part B 62, 1466–1474 (2007).

(54) A. Casavola, G. Colonna, and M. Capitelli, Appl. Surf. Sci. 208–209, 85–89 (2003).

(55) G. Colonna, A. Casavola, and M. Capitelli, Spectrochim. Acta, Part B 56, 567–586 (2001).

(56) T.E. Itina, J. Hermann, Ph. Delaporte, and M. Sentis, Appl. Surf. Sci. 208–209, 27–32 (2003).

(57) A. De Giacomo, V.A. Shakhatov, and O. De Pascale, Spectrochim. Acta, Part B 56, 753–776 (2001).

(58) V.I. Mazhukin, V.V. Nossov, M.G. Nickiforov, and I. Smurov, J. Appl. Phys. 93, 56–66 (2003).

(59) J.R. Ho, C.P. Grigoropoulos, and J.A.C. Humphrey, J. Appl. Phys. 79, 7205–7215 (1996).

(60) V.I. Mazhukin, V.V. Nossov, and I. Smurov, J. Appl. Phys. 90, 607–618 (2001).

(61) V.I. Mazhukin, V.V. Nossov, G. Flamant, and I. Smurov, J. Quant. Spectrosc. Radiat. Transfer 73, 451–460 (2002).

(62) G.J. Tallents, J. Phys. B: At. Mol. Opt. Phys. 13, 3057–3072 (1980).

(63) M.W. Stapleton and J.P. Mosnier, Appl. Surf. Sci. 197–198:72–76 (2002).

(64) N. Arnold, J. Gruber, and J. Heitz, Appl. Phys. A 69 (Suppl.), S87–S93 (1999).

(65) L.V. Zhigilei, Appl. Phys. A 76, 339–350 (2003).

(66) M.I. Zeifman, B.J. Garrison, and L.V. Zhigilei, J. Appl. Phys. 92, 2181–2193 (2002).

(67) A. Bogaerts, Z. Chen, R. Gijbels, and A. Vertes, Spectrochim. Acta, Part B 58, 1867–1893 (2003).

(68) M. Capitelli, A Casavola, G. Colonna, and A. De Giacomo, Spectrochim. Acta, Part B 59, 271–289 (2004).

(69) I.B. Gornushkin et al., J. Appl. Phys. 100, 073304 (2006).

(70) A.Y. Kazakov et al., Appl. Opt. 45, 2810–2820 (2006).

(71) P.S. Dalyander, I.B. Gornushkin, and D.W. Hahn, Spectrochim. Acta, Part B 63, 293–304 (2008).

(72) C.C. Garcia, H. Lindner, and K. Niemax, J. Anal. At. Spectrom. 24, 14–26 (2009).

(73) S. Groh, C.C. Garcia, A. Murtazin, V. Horvatic, and K. Niemax, Spectrochim. Acta, Part B 64, 247–254 (2009).