Polychromatic and Microfocused X-ray Radiation for Traceable Quantitative X-ray Fluorescence Analysis

X-ray fluorescence (XRF) analysis is used in environmental, geological, and biomedical applications for the elemental speciation of samples. Chemical or physical traceability chains are used for the quantification of the absolute elemental content within a sample; they also assess, in a reliable manner, the chemical composition of a sample to possibly meet regulatory requirements. The physical traceability chain relies on absolute knowledge of the X-ray spectral distribution used for the excitation of the XRF instrument and is currently used at synchrotron radiation facilities. We discuss the transfer of the physical traceability chain to laboratory-based X-ray sources, which are often polychromatic, with the view to generate wider application of quantitative X-ray fluorescence analysis. Furthermore, the combination with micro-focusing optics enables the extension of these capabilities to spatially resolved measurements such that samples can be investigated in more detail in cases where localized measurements are required of individual structures or of cells stemming from organic tissue.

Quantitative X-ray fluorescence (XRF) analysis provides traceable access to the absolute elemental composition of a sample in terms of elemental mass deposition (number of atoms per unit area) of the different chemical elements detected. One way to quantify the elemental composition of a sample in absolute terms is using calibration samples to account for unknown instrumental sensitivity. The peculiarity of this approach is that the selected calibration or reference samples need to be representative of the sample under investigation in terms of elemental composition and concentration (sample matrix) and to some extent the morphology as well, to ensure comparability. Alternatively, an internal standard mixture with a predetermined composition can be added to the sample of interest, but care has to be taken to ensure homogeneous intermixing and to use nontoxic and nonvolatile elements that do not have characteristic X-ray lines in the vicinity of the elements that are being quantified. The reference-free approach described here is based on a physical traceability chain and relies solely on the use of calibrated instrumentation.

In the reference-free XRF quantification scheme (1), (radiometrically) calibrated instrumentation and knowledge of atomic fundamental parameters (FPs) involved in the production of X-ray fluorescence is used to derive the elemental mass deposition from the mea- sured count rate of the characteristic lines of one element. The atomic FPs, such as photoionization cross-sections and fluorescence yields, are element-dependent and also largely energy-dependent, but can be taken from literature databases (2) or determined by means of dedicated experiments (3,4). To excite the XRF, tunable monochromatic synchrotron radiation is used, of which the spectral distribution is well-known through the characterization of the beamlines used, whereas the use of radiometrically calibrated photodiodes provides access to the photon flux incident on the sample. For detection of the fluorescence radiation, a radiometrically calibrated silicon drift detector (SDD) and calibrated apertures are used, such that the detection efficiency and the solid angle of detection are accurately known. Following a deconvolution of the measured XRF spectra and using only physical contributions to describe possible background radiation underlying the characteristic X-ray lines, solving the Sherman equation (5) makes it possible to obtain an elemental mass deposition from the modeled count rate.

Reference-Free Quantification in Conjunction with Polychromatic X-ray Radiation

The use of monochromatic X-ray radiation has several characteristics, which distinguishes its use cases from those of polychromatic radiation. For example, the tunability of monochromatized synchrotron radiation may be used to selectively induce or suppress individual electronic transitions. Conversely, polychromatic radiation can be used to ensure simultaneous and efficient excitation of a multitude of different chemical elements. Exemplary fluorescence radiation intensities are shown in Figure 1 for a thin copper foil excited by calculable dipole-white light (dashed blue line) and excited by monochromatized synchrotron radiation (solid red line). Even though the peak intensity is far lower for the polychromatic excitation condition (mainly because of the utilization of a small aperture), the total intensity exceeds that of monochromatic excitation by almost an order of magnitude.

FIGURE 1: Calculated number of copper Kα fluorescence photons emitted by a 2 μm thin copper foil when excited by monochromatic radiation of 9 keV (solid line, red) in comparison to the excitation by polychromatic radiation (dashed line, blue). Both cases are based on real examples of incident photon flux available at PTB beamlines at the synchrotron-radiation facility (BESSY II). The former case has a larger peak value because the latter utilizes a small aperture to accurately define the incidence radiation. Nevertheless, the excitation by polychromatic radiation induces roughly an order of magnitude more fluorescence photons in total.

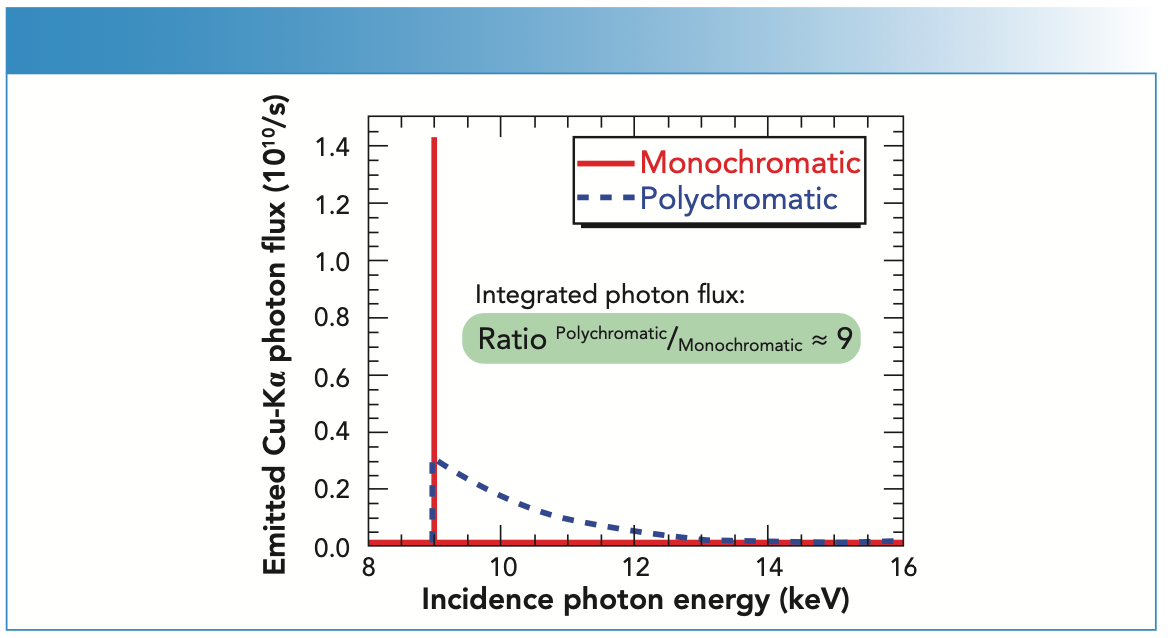
Although these differences are important from a practical viewpoint, the physical principles on which a quantitative, reference-free analysis is based on are identical. In practice, a description of the polychromatic excitation spectrum from a dedicated dipole-white light beamline can be derived from ab initio calculations (based on Maxwell’s equations) quite accurately (6). A reference-free quantification utilizing such calculable polychromatic X-ray radiation should produce quantitative results with roughly the same accuracy as a reference-free quantification based on monochromatic X-ray radiation. Additionally, it should reveal how the reference-free approach could be transferred to more commonly available X-ray radiation sources, such as laboratory setups relying on X-ray tubes.

Toward Reference-Free Quantification with Microfocused Polychromatic Radiation

In conjunction with microfocusing optics, a transition from quantitative elemental analysis towards spatially resolved (quantitative) elemental analysis can be realized. Combined with polychromatic X-ray sources, achromatic focusing optics such as mono- or polycapillary lenses are required. Depending on the source used, either full lenses are needed for point-to-point focusing or half-lenses are required to condense a parallel X-ray beam originating from a collimated source. Although transmission losses in the optics are unavoidable, the gain offered in terms of photons on the sample per unit time and per unit area is a major benefit with respect to simpler pinhole arrangements. Further benefits of poly- and monocapillary optics are their small dimensions and short working distances (typically of the order of millimeters), such that compact setups can be realized, and their high flexibility with respect to the X-ray source in terms of spatial and energetic acceptance. The achromatic character of poly- and monocapillary optics make them usable in conjunction with polychromatic laboratory-based sources, which is greatly beneficial in terms of accessibility and flexibility compared to synchrotron radiation–based sources, for which beamtime can be costly or limited.

In reference-free quantification using polychromatic X-ray sources, the downside of using focusing optics is that the determination of their energy-dependent transmission characteristics is rather complex. Indeed, the modulation in terms of spectral distribution and intensity of the X-ray radiation incident on the sample needs to be measured using adequate detection schemes such that a correct model can be implemented in the quantification scheme. This type of measurement can be done using either a photodiode at a beamline offering tunable monochromatic radiation or using an energy-dispersive detector in conjunction with polychromatic radiation. In both cases, the detectors are positioned directly in the incident X-ray beam and measurements with and without the optics are performed. When using laboratory instrumentation, the second type of measurement can be performed (7), but the high flux at synchrotron radiation facilities usually limits the characterization of the optics to the first type of measurement. For the setup presented, this part of the characterization still needs to be realized.

Position-Resolved XRF Investigations

Experiments were carried out at the dipole-white light beamline (6) in the laboratory of the Physikalisch-Technische Bundesanstalt (8). This beamline provides a polychromatic excitation spectrum corresponding to bending magnet radiation with a critical energy of 2.5 keV originating from a 1.3 Tesla dipole magnet. A 1.4 mm in diameter pinhole was used to spatially confine the bending magnet radiation and a 4 μm thick gold filter was used to eliminate visible light while ensuring good excitation conditions for mid-Z elements.

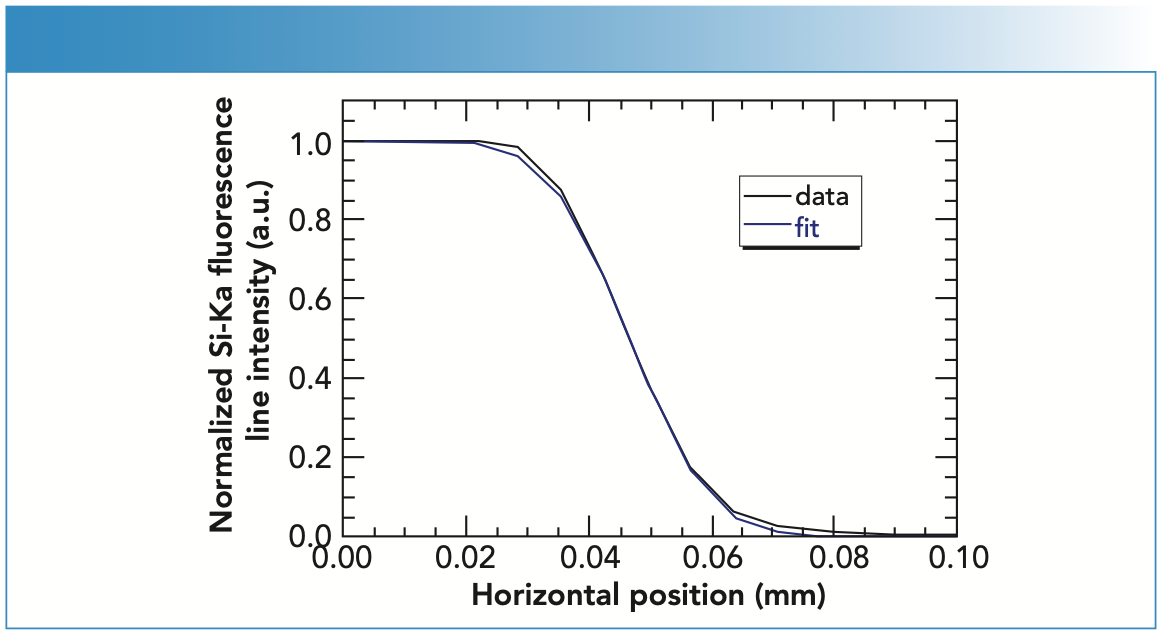
A monocapillary with a platinum coating and a gold beamstop was used. The optics used had an entrance aperture of approximately 1.5 mm in diameter, an exit aperture of about 0.8 mm in diameter, and a working distance of approximately 14 mm. The monocapillary optics were mounted on a five-axis piezo manipulator installed in an ultrahigh vacuum instrument, which can be used as a mobile end station at different synchrotron radiation beamlines and is similar to the one described in the literature (9). The required degrees of freedom for alignment of the optics are three translational and two rotational stages. The accuracy provided by piezo motors is beneficial for the rotational alignment of the optics because minor misalignments can severely impede the transmission of the X-ray radiation. The rotational alignment is performed with respect to two orthogonal axes, which are perpendicular to the incident X-ray beam. The translational alignment is first realized with respect to the two same axes such that the entrance of the optics is positioned centrally in the axis of the synchrotron radiation beam. Finally, the third translational axis makes it possible to adjust the distance between the optics and the sample to the working distance of the optics. The criteria for optimization are minimization of the spot size and enhancement of the transmission. The spot size can be qualitatively monitored using a phosphor screen and a video camera system and quantitatively characterized using a knife-edge scan that allows for investigating the beam profile downstream of the capillary (Figure 2).

FIGURE 2: Example of a knife-edge scan on the border of a silicon wafer. The beam size was determined by performing a least square fit using an error function (that is, normal cumulative distribution function).

The comparison of fluorescence line intensities from a sample detected with and without the monocapillary optics showed a reduction of the incident flux on the sample to approximately 3% for the aluminum K line and to approximately 2% for the whole spectrum. Note that in the case of measuring with monocapillary optics, a large but not determined part of the incident radiation is blocked by the housing and the central beam stop of the optics. For the polychromatic radiation used, this reduction corresponds to the convolution of the energy-dependent transmission and photoionization cross-section factors such that no direct information on the optics can be deduced without knowledge of the incident and transmitted spectral distributions.

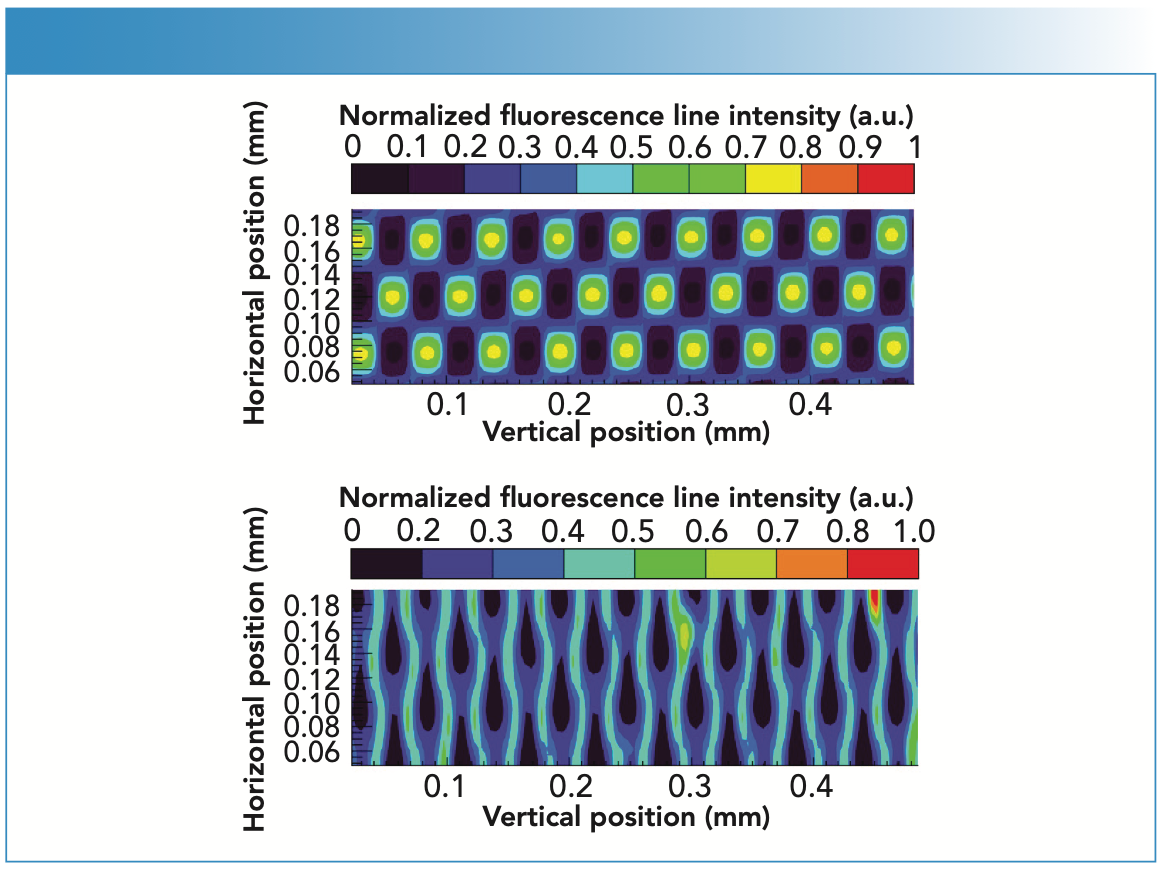
The alignment of the monocapillary optics results in a spot size of about 24 μm x 3 μm horizontally and vertically, respectively. With this beam spot, the element distribution of a tin–silver solder bump array with underlying copper vias was measured by scanning the lateral motor axes. A sample area of about 0.25 mm x 0.5 mm was investigated. This area includes about 50 solder bump structures in a periodic arrangement. The different horizontal and vertical spot size was caused presumably because of non-perfect alignment or different horizontal and vertical divergences of the dipole radiation, and results in an asymmetric shape of the tin–silver structures in the spatial element distribution. To consider the different horizontal and vertical spot size in the characterization of the structures, a maximum entropy deconvolution of the measured element distribution and the spot size was performed. Results are shown in Figure 3 for the tin fluorescence on the left, and the copper fluorescence on the right.

FIGURE 3: Left side: Spatial element distribution of tin of periodically arranged tin–silver structures after a maximum entropy deconvolution based on the respective horizontal and vertical sizes of the incident beam. The asymmetric alignment of the monocapillary optics resulted in varying horizontal and vertical beam sizes. Silver has a similar distribution as tin and is not shown here. Right side: Element distribution of copper.

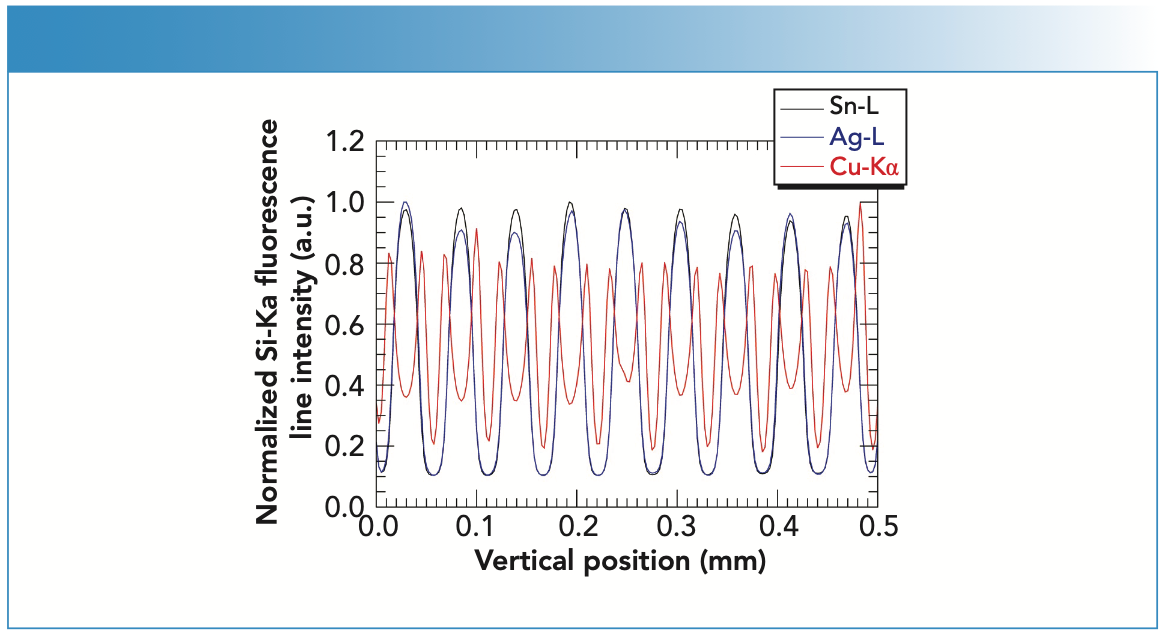
A line cut (subset of the element distribution) of the lower line is shown in Figure 4. From raster scans and closer inspection via cuts in the spatial distribution, a quality inspection can be conducted in terms of similarity of the different solder bumps or as a void inspection. Using intensity variations and intensity ratios as fingerprints, possible defects and inhomogeneities can be detected. In the case of possible defects, larger variations than for perfectly homogeneous samples must be expected.

FIGURE 4: Line scan of the lower line for the elements tin, silver, and copper are shown.

Conclusions

To summarize, the route toward reference-free polychromatic micro-focused XRF experiments has been described. In the present work, a single-bounce monocapillary-focusing optics was used in conjunction with the undispersed radiation from a bending magnet. But this approach is also realized in combination with laboratory-based X-ray sources. This way, the route toward reliable, traceable quantification schemes can be opened up for more commonly available instrumentation. The effort for calibration and characterization is considerable, but access to absolute elemental mass depositions while using polychromatic and micro-focused X-ray radiation provides more accurate information about the local stoichiometry. In addition, access to absolute elemental mass depositions while using polychromatic and micro-focused X-ray radiation leads to improved detection sensitivity as compared to monochromatic excitation. This information can be relevant in geological or biological applications as well as for technologically relevant samples where absolute information about the elemental composition can be correlated with the functionality of a material.

Acknowledgments

The project is supported by the EMPIR program co-financed by the participating states and from the European Union’s Horizon 2020 research and innovation program through grant agreements 20FUN06 MEMQuD (Memristive Devices as Quantum Standard for Nanometrology) and 19ENV08 AEROMET II (Advanced Aerosol Metrology for Atmospheric Science and Air Quality). Weblinks of the European Research projects can be found here: memqud.inrim.it and aerometprojectii.com.

References

(1) B. Beckhoff, J. Anal. At. Spectrom. 23, 845–853 (2008).

(2) T. Schoonjans, A. Brunetti, B. Golosio, M. Sanchez del Rio, V. A. Solé, C. Ferrero, and L. Vincze, Spectrochim. Acta B 66, 776–784 (2011).

(3) P. Hönicke, M. Kolbe, M. Müller, M. Mantler, M. Krämer, and B. Beckhoff, Phys. Rev. Lett. 113, 163001 (2014).

(4) R. Unterumsberger, P. Hönicke, J. L. Colaux, C. Jeynes, M. Wansleben, M. Müller and Burkhard Beckhoff, J. Anal. At. Spectrom. 33, 1003–1013 (2018).

(5) J. Sherman, Spectrochim. Acta 7, 283–306 (1955–1956).

(6) R. Thornagel , R. Klein, and G. Ulm, Metrologia 38, 385–389 (2001).

(7) Y. Kayser, W. Błachucki, J.-Cl. Dousse, J. Hoszowska, M. Neff, and V. Romano, Rev. Sci. Instrum. 85, 043101 (2014).

(8) B. Beckhoff, A. Gottwald, R. Klein, M. Krumrey, R. Müller, M. Richter, F. Scholze, R. Thornagel, and G. Ulm, Phys. Status Solidi B 1434, 1415–1434 (2009).

(9) J. Lubeck, B. Beckhoff, R. Fliegauf, I. Holfelder, P. Hönicke, M. Müller, B. Pollakowski, F. Reinhardt, and J. Weser, Rev. Sci. Instrum. 84, 045106 (2013).

Yves Kayser, Rainer Unterumsberger, André Wählisch, Karina Bzheumikhova, Philipp Hönicke, Claudia Zech, and Burkhard Beckhoff are with the Physikalisch-Technische Bundesanstalt, in Berlin, Germany. Direct correspondence to: yves.kayser@ptb.de ●