Raman Microscopy Analysis of Molecular Orientation in Organic Fibers
How the behavior of polarized Raman spectra can elucidate the microscopic structure of fibers

The processes involved in creating fibers from organic polymers — either man-made or natural — have the potential to orient the molecules in the fibers. Examination of a number of fibers confirms that orientation is a more or less ubiquitous phenomenon that can be detected and quantified by Raman microscopy. In addition, the presence of molecular orientation can mediate the crystallization process which can also be followed with the Raman signatures. Recognizing how to avoid instrumental artifacts while measuring these phenomena can aid in characterizing fibrous materials and in differentiating similar fibers collected as trace evidence in forensics investigations.
Large linear organic molecules exhibit the potential to have their molecular axes more or less aligned during the creation of fibers from these molecules. The degree of molecular alignment can affect the strength of the fibers and their ability to crystallize. The physical properties that are determined by orientation and crystallinity determine which industrial applications such fibers can be used for. For instance, cotton, silk, wool, and man-made fibers such as nylon, polyethylene terephthalate (PET), and Kevlar (aramid fiber, DuPont) are used to make textiles. For ages wool has been known for its warmth, silk for its strength, and cotton for it hygroscopic properties. The man-made fibers mentioned are known for their durability and strength (especially Kevlar). In this article, we indicate how the behavior of the polarized Raman spectra can elucidate the microscopic structure of these fibers.

Fran Adar
Instrument Behavior
All dispersive Raman instruments are, by definition, grating-based; meaning that the spectral lines are dispersed (that is, separated) by a diffraction grating. Such instruments have wonderful properties, with the exception that the dichroism of the grating's reflectivity for the two polarizations (Eperp=E ⊥ and Epar= E|| which denotes the electric vector being either perpendicular or parallel to the grooves of the grating) can strongly affect the relative intensities of the spectra recorded. The reflectivity spectral profiles are determined by the grating groove density (that is, the groove width) and the shape of the groove. The result, which determines the type of curves shown in Figure 1, is totally the result of grating physics. To the extent that the groove profile can be controlled by the shape of the diameter of the tool used to cut the grating, or an etching process of a holographically ruled grating, these curves can be optimized for a particular type of measurement. For instance, let's suppose you want to measure a series of polymer spectra using a 532-nm laser on a short focal length (200-mm) spectrograph. An 1800-g/mm grating will provide about 1500 cm-1 coverage in the fingerprint region (~1.5 cm-1 /pixel) and a 1200-g/mm grating will provide about 2600 cm-1 coverage (~2.6 cm-1 /pixel). On a long focal length spectrograph (800 mm), a 600-g/mm grating will provide 1500 cm-1 (~1.5 cm-1 /pixel), and a 300 g/mm-grating will provide about 3100 cm-1 (~3.1 cm-1 /pixel).

Figure 1: Grating reflectivity measurements, from top to bottom, of a 300-, 600-, 1200-, and 1800-g/mm grating. Each grating measurement was performed for E⥠and E|| with E⥠higher at longer wavelength. The green to red arrow at the bottom indicates the Raman range for a full 4000 cm-1 spectrum excited at 532 nm.
But in addition to effecting the dispersion, the choice of the grating affects the relative response at different wavelengths and for different polarizations. Figure 1 shows typical reflectivity curves for four gratings whose groove densities match what was discussed in the previous paragraph. Of particular interest is the polarization response, which is indicated by two curves for each grating.
For each grating there are two curves (the 1800-g/mm grating at the bottom shows three curves, the third being the average of the other two) with a crossover point. On the long wavelength side of the crossover point the higher reflectivity corresponds to E ⊥ . The most important point to notice is that the reflectivity curves for the two polarizations from the low groove density gratings (300 and 600 g/mm on top) are almost identical over the Raman range indicated by the right-pointing arrow color-coded green to red. However, the reflectivity curves for E ⊥ and E|| for the 1200- and 1800-g/mm gratings (second from bottom and bottom) are quite different with ratios as large as ~3:1 for the 1800-g/mm grating at 650 nm. What this means is that if the goal is to measure orientation effects by measuring polarization differences, it is quite important to eliminate polarization artifacts in the measurements caused by the grating properties. This can normally be done by controlling the Raman polarization — either keeping it fixed or adding a scrambler behind the polarization analyzer.
Measurement Protocol
Because the instrument response for different polarizations can be quite different, it is important to fix the sample in a reproducible manner, with its axes consistent with the instrument axes. Figure 2 illustrates how we make our measurements.
Although the polarization directions are labeled H and V in the figure (for horizontal and vertical, respectively), it should be pointed out that at the microscope stage everything is horizontal. H and V actually refer to the polarization orientation behind the microscope and the slit orientation (H or V) has to be noted as well. For most microscope configurations, H will be what we have called EW (east-west) and V will be what we have called NS (north-south) at the sample. To properly predict the instrument behavior following the grating reflectivity, it is necessary to know the orientation of the grooves. This is easy. For any grating-based spectrograph, the grooves are parallel to the entrance slit. If the instrument has a V slit, then E ⊥ is H.
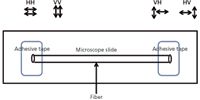
Figure 2: Schematic illustrating how a fiber is mounted for measurement. The arrows at the top indicate the polarization combinations that are available for measurement.
We still need to make contact with the fiber characteristics. Normally the Raman tensor is described in rectilinear coordinates (x, y, and z), which is fine when one is dealing with single crystals. In the case of fibers, there is (at least approximately) axial symmetry. I have found that the easiest way for me to keep track of orientation conditions is to define the fiber axis as Z, but then to define the two orthogonal axes as R and R′ because the x and y axes will occur randomly in the fiber. When reading the literature, keep in mind that most workers continue to use the rectilinear coordinates rather than the uniaxial ones.
So, in Figure 2, the fiber axis is Z, EW is Z and NS is R. Under most conditions we only measure ZZ and RR because ZR and RZ tend to be weaker and provide less information. There are two exceptions:
Because the Raman tensor is symmetric, the RZ spectrum should be exactly the same as the ZR spectrum. By making these two measurements and determining if they are different, you will be able to estimate how much polarization artifact is in your measurement (hopefully not too much). Figure 3 shows the polarized spectra of poly(L-lactic acid) (PLLA, a biodegradable polymer that is really an ester, but with acid end groups) and illustrates the equivalence of the ZR and RZ spectra.

Figure 3: Polarized Raman spectra of a PLLA fiber. From top to bottom the polarization conditions were VV, HH, HV, and VH. These spectra were recorded with an 1800-g/mm grating that has significant polarization effects that were eliminated with the use of a scrambler, as evidenced by the equivalence of the HV and VH spectra at the bottom of the figure.
The other exception is that of polyethylene. Curiously the strongest band in the fingerprint region of polyethylene is the twisting mode near 1300 cm-1 . But its symmetry determined by the Raman tensor is off-diagonal. In highly oriented fibers of polyethylene, this band only appears with reasonable intensity in the RZ or ZR configuration. Figure 4 shows the polarized spectra of a fiber of Dyneema (DSM Dyneema), an ultrahigh- molecular-weight polyethylene (UHMWPE) and illustrates this point.

Figure 4: Polarized Raman spectra of a Dyneema (high molecular weight, highly oriented polyethyelene) fiber oriented in the NS orientation (note that this fiber was not oriented according to the protocol noted in the text, but by keeping track of the fiber orientation relative to the instrument, the results are still consistent with expectations). From top to bottom the polarization conditions are RR, ZZ, and ZR. The arrow indicates the band that is maximized in the off-diagonal condition (ZR).
Examples of Fibers Exhibiting Polarization–Orientation Effects
Polyacrylonitrile, polyethylene, and polypropylene — these fibers were selected because of their chemical similarity. Polypropylene builds on polyethylene with the replacement of a proton on every other carbon atom of the backbone with a propyl group. Polyacrylonitrile builds on polyethylene by adding a pendant nitrile group (-C≡N) on every other carbon atom. Unlike polypropylene where the geometry can be controlled (isotactic, syndiotactic, and atactic) by controlling the catalytic process, that is apparently more difficult in the case of polyacrylonitrile, which is probably why the bands are so much broader. The polarized Raman spectra of these fibers are shown in Figure 5 where the ZZ spectra are displayed in a bolder hue of the color selected for each species.

Figure 5: Polarized spectra (ZZ and RR) of fibers of polyacrylonitrile, polyethylene, and polypropylene. To facilitate the evaluation of these results, the ZZ spectra are plotted in a darker shade of the color selected for each fiber type.
In all cases, there is strong polarization dependence in these spectra which means that the organic molecules are well aligned. For polypropylene and polyacrylonitrile the ZZ spectra tend to dominate the RR spectra throughout the recorded regions. Note that for polyethylene the RR spectrum has stronger bands in the CH region. Interpretation of these results requires detailed analysis of the structure and symmetry of the species in these fibers, beyond the scope of an article such as this. But clearly these spectra all indicate significant molecular orientation.
Figure 6 shows polarized spectra of a set of three man-made fibers: Kevlar (poly-paraphenylene terephthalamide), PET (poly[ethylene terephthalate]), and nylon 6-6. Kevlar has a yellowish tinge because of the near-UV electronic absorption. Due to this absorption the Raman spectrum is strongly enhanced; because the π system is coincident with the molecular–fiber axis the spectrum is enhanced for light polarized in the axis. In the spectra of the nylon, most of the bands are more intense in the ZZ condition, with the exception of the amide I band near 1650 cm-1 , which is stronger in the RR condition.
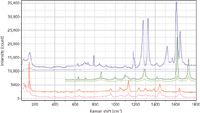
Figure 6: Polarized Raman spectra (ZZ and RR) of synthetic textile fibers known for their strength. From top to bottom, Kevlar, polyethylene terephthalate, and nylon 6-6. In each pair, the upper trace, displayed in a darker hue, represents the ZZ spectrum, whereas the lower trace is the RR spectrum.
Figure 7 shows the spectra of three natural fibers used in textiles: silk, wool, and cotton. Silk and wool are both proteins, but the spectra reveal some significant differences. The structure of silk is mainly β sheet and wool is α helix, which refers to the configuration of the peptide bond and consequently affects the observed frequency shift of the amide I band (1665 cm-1 vs. 1660 cm-1 ). Wool, like hair, contains L-cystine amino acids that can cross-link forming a disulfide detected near 500 cm-1 . For both, the presence of NH groups in the protein can be monitored by observing the broad band near 3290 cm-1 . On the other hand, cotton is composed of cellulose whose OH stretch can be observed near 3450 cm-1 . In addition, this cotton specimen contains titania particles, as evidenced by the line near 144 cm-1 (anatase) added for their brightening properties.

Figure 7: Polarized Raman spectra (from top to bottom) of silk, wool, and cotton, with the ZZ component of each pair displayed in a darker hue, above the RR component.
Detecting Varying Degrees of Orientation and Crystallinity
A study done a while ago on spin-oriented fibers of PET, spun from the melt at varying take-up-speeds, indicated that as the orientation increased so did the polarization behavior of the Raman spectrum. In addition, it was known that at speeds higher than 4000 m/min, crystallization began to occur, and this could be monitored in the width of the carbonyl band near 1728 cm-1 . Figure 8 shows some results from this study.
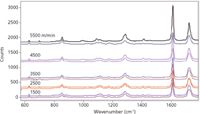
Figure 8: Polarized Raman spectra of PET fibers spun at increasing take-up-speeds, from 1500 to 5500 m/min (bottom to top). With increasing take-up-speeds, the polarization differences increased, and the carbonyl band near 1728 cm-1 sharpened, indicating the onset of crystallization.
Summary
The polarized Raman spectra of a collection of fibers indicated that molecular orientation in fibers is an almost universal phenomenon. Experimental methods designed to produce reliable polarization and orientation measurements have been described. Raman spectra of a series of PET fibers spun with increasing take-up-speed also showed that the degree of polarization can be correlated with the degree of orientation, which makes it possible to use Raman to characterize fibrous materials.
Acknowledgments
Fibers of PET were provided by Herman Noether. Fibers of polyacrylonitrile and PLLA were provided by Zohar Ophir of the New Jersey Institute of Technology. The Dyneema fiber was provided by Neil Everall of Intertek.
Fran Adar is the Worldwide Raman Applications Manager for Horiba Jobin Yvon (Edison, New Jersey). She can be reached by e-mail at fran.adar@horiba.com.
Reference
(1) F. Adar and H. Noether, Polymer 26, 1935–1943 (1985).

A Proposal for the Origin of the Near-Ubiquitous Fluorescence in Raman Spectra
February 14th 2025In this column, I describe what I believe may be the origin of this fluorescence emission and support my conjecture with some measurements of polycyclic aromatic hydrocarbons (PAHs). Understanding the origin of these interfering backgrounds may enable you to design experiments with less interference, avoid the laser illuminations that make things worse, or both.
Raman Microscopy for Characterizing Defects in SiC
January 2nd 2025Because there is a different Raman signature for each of the polymorphs as well as the contaminants, Raman microscopy is an ideal tool for analyzing the structure of these materials as well as identifying possible contaminants that would also interfere with performance.