Reducing the Effects of Interferences in Quadrupole ICP-MS

Since its inception as an analytical technique, inductively coupled plasma–mass spectrometry (ICP-MS) has gained popularity due to its ability to rapidly measure trace levels of most elements on the periodic table. As the technique has matured, new advances have allowed ever-lower levels to be measured, limited primarily by the cleanliness of the laboratory environment and reagents.

However, there has been one obstacle that has continuously plagued the technique: interferences. Although ICP-MS has relatively few interferences compared to ICP-optical emission spectrometry (OES), they are problematic when measuring low levels. Much time and effort has been devoted to removing the effects of interferences, including the development of new classes of instruments: high-resolution ICP-MS and cell-based ICP-MS. This article will discuss various manners of removing the effects of interferences for analyses performed on quadrupole ICP-MS instruments, both with and without cells.

Types of Interferences

There are two main types of interferences: isobaric and polyatomic. Isobaric interferences refer to different elements whose isotopes share a common mass. For example, both Fe and Ni have isotopes at mass 58. Therefore, any signal measured at m/z 58 will have contributions from both Fe and Ni.

Polyatomic interferences result from the combination of two or more isotopes from different elements, which usually occur in the plasma. The elements that form the polyatomic interferences usually result from the sample matrix, sample diluent, and argon itself. An example of a polyatomic interference is ArCl⁺ on As⁺ , both of which occur at m/z 75. The ArCl⁺ forms from a combination of Ar in the plasma and Cl from the sample matrix or diluent; if no Cl is present, then ArCl⁺ will not form, and As will be interference-free.

The Easy Solution . . . : Because ICP-MS has the ability to measure multiple isotopes, the easy solution is to measure another isotope that does not suffer from interferences. For the Fe/Ni example, this would be easy: ⁶⁰ Ni does not suffer from any isobaric interferences. Because all elements (with the exception of In) have at least one isotope that does not have an isobaric interference, this would appear to be a simple solution.

. . . Isn't Always So Easy: Unfortunately, the solution isn't always this simple: ⁶⁰ Ni suffers from the polyatomic interference CaO⁺ , which originates from calcium-containing samples. Also consider the ArCl⁺ interference on As: As has only one isotope, so choosing As isotope without interference is not an option.

But There Is Another Solution: We can take advantage of the fact that most elements have multiple isotopes to correct mathematically for both isobaric and polyatomic interferences. By considering the natural abundance of different isotopes and measuring the intensity of a noninterfered isotope, we can calculate the extent of the interference and subtract this contribution to yield the concentration.

Let's look at Cd as an example. The most abundant isotope of Cd is m/z 114 (28.73%). But Sn also has a minor isotope at m/z 114 (0.65%). This means that any intensity at m/z 114 is a contribution from both Cd and Sn. However, Sn has other isotopes which are interference-free, such as m/z 118 (24.23%). Therefore, we can measure Sn at m/z 118 and calculate its contribution to the signal at m/z 114.

Let's look at this in mathematical terms:

I(m/z 114) = I(¹¹⁴ Cd) + I(¹¹⁴ Sn) [1]

where I = intensity

Rearranging terms:

I(¹¹⁴ Cd) = I(m/z 114) − I(¹¹⁴ Sn) [2]

To figure out the contribution of Sn to the signal at m/z 114, we take into account the natural abundance of both ¹¹⁴ Sn and ¹¹⁸ Sn and the signal intensity of ¹¹⁸ Sn:

I(¹¹⁴ Sn) = [A(¹¹⁴ Sn)/A(¹¹⁸ Sn)] × I(¹¹⁸ Sn) [3]

where A = abundance

or

I(¹¹⁴ Sn) = [0.65/24.23] × I(¹¹⁸ Sn) [4]

I(¹¹⁴ Sn) = 0.0268 × I(¹¹⁸ Sn) [5]

Substituting this expresession into equation 2 yields the following:

I(¹¹⁴ Cd) = I(m/z 114) − 0.0268 × I(¹¹⁸ Sn) [6]

This equation can be entered into the instrument software so that the correction is automatically performed online.

This strategy is also applicable to polyatomic interferences, where alternate isotopes of the interference can be measured. For example, Cl contains two isotopes: m/z 35 (75.77%) and m/z 37 (24.23%). Therefore, if correcting for the ³⁵ Cl⁴⁰ Ar⁺ interference on As at m/z 75, the signal for the ³⁷ Cl⁴⁰ Ar⁺ ion at m/z 77 can be used, as described earlier.

Correction equations work well and can correct for a wide range of interferences and concentrations. However, they do have their limitations. First, if a correction equation is used but there is no interference, the equations tend to over-correct, leading to low or negative concentrations. Also, if the interference concentrations are very high, the equations might not compensate for the elevated levels adequately. Finally, equations can become complicated if the alternate isotope also has an interference. For example, consider the ClAr⁺ interference on As described above. The ³⁷ Cl⁴⁰ Ar⁺ ion at m/z 77 would be used for the correction. However, because Se also has an isotope at m/z 77 (7.63%), an additional term must be used to correct for the isobaric interference on the polyatomic interference. This can be accomplished by using the ⁸² Se isotope (8.73%), which yields a final equation of:

I(⁷⁵ As) = I(m/z 75) − 3.127 × [I(⁷⁷ Se) − [0.874 × I(⁸² Se)]] [7]

Despite their complexity, correction equations work well when measuring moderate analyte concentrations, generally more than 1 ppb. Correction equations have even been published as part of regulated methods, such as U.S. EPA Methods 200.8 and 6020 for the analysis of waters, soils, and solid wastes. Therefore correction equations are a valid, well-established, and well-understood way to correct for interferences.

But for analysis of lower concentration levels, other options may be considered.

How About Removing the Matrix? It stands to reason that if the matrix can be removed from the sample without removing the analyte, then polyatomic interferences will not be a problem. Toward that end, much work has been done for both online and offline matrix-removal techniques. Online versions generally involve passing the sample through a low-pressure column that has a specific affinity for cations, so that all cations stick to the column. The column is then flushed two more times: once to remove the ions of interest and once to remove the cation interferences. This process is represented in Figure 1. This technique has the added advantage of preconcentrating the analytes of interest, so that lower levels can be measured. Much work has been done with this technique and several commercial versions are now available.

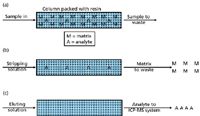
Figure 1: A representation of the process of matrix separation: (a) The sample passes over a column, where both the matrix and analytes adhere to the column. (b) A stripping solution passes over the column and washes the matrix components to waste. (c) An eluent solution passes over the column transporting the analyte components to the ICP-MS system for analysis.

However, online matrix removal also has its drawbacks. First, the chemistry of the column must be matched to that of the sample, meaning that a single column type cannot be used for all sample types. Also, the columns age over time and must be replaced eventually, which means that over the lifetime of the column, QC results must be monitored closely to know when the column is not performing adequately. Nevertheless, this technique has met with success.

The offline version of matrix removal involves removing the analytes from the matrix before analysis. The most popular methods for accomplishing this are through liquid–liquid or solid-phase extraction. Again, this technique has been successful but faces some of the same limitations as the online technique: the chemistry of the extracting medium must be matched to the sample and the extracting medium must be monitored over time for performance degredation.

How About Removing the Interferences in the ICP-MS System Itself? This is certainly possible, but it requires different ICP-MS instrumentation. The two most common techniques are high-resolution ICP-MS and cell-based ICP-MS. High-resolution instruments use electric or magnetic fields to separate ions of different masses instead of quadrupoles. However, a discussion of high-resolution instruments is beyond the scope of this work. Instead, cell-based ICP-MS will be discussed in more detail.

Cell-based ICP-MS employs the use of a gas-filled cell positioned between the ion optics and the analyzer quadrupole. Depending upon the instrument configuration, this cell can be a quadrupole, hexapole, or octopole. The principle of operation is that ions from plasma enter the cell and interact with gas within the cell. Interfering and analyte ions interact differently with the gas, and only the analyte ions pass through the cell to the analyzer quadrupole, as shown in Figure 2. In this way, interferences are removed. These cell-based instruments can be operated in both collision and reaction modes. .

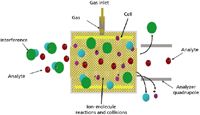
Figure 2: A representation of how cell-based ICP-MS works.

Collision-Mode ICP-MS

In collision mode, ions enter a cell filled with a nonreactive gas, typically helium. All ions collide with He molecules, but the larger ions undergo more collisions. With each collision, the ions lose some kinetic energy, so larger ions will lose more kinetic energy than smaller ions. At the exit of the cell, an energy barrier is established so that ions with only a certain amount of kinetic energy will be able to exit the cell and enter the analyzer quadrupole. This process is illustrated in Figure 3. .

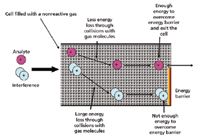
Figure 3: A representation of collision mode in a cell-based ICP-MS system.

So, how do these collisions remove the effects of interferences? Polyatomic ions are bigger than molecular ions: ArCl⁺ consisists of two components, while As⁺ is only a single molecule. As a result, polyatomic ions undergo more collisions with the gas than molecular ions. Because each collision results in energy loss, polyatomic ions lose more kinetic energy than molecular ions. Therefore, polyatomic ions cannot exit the cell because they do not have enough energy to overcome the energy barrier, while molecular ions have enough energy to exit the cell. As a result, the effects of polyatomic interferences are removed.

Collision mode is a simple and effective way to remove polyatomic interferences and, because of the mechanism of interference removal, one set of conditions can be applied to many elements in many matrices. This offers a clear advantage over correction equations and matrix removal: a single set of conditions is applicable to multiple analytes and matrices. Of course, conditions can also be tweaked to improve performance for specific elements and matrices.

Although simple and effective, collision mode also has its limitations. Because interferences are removed based upon differences in atomic size, collision mode cannot remove isobaric or doubly charged interferences. Fortunately, it is usually possible to choose an isotope which does not have an isobaric interference, and doubly charged interferences are usually not problematic.

Another limitation of collision mode is that significant analyte sensitivity is lost. This loss results from some analyte molecules losing so much energy during collisions in the cell that they cannot overcome the energy barrier. So, although the signals from polyatomic interferences can be almost completely eliminated, enough analyte sensitivity is lost to make ultratrace-level quantitative analysis difficult.

Despite these drawbacks, collision mode is a very effective technique for analyzing samples that have widely varying matrices, such as in environmental applications.

Reaction-Mode ICP-MS

In reaction-mode ICP-MS, the cell is filled with a reactive gas that is chosen so that it reacts faster with the interference than the analyte. Also, the voltages at both the cell entrance and exit are equal, which has two important consequences:

• All ions in the cell have the same energy. This means that the chemistry follows the laws of kinetics and thermodynamics, so that it is predictable, repeatable, and transferrable.

• There is no energy barrier at the exit of the cell, which means that the only loss of analyte sensitivity results from interactions with the gas.

The functionality of reaction-mode ICP-MS is depicted in Figure 4.

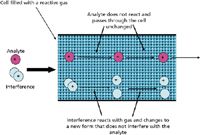
Figure 4: A representation of reaction mode in a cell-based ICP-MS system.

Another important characteristic of reaction-mode ICP-MS is the ability to control the chemistry in the cell. This is easily accomplished in quadrupole cells by applying a bandpass, which is essentially an electronic low-mass filter that ejects ions below a certain mass from the cell before they have a chance to react.

Because the chemistry in the cell can be tailored to the elements and interferences of interest, it is possible to eliminate the effects of interferences and measure as low as possible, primarily limited only by contamination in the laboratory environment and reagents. The use of chemistry not only removes polyatomic interferences but is also capable of removing the effect of isobaric and doubly charged interferences.

However, the chemisty also poses a limitation to reaction mode: one set of conditions cannot be applied to samples of widely varying matrix composition. Different matrices can require different cell conditions for maximum interference removal. Reaction mode is best suited for those applications where the matrix is relatively constant within a batch of samples, such as in semiconductor and clinical analyses.

Another possibility in reaction mode is to take advantage of chemistry to move elements of interest away from the interference, instead of removing the interference. This aspect is advantageous when the interference is not very reactive. An example of this application is the ⁴⁰ Ca³⁵ Cl⁺ interference on As⁺ at m/z 75. Because of the very strong bond between Ca and Cl, CaCl⁺ is unreactive with most gases. However, it is known that As⁺ reacts rapidly with oxygen to form AsO⁺ :

As⁺ + O₂ → AsO⁺ + O Rate Constant ≈ 10⁹

Therefore, we can take advantage of this chemisty to analyze As as AsO⁺ at m/z 91, thereby being free of the CaC^l+ interference at m/z 75.

Summary

Because of its ability to measure low levels rapidly, ICP-MS has grown quickly as an analytical technique. However, the issue of interferences has always plagued the technique, especially as technology improvements have allowed lower and lower levels to be measured. The effects of interferences have been removed both through mathematical means and new developments in instrument technology. The current state-of-the-art ICP-MS instruments can remove interferences effectively, which allows quantitative measurements to levels that are limited by the cleanliness of the laboratory environment, reagents, and water.

Kenneth Neubauer is with Perkin-Elmer Life and Analytical Sciences, Shelton, Connecticut.