Resonance Enhancement of Raman Spectroscopy: Friend or Foe?

The presence of electronic transitions in the visible part of the spectrum can provide enormous enhancement of the Raman signals, if these electronic states are not luminescent. In some cases, the signals can increase by as much as six orders of magnitude. How much of an enhancement is possible depends on several factors, such as the width of the excited state, the proximity of the laser to that state, and the enhancement mechanism. The good part of this phenomenon is the increased sensitivity, but the downside is the nonlinearity of the signal, making it difficult to exploit for analytical purposes. Several systems exhibiting enhancement, such as carotenoids and hemeproteins, are discussed here.

The physical basis for the Raman effect is the vibrational modulation of the electronic polarizability. In a given molecule, the electronic distribution is determined by the atoms of the molecule and the electrons that bind them together. When the molecule is exposed to electromagnetic radiation in the visible part of the spectrum (in our case, the laser photons), its electronic distribution will respond to the electric field of the photons. Then, as the atoms move during a vibration, the electronic polarizability will be modulated. This modulation of the electronic polarizability is what gives rise to the Stokes and anti-Stokes Raman spectrum.

If you think about it, electrons that are more loosely bound will be more easily modulated. So, because π electrons are more loosely bound than σ electrons, the polarizability of any unsaturated chemical functional group will be larger than that of a chemically saturated group. Figure 1 shows the spectra of stearic acid (18:0) and oleic acid (18:1). These two free fatty acids are both constructed from a chain of 18 carbon atoms, in one case fully saturated and, in the other, with one carbon double bond between the ninth and 10th carbon atom from the methyl end. In addition to this chemical difference, the saturated fatty acid is solid, whereas oleic acid is liquid. The liquid form accounts for the relative broader lines and some differences in the band ratios. However, the most important difference for this discussion is the presence of the analytical bands for the -C=C- stretch at 1655 cm^-1, and the CH stretch on the –C=C- bond at 3009 cm^-1. Assuming the signal strengths reflect the number of functional groups, one would expect the –C=C- band to be weaker than the C-C bands. In fact, the integrated intensity between 1600 and 1700 cm^-1 (C=C) is twice as strong as the integrated intensity between 1030 and 1140 cm^-1 (C-C) even though there are 16 single bonds versus 1 double bond! The spectrum of an unsaturated fatty acid is dominated by the -C=C- stretch.

Figure 1: Raman spectra of stearic acid (red) and oleic acid (blue).

These compounds are still optically clear. There is no electronic transition in the visible part of the spectrum where the Raman effect is being excited and transmitted. When there are such transitions things become much more complicated. Aside from needing to worry about the sample self-absorption (similar to the issue in the near infrared [NIR]), there is the enhancement issue that we want to explore in this column.

Resonant Raman Scattering

The previous example illustrates how the presence of π electrons can increase the polarizability of a molecule, thus increasing the Raman cross-section. But this does not yet represent resonant conditions. The resonance condition for Raman scattering occurs when there is a transition in the vicinity of the energy of the laser photon. Because most work, especially analytical work, is done with visible lasers, this means a visible transition, which will be an electronic transition because typical visible photon energies (1.5–3 eV) are in the range of electronic transitions. For the purposes of this article we will discuss molecular systems with π electrons, although there are certainly other classes of electronic transitions in the visible part of the spectrum.

For unsaturated molecular systems to produce visible optical transitions, the π electrons must be "conjugated," which means the molecule must contain multiple carbon double bonds separated by single bonds. These molecules can be linear (called polyenes) or they can be represented by closed rings that are called "aromatic," certainly because of the characteristics of some of the earliest known examples such as benzene. Toward the end of the 19th century and into the beginning of the 20th century, there was development of synthetic textile dyes based on conjugated molecules, often containing N atoms; this industry actually represents the beginnings of organic chemistry. Very often the excited electronic states of dye molecules are long-lived and result in decay via radiative emission, which is known as fluorescence and phosphorescence. Fluorescence tends to be an intense process (quantum yields of the order of 0.5). Because Raman quantum yields are of the order of 10^-6, any molecule that fluoresces will probably not be a good candidate for Raman measurements; the Raman signal will be overwhelmed by its "fluorescence background."

Examples that exhibit beautiful resonance-enhanced spectra are taken from biomolecules — carotenoids and hemes. They illustrate the sensitivity of the technique, as well as the information that can be derived. They also illustrate that the symmetry of the molecule as well as the electronic and vibrational states determine the mode of enhancement and the types of vibrations that are enhanced.

Carotenoids

Carotenoids are chains of alternating double and single bonds. β-Carotene, the orange pigment in carrot root, is the quintessential carotenoid, although there are many others, such as lycopene, the red pigment in tomatoes and red peppers. The visual pigments are also carotenoids. In fact, retinal can be conceptualized as β-carotene cleaved in half and then terminated with an aldehyde group. Figure 2 illustrates the structures of β-carotene and retinal.

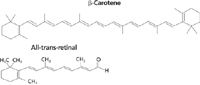
Figure 2: Structures of β-carotene and all-trans retinal.

An isolated double bond absorbs at about 200 nm (~6 eV). As double bonds are added to a conjugated chain, the energy of the lowest energy absorption occurs at decreasing energies (or increasing wavelengths). The free electron model has been used to describe these states: The electrons are approximated as particles in a box defined by the length of the chain (1). As the box becomes longer, the energy of the states become lower, with an asymptotic long wavelength value of about 600 nm.

There is an analogous phenomenon in describing the vibrational states (2). An isolated double bond has a frequency of about 1650 cm^-1 and a single bond has a frequency near 1000 cm^-1. But in a conjugated system the double bond frequency is lowered, and the single bond frequency is raised (because of the averaging of the bond order). As the chain is lengthened, these shifts will increase until the frequencies hit "limiting" values of about 1495 and 1150 cm^-1.

All of this means that there is a correlation between the conjugation length, the optical absorption wavelength, and the Raman vibrational frequencies. However, there is actually more than a correlation. The presence of the optical absorption enables the resonance Raman phenomenon, and the resonance is quite sensitive to the excitation wavelength; that is, the important parameter in the resonance phenomenon is the proximity of the laser wavelength to the absorption maximum. So, if you are able to tune the laser wavelength, you will see a variation in the resonance enhancement that follows the visible absorption spectrum.

The resonance enhancement of the Raman signals of polyenes shows up in surprising circumstances. The first example that comes to mind is that of degraded polyvinyl chloride (PVC) (3). It turns out that during the degradation of PVC there is an extraction of H and Cl across one of the polymer backbone bonds resulting in a π bond. Interestingly, the extraction tends to occur on adjacent bonds so that one ends up with a segment of alternating single and double bonds. The result is that degraded PVC has polyenes with a population of sequence lengths. If you examine such materials visibly they may be tinged orange or there may be no visible coloration. But if you record a Raman spectrum the polyene can be observed with very high sensitivity, even when there is no evidence for its presence optically. What is additionally interesting is that the observed Raman frequency depends on the excitation wavelength because each wavelength excites a different part of the population with its own resonance values.

The second surprising instance where I observed a carotenoid occurred while I was working with Professor Gene Hall of Rutgers University. Professor Hall was studying a series of fish oil supplements. When he examined a krill oil, he found that the spectrum was overwhelmed by the spectrum of a carotenoid, even though the sample was colorless. A more careful examination of the spectrum, while referring to the Merlin publication that we cited earlier (2), indicated the presence of astaxanthin, a well known natural carotenoid that differs from β-carotene in that there are carbonyl and OH functionalities on each of the ring end groups.

It is useful to review briefly the mechanism by which the spectra of polyenes is enhanced. The π to π* electronic transition is an "allowed" transition that is polarized along the axis of the molecule, so vibrations that couple to it most effectively have symmetric character. But not all vibrations will be resonance enhanced. A vibration will be resonance Raman active if there is a change in bond length or angle in the excited electronic state compared to the electronic ground state (Franck Condon effect). In fact, because the π to π* excitation has the effect of shortening the single bonds and lengthening the double bonds, it is the stretching vibrations of the carbon-carbon bonds in the chain that will have the greatest resonance Raman intensity (4).

This becomes interesting in the study of the biological polyenes because there are important biological processes that depend on isomerization (4). There are two light-sensing systems that function because of isomerization. In vertebrate eyes, the pigment for black and white vision has an 11-cis retinal chromophore bound to lysine on a particular protein by a protonated Schiff base bond. In photosynthetic bacteria there is a bacteriorhodopsin containing an all-trans retinal protonated Schiff base. During the visual process, the vertebrate rhodopsin undergoes a series of bleaching intermediates until the chromophore is separated from the protein in the all-trans isomer. In photosynthetic bacteria light absorption results in conversion to the 13-cis isomer, driving the translocation of protons across the cell membrane, and then reisomerization back to the light-adapted form (4). Isomerization can be followed in the resonance Raman spectra because isomerization changes the conjugation of the π electrons, which will shift the electronic absorption maximum and the resonance Raman observed frequencies. What is even more interesting is that dynamic Raman and hole burning absorption studies (on the picosecond time scale!) have been used to follow cis–trans photoisomerization (5).

Hemeproteins

Hemeproteins form another class of biomolecule that experiences a strong resonance enhancement. The heme, which is the part that is interacting strongly with visible light, is an iron complex of porphyrin. The porphyrin molecule is classified as an aromatic molecule even though it is not based on aromatic six-membered rings. Porphine, on which porphyrin is based, is a tetrapyrrole shown in Figure 3. Molecular orbital theory argues that the inner ring of 16 atoms has 14 π electrons plus four out-of-plane N electrons, with the outer π electrons being essentially ethylenic (6).

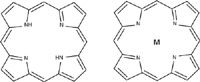
Figure 3: Structure of porphine (left) and porphine complexed with a metal as in heme or chlorophyll (right); note that metal complex with porphine has two tautomeric equivalent forms (second not shown) resulting in fourfold symmetry.

The electronic absorption spectrum of porphine consists of a strong band near 400 nm (termed the B or Soret band) and four weaker bands between 500 and 900 nm (the Q bands). In the metal complex, the four weaker bands in the visible part of the spectrum collapse into two bands. This has been interpreted to be evidence for twofold symmetry in the porphine, and fourfold symmetry in the metal–porphine complex. This spectral behavior has been explained in terms of a cyclic polyene model (6). Note that the hemes that occur in hemoglobin, myoglobin, and the cytochromes all contain side groups that, in principle, lower the symmetry of the porphine core. But the electronic spectra have still been successfully explained by the fourfold symmetry model (except for chlorophyll, the Mg complex of a substituted chlorin, in which the π system's symmetry is lowered by some saturation). The Q and Soret absorptions were respectively assigned to transitions between the two highest filled orbitals and the lowest empty orbital. Martin Gouterman then included the evolving techniques of molecular orbital theory to include configuration interactions to correct for the neglect of electron correlations. Using group theory he found that the excited B and Q states have the same symmetry, so they mixed. In addition, there can be vibronic mixing between the B and Q states, but because these states are already orthonormal, only asymmetric vibrations can mix the states. In this Hertzberg-Teller coupling, the Q band acquires intensity from the B or Soret band via vibronic mixing. And this same Hertzberg-Teller coupling accounts for the resonance in the Raman scattering (7). The predictions for the resonance Raman active vibrations have A_2g, B_1g, and B_2g symmetry in the D_4h point group used to describe the heme. Unlike A_1g vibrations, which are usually the most intense features in the spectrum, these asymmetric vibrations will not have high intensity in parallel polarized scattering and, in fact, the highest intensity was observed for the antisymmetric A_2g modes (7).

Some of the hemeproteins, especially reduced cytochrome c (cyt c⁺²) and to some extent oxyhemoglobin (HbO₂) have vibronic states in the Q band that are so long lived that the absorption spectra recorded close to the temperature of liquid nitrogen exhibit sharp bands, from which the lifetimes of the states can be calculated (8). In reference 8 the quantum yields of the Raman and the resonance fluorescence were measured from five hemeproteins and were found to be consistent with the lifetimes as determined by the optical spectra.

The optical spectra of hemes (the iron complexes of porphyrin) depend on the oxidation and spin state of the iron, the protein environment (including the axial ligands), and the side groups on the porphyrin. Many hemeproteins (including hemoglobin and myoglobin) incorporate protoheme in which two of the side groups are vinyl (C=C) groups. The π electrons of the vinyl groups are conjugated to the porphine ring, which causes a red shift in the absorption spectra. Mitochondria, the energy-releasing organelles in cells, contain a series of cytochromes that includes "b-" and "c-" types. The b-type cytochromes are also based on protoheme, but the c-type cytochrome is covalently attached to its protein via a reaction of the vinyl sidegroups with the protein resulting in a loss of their π electron character. Because the absorption spectra of the c-type cytochromes are blue-shifted relative to that of the b-type cytochromes, the enhancement of their vibrational spectra will be different. In my previous life I measured the resonance Raman spectra of the b-c₁ complex (also known as cytochrome c reductase) solubilized in a detergent solution and in whole mitochrondria (9). These mitochrondrial membranes contain one c-type heme and two b-type hemes. The goal was to identify spectral features of the different heme types and then follow them as a function of redox potential, a parameter controlling the oxidation-reduction state of the hemes (which would be determined by the membrane structure) and the controlled capture of energy that enables the production of adenosine triphosphate (ATP). We determined that the b-type hemes have extra resonance Raman bands resulting from the extra π electrons on the vinyl side groups, which provided a marker to differentiate the two cytochrome types. For instance, the 1315 cm^-1 inverse polarized band in cytochrome c is replaced by two bands near 1305 and 1340 cm^-1 in cytochrome b. Figure 4 shows the resonance Raman spectra of the b-c₁ complex excited at 568.2, 530.9, and 520.8 nm (lines of a krypton laser). Table I lists the marker lines that appear in the spectra.

Figure 4: Resonance Raman spectra of cytochrome c reductase (cytochrome b-c1 complex) in the reduced state, excited by 520.8, 530.9, and 568.2 nm at a concentration of 66 µM cytochrome c1. These spectra were recorded on a single channel detector on a scanning double monochromator using about 350–400 mw of laser power and scanning at a rate of 50 cm-1/min. This figure has been adapted with permission from reference 9.

Because of the red shift in the electronic spectrum for the b-type cytochrome, the 568 nm line only excites those cytochromes. However, the other lines excite both types. We did make measurements of this preparation as a function of redox potential to follow the disappearance of the various species (10). What we expected to see was the bands of each species disappearing separately as that species became oxidized (the resonance Raman spectrum is much stronger for the reduced rather than the oxidized species). What we found was that the spectra seemed to disappear in parallel, as if the hemes are all coupled together on the membrane!

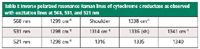
Table I: Inverse polarized resonance Raman lines of cytochrome c reductase as observed with excitation lines at 568, 531, and 521 nm

Downside

Ok, so what is the downside of making resonance Raman measurements? With these examples, we have discussed the extremely high sensitivity and shown how powerful the information can be in characterizing samples. What we have not discussed is the fact that the intensity of the measurement is not linear with concentration. The samples are colored; the source of the coloration, in fact, is where the resonance is coming from. But that color will cause self-absorption, reducing the signal, and that reduction will change with excitation wavelength, and will also change over the range of the resonance Raman spectrum at a given excitation wavelength. I remember one of the last things that I did was to measure a sample of reductase at 10 µM. I had previously standardized my measurements at 100 µM and then was surprised to find that a lower concentration gave me a stronger signal. For sure, this is because at 100 µM the sample had a definite pink tinge to it and was absorbing the green light. In principle, one can calculate what this absorption would be, but the calculation would require knowing the pathlength into the sample, and then back out, as well as all the other usual parameters. This means that using these measurements for analytical purposes in which concentration information is the goal may not be realistic.

Summary

In this installment of "Molecular Spectroscopy Workbench," we have considered the phenomenon of resonance enhancement of Raman signals. We have attempted to introduce the origin of the phenomenon with a few examples taken from biomolecules that indicate the potential value of the information available, but we then explained that because of the electronic absorption that provides the enhancement mechanism, self absorption of the sample will make extraction of concentration information problematic.

Please note that in citing publications, it is difficult for me to make a thorough search of the literature. For the work on carotenoids, I have cited articles that are available and known to me. My apologies to anyone whose work was not cited. In describing the work on hemes, my goal was not to describe my own work (which was done more than 35 years ago). I used the example of the hemes because I know it well, and it really is a spectacular example of resonance Raman.

References

(1) M. Orchin and H.H. Jaffe, Symmetry, Orbitals, and Spectra (Wiley Interscience, New York, 1971).

(2) J.C. Merlin, Pure & Appl. Chem. 57(5), 785–592 (1985).

(3) D.L. Gerrard and W.F. Maddams, Macromolecules 14, 1356–1362 (1981).

(4) B. Curry, I. Palings, A.D. Broek, J.A. Pardoen, J. Lugtenburg, and R. Mathies, in Advances in Infrared and Raman Spectroscopy Vol.12, R.J.H. Clark and R.E. Hester, Eds. (Wiley, New York, 1985), Chapter 3.

(5) W.T. Pollard, C.H. Brito Cruz, C.V. Shank, and R.A. Mathies, J. Chem. Phys. 90(1), 199–208 (1988).

(6) F. Adar, in The Porphyrins, Vol. III, D. Dolphin, Ed. (Academic Press, Waltham, Massachusetts, 1978).

(7) T.G. Spiro and T.C. Strekas, Proc. Nat. Acad. Sci. 69(9), 2622–2626 (1972).

(8) F. Adar, M. Gouterman, and S. Aronowitz, J. Phys. Chem. 80(20), 2184–2191 (1976).

(9) F. Adar and M. Erecinska, Biochemisty 17(25), 5484–5488 (1978).

(10) F. Adar and M. Erecinska, Febs. Lett. 80(1), 195–200 (1977).

Fran Adar is the Worldwide Raman Applications Manager for Horiba Jobin Yvon (Edison, New Jersey). She can be reached by e-mail at fran.adar@horiba.com

Fran Adar