Raman Spectra of Metal Oxides
Metal oxides often occur in crystals where there are no molecular units. Here, we provide an introduction to the concepts that need to be understood when analyzing metal oxides in materials such as paint, ceramic pigments, corrosion films, catalysts, and minerals.

Metal oxides often occur in crystals where there are no molecular units. In contrast to Raman analysis of organic compounds in which there are molecular vibrating units with functional subunits, many of whose vibrations are isolated from the remainder of the molecule, the analysis of spectra of oxides requires understanding how atoms move in crystal lattices. These oxides occur in diverse materials such as paint and ceramic pigments, corrosion films, catalysts, and minerals. This column installment attempts to introduce analysts who often work with organic materials to the concepts that need to be understood in analyzing these materials.
The distinction between the vibrational analysis of an organic molecule, which can occur in a crystalline lattice, and that of a metal oxide, which normally occurs only in a crystalline lattice without a clearly defined molecular functional group, is an important one. The analysis of atomic motion is described by a field called lattice dynamics; the unit cell of the crystalline form is defined and the motion of each atom in the unit cell is determined by solving the potential energy function of the lattice, which is the sum of all pairwise potential energies between each pair of atoms. To solve the equation, the sum is performed only over neighboring atoms (that is, in the unit cell) because the electric fields of distant atoms are assumed to be screened. In addition, the potentials are assumed to be harmonic, which will be true if the motion is small compared to the interatomic spacings. Another assumption is that the electronic motion can be separated from the atomic motion because, being much less massive, the electrons move much faster than the atoms. This is called the adiabatic or Born-Oppenheimer approximation.
An important concept in the description of the motion of atoms in crystals (phonons) is the symmetry of the lattice. Group theory enables one to classify a crystal lattice according to what geometrical operations in space leave the lattice unchanged; if there is hexagonal symmetry, for example, and the lattice is rotated by 60°, the lattice will not have been changed. When the phonons are worked out, they will have symmetries consistent with the lattice symmetry. If a phonon leaves the lattice symmetry unchanged, it is described as being symmetric. However, a phonon can distort the lattice in a way that is consistent with its character; this type of phonon can be described by one of the "irreducible representations" of the point group of the lattice. Clearly, this short column cannot cover lattice dynamics, but if readers are sufficiently interested they can take a look at numerous texts that are available (1,2). In addition, two of David Tuschel's recent installments of "Molecular Spectroscopy Workbench" considered some of these concepts in more detail (3,4).
For analysts who need information on this class of materials, I can recommend a text by Ross (5) that may be difficult to locate, and a more recent text from the European Mineralogical Union (6). There are also emerging resources on the web such as the RRUFF data base (7) and the Romanian Data Base of Raman Spectroscopy (8).
Before going on, I want to add some remarks on amorphous oxides and "soft modes." Metal oxides do not always occur in a crystalline form. When in an amorphous state, the Raman bands are quite broad, but often can be derived from one of the crystalline forms of the same material. In fact, in the case of ceramics, the transition from the amorphous to crystalline form (devitrification) can be an important phenomenon in terms of the performance of the ceramic. The assumption is that in the amorphous form there is a distortion of interatomic bond angles. Long-range order is lost, but nearest-neighbor interactions are affected so as to shift a particular vibrational frequency; because there is a population of such distortions, the observed band will be broad.
There is one important case where atomic motion may not be small compared to the interatomic spacings. There are cases where there can be a (crystalline) phase transition in which the lattice changes symmetry. The most cited example is that of BaTiO3, which is cubic at any temperature above 393 K, but is tetragonal at lower temperatures. In the cubic phase, every atom sits on a center of symmetry, but in the tetragonal form the Ti atom in the center of the unit cell is displaced in one of the cubic directions and the octahedral cluster of O atoms are displaced in the opposite direction. It turns out that there is a normal mode that, if frozen, exactly induces this change. When one follows this mode as a function of temperature, its frequency is observed to go lower as the atoms in the lattice move toward their new coordinates. Figure 1, adapted from reference 9, shows the unit cell of BaTiO3 in the distorted tetragonal phase.
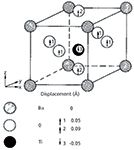
Figure 1: BaTiO3 in the distorted tetragonal phase. Adapted with permission from reference 9.
The Oxide Lattice
Metal oxides occur in crystals in which the O-2 anions are often in close-packed sites and the metal cations fit into tetrahedral or octahedral sites, with two tetrahedral sites per O-2 ion and one octahedral site per O-2. To a large extent, this follows from the fact that the ionic radii of anions are significantly larger than that of the cations. In fact, relatively simple geometric arguments based on ionic radii enable predicting whether a particular anion will occupy tetrahedral or octahedral sites (10). Of course, the stoichiometry of the oxide will determine how many metal cations will be present and details regarding the electronic states will play a role determining the overall symmetry of the lattice.
But the structure for a particular oxide is often not unique. A common example is TiO2, a white pigment that is found in paper, polymers, and paints, and as photoreactive coatings on glass. TiO2 occurs in two common forms known as anatase and rutile, as well as a form known as brookite that sometimes occurs in minerals. As shown in Figure 2, the Raman spectra of these forms are quite different from each other. Aside from the limited intensity in the low 140 cm-1 region in the spectrum of rutile that overlaps with the strong band in anatase, these spectra would not be suspected as originating from the same chemical entities.
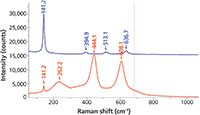
Figure 2: Raman spectra of crystalline anatase (top) and rutile (bottom), two forms of TiO2.
Another metal oxide that has multiple forms is ZrO2. The high temperature phase (>1500 °C) is cubic and the first order phonons are not strictly Raman active because every atom sits on a symmetry site, although broad bands do often appear. The normal room temperature phase is monoclinic, but the ceramics engineer might want to create a powder or compacted part in the tetragonal phase (that normally exists at a temperature above room temperature but lower than that of the cubic phase) by alloying with a small amount of CaO or Y2O3. Figure 3 shows the spectra of the tetragonal and monoclinic phases of ZrO2.
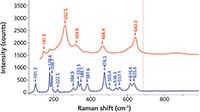
Figure 3: Raman spectra of the tetragonal (top) and monoclinic (bottom) phases of ZrO2.
Some years ago Professor William Wright of Penn State University described to me the phenomenon of topotaxy (11), which could be quite interesting, although I have never observed it. In this case, in a system like that of ZrO2 or TiO2, which both have two or more crystal phases, it is possible for the two phases to exist simultaneously in a continuous "crystal." In this case, there are specific crystal planes where the atomic placements are the same for the two phases, and this is what enables one phase to "grow" out of the other.
Another phenomenon that is sometimes observed in oxide powders is the effect of particle size. There is often an interest in engineering materials of particular dimensions, especially with the current activities in nanotechnology. When the dimensions of crystals get small enough, the phonons experience "quantum confinement." Because the crystals are so small, the normal assumption of translational symmetry does not apply. When this happens, the phonon wavevector is no longer constrained at 0; that is, there is uncertainty in the value of the scattering wavevector. Raman scattering has contributions away from the center of the Brillouin zone.
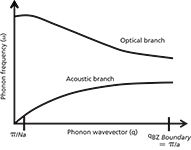
Figure 4: Hypothetical dispersion of acoustic and optic phonons in a lattice with two atoms in the unit cell (for example, silicon).
The concept of the wavevector has not yet been introduced in this column. The phonon wavevector describes how the vibration propagates through the crystal. As a result of translational symmetry, there has to be wavevector conservation. That means that the difference between the wavevectors of the incident and scattered light in the crystal has to be transferred to the phonon. For crystals larger than 1 μm, this difference will always be much smaller than the dimension of the Brillouin zone, which scales as the reciprocal of the lattice constant. That is why you may hear it said that Raman scattering in crystals always occurs at the Brillouin zone center. When the crystal becomes much smaller than 1 μm, there is uncertainty in the phonon wavevector because the mean free path of the phonon is larger than the nanocrystal's dimensions. So, there will be contributions to the Raman scattering from phonons away from the center of the Brillouin zone. In most crystalline systems, the phonon energy decreases with the phonon wavevector so one tends to observe the Raman frequency decrease in nanocrystals; the band tends to be broadened and asymmetrical, with a low frequency tail because one is observing contributions from particles of varying sizes. This phenomenon has often been observed in crystal silicon that had been annealed from the polycrystalline state (12,13).
Figure 5 shows spectra of elemental silicon in various stages of crystalline order. Although this material is not an oxide, the spectra illustrate the phenomena that we have been discussing. The bottom spectrum is that of amorphous silicon, the top of crystalline material. The strong band assigned to the optical phonon in the crystal at 520 cm-1 is broadened and down-shifted in the amorphous material. The amorphous phase also shows a broad band centered near 150 cm-1, which can be assigned to scattering by phonons that are not restricted by symmetry arguments of the crystal because there is no translational symmetry.
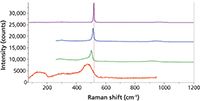
Figure 5: Raman spectra of crystalline (top) and amorphous (bottom) silicon as well as two samples with the crystalline silicon peak down-shifting because of particle size (middle two spectra), with evidence for a mixture with the amorphous phase in the spectrum second from the bottom.
One other curious thing can be said about observations in nanocrystals. It is possible in some instances for the optical phonon dispersion curve to curve up before it goes down, and this has been observed in anatase (14). In this case the phonon will be shifted up in frequency and broadened on the high frequency side.
To add to the usefulness of this article, I will discuss the oxides of iron along with some related phases. Iron is the metal element that is used most prevalently for mechanical structure. However, it easily forms a surface oxide and the most common surface oxides ("rust") do not stick to the metal. That means that the metal continues to oxidize, reducing the metal content continuously. For uses that can support additional cost, the metal is alloyed with additional metals, especially chromium, which has the benefit of "passivating" the surface. That means that a layer of Cr2O3 forms on the surface and sticks. Figure 6 shows the spectra of four common oxides of iron as well as Al2O3 and Cr2O3. Hematite, Fe2O3, is the common form of rust. When it is highly crystalline, the peak near 225 cm-1 sharpens and splits. Magnetite, Fe3O4, crystallizes in the spinel form, which can accommodate a range of metals in solid solution, especially transition metals; it is easily distinguishable from hematite, but its spectrum is generally weaker and the bands are broader and they will be shifted when it occurs as a solid solution. A third oxide, FeO, crystallizes in the rock salt structure in which every atom sits on a site of symmetry, and is thus Raman-inactive. Geothite and lepidocrocite are two forms of FeOOH, and they are easily distinguishable in their spectra. The spectrum of Cr2O3 was recorded from a powder from a bottle with no information on elemental composition. It is well known that metal oxides can form solid solutions, so the frequencies shown in this spectrum may not be accurate for pure Cr2O3. Note that Cr2O3 has a very strong photoluminescence doublet at 693 nm that is used as a strain calibrant. The spectrum of Al2O3 is shown because it, like Cr2O3, is isomorphic with Fe2O3. The ability to differentiate these species enables the use of Raman microscopy to aid in the elucidation of corrosion mechanisms. Also, because measurements can be made in situ, one can follow corrosion in water, brine, or at elevated temperatures.
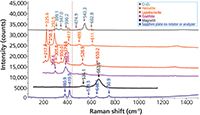
Figure 6: Raman spectra of the two common iron oxides, two common iron hydroxides, Cr2O3 and Al2O3, which are isomorphic to Fe2O3. From top to bottom they are Cr2O3, hematite, lepidocrocite, goethite, magnetite, and sapphire (Al2O3).
Metal Oxide Heterogeneous Catalysts
It is fairly well known that many heterogeneous catalysts are prepared by creating monolayers of defined transition (or other) metals on silica, alumina, ceria, zirconia, titania, or magnesia. Although there are numerous groups working in this field using Raman spectroscopy, in conjunction with other techniques, to elucidate the catalytic reaction, I am going to cite the work from Lehigh University because that is what I know best (15).
It is understood that a heterogeneous catalyst works by providing electronic sites on the surface that react with organic molecules that come into contact with these surfaces. In his review of the field in 2002, Wachs (15) described the surfaces as having monoxo, dioxo, and trioxo metal ions, and the geometry and electronic properties of these species will then determine the reactivity of the surface.
In that article (15), Wachs proposed that exchanging the oxygen on the surface with 18O would enable differentiating the possibilities. Mono species would show two Raman bands in isotopically substituted samples, dioxo species would show three bands, and so on. Thus, Raman spectroscopy has the ability to uniquely describe the surface configuration of a heterogeneous catalyst! However, to do this the catalytic surface has to be controlled by controlling the temperature and chemical state of the surface in a chemical reactor. Bañares and Wachs (15) argued that the catalytic performance can be understood by controlling the temperature and chemistry of the environment while making measurements (Raman, infrared, gas chromatography, and so on). In particular, molecular level information regarding the surface metal oxides are revealed by Raman spectroscopy. In this article, Bañares and Wachs systematically studied the behavior of numerous catalysts as a function of temperature cycling, reduction, and hydration to put together a story about the structure and reactivity of the catalytic surfaces.
In contrast to a Raman study of a bulk crystalline or amorphous form of a metal oxide in which spectral bands correspond to atomic motions representing long or mid-range order in the solid, in the case of catalysts, the Raman spectrum represents the structure of a surface. That means that without nanometer spatial resolution one can infer the geometrical or chemical properties of atoms at a surface by interpreting the Raman spectrum! With this information one can understand the reactivity of a surface, and then engineer a catalytic surface to perform a desired task. This takes catalyst engineering from empirical-try-everything to rational design.
Summary
We have reviewed some of the major characteristics of Raman scattering in bulk oxides (crystalline, and to some extent amorphous) and in heterogeneous catalytic materials. Depending on the application, the spectrum can provide identification of pigment, elucidation of a corrosion process or surface oxide treatment, characterization of amorphous or nanocrystalline phases, and characterization of heterogeneous catalysts in which the active phase is a properly prepared surface metal oxide.
References
(1) A.A. Marududin, E.W. Montroll, and G.H. Weiss, "Theory of Lattice Dynamics in the Harmonic Approximation," Solid State Physics, supplement 3 (Academic Press, 1963).
(2) B. DiBartolo and R.C. Powell, Crystal Symmetry, Lattice Vibrations, and Optical Spectroscopy of Solids, A Group Theoretical Approach (World Scientific Publishing Co. Ptc. Ltd., Singapore, 2014).
(3) D. Tuschel, Spectroscopy 29(2), 14–23 (2014).
(4) D. Tuschel, Spectroscopy 29(3), 14–22 (2014).
(5) S.D. Ross, Inorganic Infrared and Raman Spectra (McGraw Hill, London, 1972).
(6) Raman Spectroscopy Applied to Earth Sciences and Cultural Heritage, J. Dubessy, M.C. Caumon, and F. Rull, Eds., Vol 12 of the European Mineralogical Union Notes in Mineralogy (European Mineralogical Union and the Mineralogical Society of Great Brittain and Ireland, London, 2012).
(7) http://rruff.info/.
(8) http://rdrs.uaic.ro/index.html.
(9) R.A. Cowley, Structural Phase Transitions - I.Landau Theory, Advances in Physics 29(1), 1–110 (1980).
(10) J.E. Huheey, Inorganic Chemistry, Principles of Structure and Reactivity, (Harper and Row, New York, 1972).
(11) Professor William Wright, private communication.
(12) G. Kanellis, J.F. Morhange, and M. Balkanski, Phys. Rev. B 21(4), 1543–1548 (1980).
(13) J. Richter, Z.P. Wang, and L. Ley, Solid State Commun. 39, 625 (1981).
(14) S. Mamedov, T. Moriyama, T. Numata, N. Nabatova-Gabain, S. Yanagida, and L. Yan, Mater. Res. Soc. Symp. Proc. 1578, http://dx.doi.org/10.1557/opl.2013.915 (2013).
(15) M.A. Bañares and I.E. Wachs, J. Raman Spectrosc. 33, 359–380 (2002).
Correction
The original, published version of this article contained an error in this paragraph: Another metal oxide that has multiple forms is ZrO2. The normal stable room temperature phase is cubic, which is not Raman active because every atom sits on a symmetry site. However, for chemical or physical reasons a ceramics engineer might want to create a powder or compacted part with one of the other two phases. ZrO2 can be stabilized in the tetragonal or monoclinic phases by alloying with a small amount of CaO or Y2O3.
The text has been corrected to read: Another metal oxide that has multiple forms is ZrO2. The high temperature phase (>1500 °C) is cubic and the first order phonons are not strictly Raman active because every atom sits on a symmetry site, although broad bands do often appear. The normal room temperature phase is monoclinic, but the ceramics engineer might want to create a powder or compacted part in the tetragonal phase (that normally exists at a temperature above room temperature but lower than that of the cubic phase) by alloying with a small amount of CaO or Y2O3. Figure 3 shows the spectra of the tetragonal and monoclinic phases of ZrO2.
Fran Adar is the Principal Raman Applications Scientist for Horiba Scientific in Edison, New Jersey. She can be reached by e-mail at fran.adar@horiba.com

Fran Adar
AI-Powered SERS Spectroscopy Breakthrough Boosts Safety of Medicinal Food Products
April 16th 2025A new deep learning-enhanced spectroscopic platform—SERSome—developed by researchers in China and Finland, identifies medicinal and edible homologs (MEHs) with 98% accuracy. This innovation could revolutionize safety and quality control in the growing MEH market.
New Raman Spectroscopy Method Enhances Real-Time Monitoring Across Fermentation Processes
April 15th 2025Researchers at Delft University of Technology have developed a novel method using single compound spectra to enhance the transferability and accuracy of Raman spectroscopy models for real-time fermentation monitoring.
Nanometer-Scale Studies Using Tip Enhanced Raman Spectroscopy
February 8th 2013Volker Deckert, the winner of the 2013 Charles Mann Award, is advancing the use of tip enhanced Raman spectroscopy (TERS) to push the lateral resolution of vibrational spectroscopy well below the Abbe limit, to achieve single-molecule sensitivity. Because the tip can be moved with sub-nanometer precision, structural information with unmatched spatial resolution can be achieved without the need of specific labels.
