Spectrometers for Elemental Spectrochemical Analysis, Part I: The Basic Spectrometer

A spectrometer consists of four basic modules: an excitation source, a dispersing element, a detector, and a read-out system.

Sir Isaac Newton (1642–1727) showed that the white light from the sun could be dispersed ("spread out") into a continuous series of colors. His apparatus was essentially the first spectroscope, consisting of an aperture or opening to define a light beam, a lens, a prism, and a screen (1). In this simplest of spectrometers, the sun provides the excitation source, the prism is the dispersing element, and the eye is the detector of the spectrum on the screen. In this case the "readout" is provided by the brain.

Figure 1

In emission spectroscopy, the excitation source excites the electrons of the sample atoms. In optical emission, the electrons transit to higher energy states and emit light on the return to lower energy levels. In X-ray fluorescence, an inner shell electron is ejected, an outer shell electron "drops down" to occupy this vacancy, and an X-ray is emitted. The dispersing system "spreads out" the light given off at various wavelengths. The detector measures the light intensity at the various wavelengths of interest. The read-out system provides a visual indication of the amount of light at these wavelengths.

The Excitation Source

There are many different ways to supply energy to a sample so that the atoms of the sample are induced to give off their characteristic radiation. The earliest method used was a simple flame. In 1826, the English inventor Talbot studied the color changes of flames when different salts were introduced. For example, the chlorides of lithium, sodium, and potassium produce red, orange, and lilac colors, respectively.

The German scientists Bunsen and Kirchhoff furthered the work of Talbot in the 1860s and were the first to realize that spectral lines are associated with elements and not molecules. Figure 2 (2) shows their spectroscope with a Bunsen burner providing the excitation. Note the other spectroscope modules: "D" is the Bunsen burner (excitation source), "E" shows a sample stand, "F" is the prism (dispersing element), and "C" is the viewer for detection and read-out.

Figure 2

Other excitation sources to be discussed in this series are arc/spark, X-ray excitation, the "hollow cathode lamp" used in atomic absorption, glow discharge, and the inductively coupled plasma. In this introductory article, we will focus on the various dispersing systems and detectors and then show how the modules are put together into a functioning spectrometer.

The Dispersing System

The first dispersing system was the simple prism. The prism functions by refraction, with the different light wavelengths "bending" by different amounts.

The grating was introduced in 1814 by Joseph von Frauenhofer for astronomical studies. In the X-ray region of the electromagnetic (EM) spectrum, the first dispersing element was a crystal.

We should note that sometimes the dispersing system and detector modules are one and the same as in the case of the semiconductor detectors used in energy dispersive X-ray fluorescence (EDXRF). Also, in optical emission–absorption spectrometers, the dispersing system plus detector often is called simply the "optical system" and sometimes the spectrometer, although this last is a misnomer as this term applies to the complete instrument.

The Grating

The grating is an optical component that disperses light. It is composed of many parallel, equally spaced slits or indentations (grooves). Just how many grooves the grating has is an important measure of its ability to disperse light. This quantity is measured in grooves per millimeter or grooves per inch (N).

The distance (d) between successive grooves is called the "grating constant," and is simply the reciprocal of N: that is, d = 1/N. Typical gratings used in modern optical emission spectrometers have 1800, 2400, or 3600 grooves/mm.

There are two basic types of gratings: The transmission grating, where the light goes through the slits of the grating, and the reflection grating, either plane or concave, where light is reflected from the grooved grating surface. The principle of operation is the same for both types.

The Simple Grating Equation

The grating works due to the interference of light waves. Consider the configuration shown in Figure 3, with two slits of a transmission grating, light entering from the left, parallel to the optic axis, and a screen some distance away to the right. The light waves impinging on the small openings spread out in circular wavefronts (a phenomenon called "diffraction"). Now, for light from the two slits to reinforce each other (add) so that we see light on the screen, the distance Y must be some (integral) multiple of the wavelength of light. That is, Y = ± nλ, where λ is the light wavelength and n is an integer. If we assume the light waves are one wavelength apart, n = 1, then Y = ± λ.

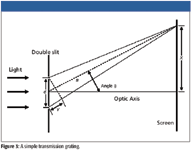
Figure 3

Notice that the triangle with sides X and B is similar to the triangle with the sides Y and d. Therefore, from the law of similar triangles (geometry), we have the relationship, X/B = Y/d. That is, side X is to side B as side Y is to side d. Because Y = λ and d = 1/N, we find by substitution that

where X is the distance of the image on the screen from the optic axis, B is approximately equal to the distance from the grating to the screen (given the dimensions involved), N is the number of grooves per mm on the grating, and λ is the wavelength of light. This is a very simple grating equation for the special case of light input perpendicular to the grating.

What does this grating equation tell us? First, that the distance X is dependent upon the wavelength of light. That is, the greater the wavelength, the greater the distance X from the optic axis, or the angle θ. Stated another way, different wavelengths of light will appear at different locations on the screen. Second, the greater the value of N, the number of grooves, the greater is the distance X or the angle θ. This means that the more grooves per millimeter the grating has, the greater the dispersion or separation of the light on the screen.

Using equation 1, we can calculate where a particular light wavelength (that is, spectral line) will appear on the screen, given the number of grooves per millimeter on the grating (N) and the distance of the grating (B). We can then place some sort of light-intensity measuring device (detector) at this location. The light measured then will be related to the element producing this particular spectral line at the particular light wavelength. The variable X is a measure of the dispersion or spread of the light wavelengths and "B" is related to the focal length of the optical system.

Note that the light which appears at location X in Figure 3 also will appear an equal distance (–X) below the center line (optic axis) in the drawing. Furthermore, if the light from the two slits had been separated by two light wavelengths (n = 2), there would appear another spot of light on the screen at a distance 2X from the center line or optic axis. This second "spot" is less bright than the first, however. This process continues for n = 3, 4, and so forth, with each succeeding light "spot" where the two waves from the slits interfere constructively becoming less intense. The integer n is referred to as the spectral order. In general, measurement of light in "first order" (n = 1) is preferable because it is more intense than succeeding spectral orders. Further details on the grating equation are included in Appendix I.

The Crystal

The dispersion of X-rays from crystals follows essentially the same law as that of light from a grating. This is to be expected since they are both electromagnetic waves.

In 1912, the German physicist Max von Laue (1879–1960) suggested using crystals to diffract X-rays. Later that same year, two German physicists, Walter Friedrich and Paul Knipping, acting on his suggestion, showed the diffraction of X-rays in zinc-blende (sphalerite).

The incident X-rays striking the atoms give reflected radiation that may be reinforced by the reflections from lower lattice planes (Figure 4). The requirement for positive reinforcement is that the extra path taken by the reflected beam from the lower plane of atoms (highlighted in red) must equal some integer number of wavelengths, just as in the case of the grating. That is, nλ = 2d sin θ, where θ is the angle of incidence of the X-ray beam, d is the distance between planes, and n is an integer number. This condition is known as Bragg's law. (Note: This condition is equivalent to the grating equation may be seen by setting φ = θ in equation 2 of Appendix I.)

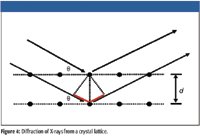
Figure 4

The Detector

The first detector for visible light radiation was the eye. This was followed by photographic film, which produced a permanent record of the spectrum. In the early 1940s, the photomultiplier tube was developed. With the discovery of the transistor in 1949 began the development of semiconductor detectors. The photodiode was the first, followed by the linear diode array (LDA) and various other solid-state devices.

The nature of the detector determines the terminology used in reference to the instrument:

• Spectroscope: Eye as detector

• Spectrograph: Photographic film as detector

• Spectrometer: Photomultiplier tube or semiconductor detector

The Photomultiplier Tube (Optical)

In the case of atomic emission and absorption instrumentation, photomultiplier tubes (PMT) have long been popular. A PMT is essentially a vacuum tube with a cathode and multiple anodes (called "dynodes"), as shown in Figure 5. A power supply of approximately 1000 V is required. This is divided up among the dynodes in, for example, 100-V increments, depending upon the number of dynodes.

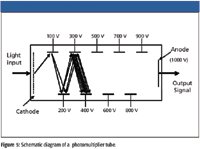
Figure 5

When a photon strikes the cathode, an electron is emitted. This process is called the photoelectric effect, first elucidated by Einstein in 1905. The negatively charged electron is accelerated to the first dynode, which is at a positive 100-V potential. Upon striking this dynode, additional electrons are ejected. These are then accelerated to the second dynode at a positive 200-V potential, emitting more electrons. These now impact the third dynode, at a positive 300 V, producing still more electrons. This process continues until the final dynode (also called the "anode" in this case) has collected a huge number of electrons resulting from the initial impinging photon. It should be clear why the PMT is also referred to as an "electron multiplier."

The spectral sensitivity of the PMT depends upon the coating material of the cathode and the window material. The appropriate sensitivity for the wavelength region of interest must be selected accordingly (see manufacturer's specifications for details).

There is a new generation of these detectors called "channel photomultipliers" (CPM) that promise both higher gain and lower noise.

Proportional Counter (X-Ray)

In X-ray fluorescence spectrometers, one of the first detectors was the proportional counter, one of the various types of ionization chambers, including the Geiger counter (3). Shown in the diagram of Figure 6, it consists of a gas-filled tube, which is the cathode (–), and a thin wire in the center making up the anode (+). A high-voltage source (~2000 V) is connected across the anode and cathode. Normally no current flows. However, when an incoming X-ray enters the tube, it produces ionization in the gas, allowing a current to flow through the circuit and producing an output signal.

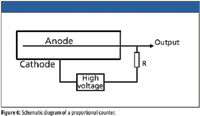
Figure 6

Solid-State Detectors (Optical)

Solid-state detectors have gained popularity in both optical emission and X-ray instruments in the past several decades. These are referred to collectively as charge-transfer devices (CTDs), which include such solid-state detectors as LDAs, charge coupled devices (CCDs), and charge injection devices (CIDs). These offer distinct advantages inasmuch as they can measure multiple parts of the spectrum at the same time, which allows the simultaneous measurement of multiple spectral lines of the analyte and permits real time background measurements.

The LDA (Figure 7) is essentially a chain of photodiodes. These devices are common in barcode readers, for example. The photodiode is a semiconductor diode that can detect light and convert it into an electrical voltage or current. One may think of a photodiode as a light-emitting diode (LED) in reverse.

Figure 7

When a photon of sufficient energy strikes the diode, it produces an electron-hole pair — that is, an electron and a positively charged electron hole. If a voltage is applied across the diode, then the electrons will move to the positive junction and the "hole" to the negative. This generates an electrical current which can be accurately measured.

The currently popular semiconductor detectors, CCDs and CIDs, are more sophisticated. They allow detection of light in a two-dimensional array, so the readout electronics are more complicated. One might think of them as a collection of coupled, side-by-side LDAs.

Solid-State Detectors (X-Ray)

In the X-ray region, the silicon PIN (positive–intrinsic–negative) and more recent silicon drift detectors (SDDs) obviate the need for a crystal-dispersing element (for certain applications) and provide complete spectral information. These are photodiodes sensitive to the X-ray region of the EM spectrum.

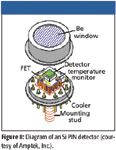
Figure 8

Figure 8 shows a pictorial diagram of this detector. (FET stands for field-effect transistor, a signal amplifier.) The schematic diagram of Figure 9 shows the internal workings. The large central "intrinsic" region is a charge-depleted silicon slab sandwiched between the P (positive anode) and N (negative cathode) layers of the diode.

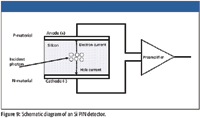
Figure 9

The incoming X-rays interact with the silicon atoms such that one electron-hole pair is produced for every 3.6 eV. A voltage is applied across the diode so that when an incoming X-ray produces ionization in the silicon region, a charge is immediately transferred.

The detector is thermoelectrically cooled to about –25 °C to decrease the leakage current and thus reduce noise. The lower background provided by the reduced noise enhances performance by enabling lower limits of detection.

The Readout System

The function of the read-out system is to take the electrical signal from the detector and provide an indication of the intensity of the spectral lines of interest. A schematic diagram of a computer-based readout system is shown in Figure 10. The signal from the detector is amplified and integrated (added) over the complete measurement time. The total is sent to an analog-to-digital converter, which provides a digital output that the computer can work with. The computer then compares this intensity signal to calibration data stored in memory to provide an output in the form of concentration of the elements present.

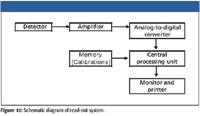
Figure 10

For X-ray systems using the crystal dispersing element (called wavelength dispersive X-ray fluorescence or WDXRF), the readout system is essentially the same as shown in Figure 10. For X-ray spectrometers using semiconductor detectors (EDXRF), the charge from the detector is converted to a voltage signal, which is proportional to the energy of the incoming X-ray. These voltages are then sorted by a multichannel analyzer (MCA) before being fed to the computer.

The readout for the spectrometer must be such that the counts (that is, number of photons collected within a given period of time) or the calculated concentration is displayed and stored along with the sample identification. Depending upon the actual instrument, it is possible to recalculate concentrations on-line if necessary (that is, the sample was analyzed using the incorrect analytical program), otherwise, off-line (manual) calculations might be required. The calculated concentrations are obtained by comparing the obtained signal (photon counts) with predetermined calibration curves.

Spectrometer Systems

How are these four spectrometer modules integrated to provide a complete spectrometer system? The EM radiation generated by the excitation source proceeds to the optical system (dispersing element plus detectors) and the detector signals are analyzed by the read-out system.

The optical system itself consists of three elements:

• light input from the excitation source,

• the dispersing element (grating, crystal), and

• the detector with output to the readout system.

The light path from the plasma generated by the optical emission or absorption excitation sources is either direct or by fiber-optic cable. The direct light path is simply a tube that generally is evacuated or filled with argon gas. At the end of the light path is the entrance slit. This is simply a thin (about 10–20 μm) vertical slit that makes a thin vertical line image of the light from the excitation source. The light from the entrance slit proceeds to the dispersing element.

The "light path" for X-rays is from the X-ray tube or radioisotope excitation source to the sample and then to the crystal or semiconductor detector as shown in the schematic of Figure 11.

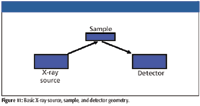
Figure 11

The Rowland Circle

One very common means of assembling the entrance slit, grating, and detector is through the use of what is called the Rowland circle, after the American physicist Henry A. Rowland who developed it in the late 19th century. This means that the entrance slit, grating, and detectors lie on the circumference of a circle, the "Rowland" circle (4).

(Historical note: Henry Rowland [1848–1901], a professor of physics at Johns Hopkins University from 1875 on, was the first to develop the [mechanical] means of ruling [inscribing the lines on] the concave grating. He also did experimental work resulting in accurate determinations of the value of the ohm and the mechanical equivalent of heat.)

The radius of curvature of the Rowland circle is one half of that of the concave grating so that everything is in focus at the circumference where the detectors are placed. That is, the images of the entrance slit are brought into focus somewhere on the circle and here we place detectors. This configuration is shown in Figure 12.

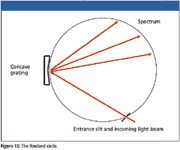
Figure 12

One correction must be made to the above in the case of PMTs. The detectors are not actually placed on the circumference of the Rowland circle. Their cathode-sensitive light-input entrances are too large and would receive the light input from many wavelengths simultaneously. Therefore, in front of the detectors, and on the Rowland circle are placed exit slits: generally 25–150 μm vertical slits, like the entrance slit, that block out most light wavelengths other than the one of interest. The various types of solid state detectors, with arrays of very-small-dimension semiconductor light-sensitive diodes that provide the simultaneous measurement of multiple spectral lines, do not require the use of these exit slits.

There are other optical systems (for example, the Littrow, Ebert, Wadsworth, and echelle configurations), but for many modern spectrometers, the Rowland design is probably the most common. This configuration often is called the "Paschen-Runge mount."

Polychromators Versus Monochromators

There are two very basic types of optical systems: The polychromator with many detectors set at fixed wavelengths, and the monochromator with one (or few) detectors set up in a manner such that many wavelengths may be scanned.

The polychromator is a simultaneous optical system with a fixed set of spectral lines. Such systems are designed for fast results on routine analytical tasks. This is the type of optical system used in the vast majority of spark spectrometers.

The monochromator is a sequential optical system, which allows scanning from wavelength to wavelength. This scanning of wavelengths is usually accomplished by moving the detector along the focal curve or by moving the grating. The monochromator provides greater flexibility for the chemist or operator, but at the expense of speed of analysis.

Optical emission spectrometers with one or more polychromators and a monochromator are currently available and so, in many ways, combine the best of both worlds: speed and flexibility. Such systems are particularly of interest for research laboratories and independent testing services, where a great variety of analytical tasks can be encountered.

The introduction of solid-state detectors, with their intrinsic ability of carrying out multiple wavelength detection has been gradually replacing the conventional combination of mono- and polychromators traditionally used with PMTs. This is accomplished by simply placing these CTDs at the end of the optical path, either as a pair of two-dimensional, or as a set of linear arrays, depending upon the optical arrangement, and providing simultaneous measurement of the whole spectra in integration times around 1 min.

Conclusions

The various modules of a spectrometer have been addressed:

• An excitation source provides energy to excite the atoms of the sample.

• Light-dispersing elements have been discussed.

• Various detectors for the emitted radiation have been covered.

• A simple model of the readout system has been presented.

• Finally, we have shown how these modules are connected into a complete spectrometer system.

Appendix I: The Grating Equation

Refer back to Figure 3. We will start with the relationship X/B = Y/d. For any right triangle, trigonometry defines the sine of the angle θ as the side opposite the angle (X) divided by the hypotenuse (B). That is, sin θ = X/B. Substituting this relation and the necessary condition for maximum light (Y = ± n λ) into the first equation above we find,

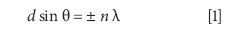
where the plus or minus simply means that the maxima (and minima) of light are symmetric about the optic axis. This is called the Grating Equation for the special case where the incoming light is at 90° to the grating.

The more general grating equation includes the sine of the angle of incidence of the light to the grating, with respect to the grating normal. The grating normal is a line drawn perpendicular to the surface of the grating. Since this is the form used in most all optical systems, it is presented below:

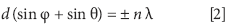
where φ is the angle of incidence of the light. Where this angle is zero, that is, the incoming light is perpendicular to the grating or parallel to the grating normal, we have sin(0) = 0. Then the general form of the grating equation reduces to that given by the first equation above.

Acknowledgment

The author would like to thank Carlos Coutinho for several helpful discussions.

Volker Thomsen is a consultant in spectrochemical analysis and lives in Atibaia, SP, Brazil. He can be reached at vbet1951@uol.com.br.

References

(1) V. Thomsen, Spectroscopy 21(10) 32–42 (2006).

(2) This illustration is from their 1860 paper in Annalen der Physik. It is available online at http://en.wikipedia.org/wiki/Gustav_Kirchhoff.

(3) G.F. Knoll, Radiation Detection and Measurement, 3rd Edition (Hoboken, New Jersey, John Wiley & Sons, 2000).

(4) V. Thomsen, Modern Spectrochemical Analysis of Metals: An Introduction for Users of Arc/Spark Instrumentation (ASM International, Materials Park, Ohio, 1996).