Quantitative Analysis of Crystal Violet by Raman Spectroscopy

This article describes the application of Raman spectroscopy to quantitatively measure the concentration of crystal violet using acetonitrile as the internal standard. Band intensity ratios of crystal violet to acetonitrile, that is I₁₁₇₉/I₂₂₆₀ and I₁₆₂₂/I₂₂₆₀, were linear with the concentration of crystal violet. The equations of the linear calibration curves were y = 0.4586x – 0.0115 with R² of 0.9926 for I₁₁₇₉/I₂₂₆₀ and y = 0.6431x – 0.01076 with R² of 0.9960 for I₁₆₂₂/I₂₂₆₀. The limit of detection was 4.62 × 10^-6 M.

Crystal violet is a dye belonging to the triphenylmethane family. It has been used to control fungi and intestinal parasites in humans, as an antimicrobial agent on burn victims, to treat umbilical cords of infants, for the treatment of long-term vaginal candidosis, and for various purposes in veterinary medicine (1,2). Crystal violet also is widely used in aquaculture for its effectiveness against fungal and parasite infections on fish. However, because of its mutagenic and teratogenic effects on humans, crystal violet is banned from use in aquaculture (3) and is cautiously applied in medical treatment. In this sense, it is of great interest to perform a quantitative analysis of the compound. Furthermore, as a conventional dye binding to nucleic acid and other components in biological samples, the quantitative analysis of crystal violet can be a reference for the quantitation of the biological components (4).

In most cases, liquid chromatography procedures were used for the quantitative analysis of crystal violet. But the dye usually had to be preconcentrated from the analyzed samples using various extraction procedures, such as magnetic solid phase extraction (MSPE), making it a time-consuming process (5). Thus, developing a real-time tool for the quantitative detection is required.

As we know, Raman spectra usually show narrow bands associated with the analyte, and, in theory, the intensity of an analyte band is linearly proportional to the analyte concentration (6). This makes Raman quantification promising. However, one has to be aware of disturbing features that can jeopardize accurate quantification, such as variation in the laser power and alignment of the sample with respect to the excitation laser and collection optics, meaning that it is difficult to reproduce the absolute intensity of signals. Therefore, obtaining quantitative information requires a standard (internal standard or external standard); the band intensity ratio of the target molecule and the standard is used for quantitative analysis (6–13). An internal standard is either the solvent or an added component. In this study, we quantitatively determined the concentration of crystal violet using acetonitrile (containing a distinct peak at 2260 cm^-1 ) as the internal standard by Raman spectroscopy.

Experimental

Chemicals and Sample Preparation

Crystal violet and acetonitrile were analytical grade and chromatographic grade, respectively. Doubly deionized water was used for all experiments. A stock 2 ×10^-4 M solution of crystal violet was made to prepare the measured sample solutions by dilution.

The total number of mixed aqueous solutions of crystal violet and acetonitrile was 28. Each solution was prepared using a constant concentration of acetonitrile and concentrations of crystal violet ranging from 4.62 ×10^-6 to 1.54 ×10^-4 M. To do this, we first prepared 28 crystal violet aqueous solutions at different concentrations with an equal volume of 1 mL, then added 300 µL of acetonitrile to each sample.

Instrumentation

Raman measurements were performed using a Renishaw (New Mills, UK) inVia micro-Raman spectroscopy system equipped with a 514.5-nm Ar⁺ laser. A Leica microscope objective of magnification 20× was used both to focus the incident laser light on the solution in a capillary tube about 1 mm in diameter, and to collect the back-scattered Raman light with a detection range of 700–2400 cm^-1. Each Raman spectrum is the result of two accumulations of 10 s exposure time. All the data were collected under the same conditions.

Spectra Analysis.

In our study the band intensity ratios, I₁₁₇₉/I₂₂₆₀ and I₁₆₂₂/I₂₂₆₀, were calculated respectively for linear fitting. First, we removed the fluorescence background from the obtained Raman spectra by a baseline correction algorithm implemented in R language and MATLAB (14,15). As a result, the baseline was corrected to zero. Then, we used software Origin 8.0 to read the band intensity (peak height) at 1179 cm^-1 and 1622 cm^-1 of crystal violet and 2260 cm^-1 of acetonitrile, and calculated the ratios.

Results and Discussion

Raman Spectra of Crystal Violet

Raman spectra of solid crystal violet, pure acetonitrile, and one of their aqueous solutions from 700 to 2400 cm^-1 are shown in Figure 1, from top to bottom. It can be seen that the spectrum of mixed solution contains the bands of pure crystal violet and pure acetonitrile and shows lower intensities than those of the pure compound, which is consistent with the theory. Three of the most intense bands, 1179 cm^-1, 1622 cm^-1 of crystal violet, and 2260 cm^-1 of acetonitrile were selected for quantitative analysis. Figure 2 shows the representative Raman spectra of the solutions with the increased concentration of crystal violet from the bottom to top. It is evident that the Raman intensity of all vibrational bands of crystal violet is sensitive to concentration changes and rises with the increasing concentration, as well as the fluorescence background.

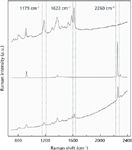
Figure 1: Raman spectra of solid crystal violet (top), pure acetonitrile (middle), and the aqueous solutions of the two mixed (bottom). The concentration of crystal violet is 0.57 ×10-4 M and the volume ratio of acetonitrile to water is 3:10.

Calibration Curves

An illustration of the baseline correction of Raman spectra is shown in Figure 3. It can be seen that the background was successfully removed, and a uniform baseline for all spectra was created. The intensity ratios were plotted against analyte concentrations in Figure 4: Figure 4a for I₁₁₇₉/I₂₂₆₀ and Figure 4b for I₁₆₂₂/I₂₂₆₀. The corresponding calibration curves also are shown in the figure. For each calibration curve, a good linear dependence is observed. The equations of the calibration curves were y = 0.4586x – 0.0115 with R² of 0.9926 for I₁₁₇₉/I₂₂₆₀ and y = 0.6431x – 0.01076 with R² of 0.9960 for I₁₆₂₂/I₂₂₆₀. Note that y and x represent the Raman intensity ratio and the concentration of crystal violet, respectively.

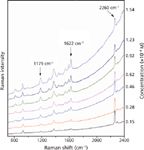
Figure 2: Representative Raman spectra of mixed solutions with different concentrations of crystal violet. Raman bands (at 1179, 1622, and 2260 cm-1) used for quantitative analysis are indicated by arrows.

The result indicates that the intensity ratio between Raman bands of crystal violet and that of acetonitrile provide quantitative information even if the laser power fluctuates or the alignment of the sample changes. Acetonitrile was successfully applied as the internal standard because its intense band at 2260 cm^-1 does not interfere with the crystal violet bands. And more than one band of crystal violet can be used for the quantitative analysis.

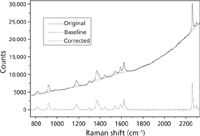
Figure 3: Illustration of the baseline correction of Raman spectra.

In the quantitative analysis, the removal of the background is required for the band intensity ratio calculation. To confirm its necessity, we also studied the relationship between the band intensity ratios and the concentration without background removal, as shown in Figure 5. As can be seen, they do not show linear dependence. Therefore, removing the background is critical for quantitative analysis.

Figure 4: Calibration curves for crystal violet using band intensity ratio, (a) I1179/I2260 and (b) I1622/I2260.

Conclusions

Raman spectroscopy has been tested to quantitatively determine the concentration of crystal violet. It requires an internal standard (acetonitrile) to eliminate factors such as laser power or instrumental effects and a baseline correction of the spectra to obtain the band intensity. Band intensity ratios of crystal violet to acetonitrile have a good linear relationship, with the crystal violet concentration ranging from 4.62 ×10^-6 M to 1.54 ×10^-4 M. Our results demonstrate that the proposed method is quick and accurate and may permit the detection of crystal violet in aquaculture.

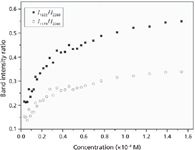
Figure 5: Plots of the band intensity ratios against the concentration without background removal.

Acknowledgments

The authors would like to extend their thanks to Ning Li and Wen Zhuo Yin for their assistance during experiments. This work was supported by the Foundation for the Construction of Strong Province in Traditional Chinese Medicine from Administration Department of Traditional Chinese Medicine of Guangdong Province (No.20112179), the National Natural Science Foundation of China (No. 60778047), the Natural Science Foundation of Guangdong Province (No. 9251063101000009), and Specialized Research Fund for the Doctoral Program of Higher Education of China (No. 200805740003).

Rui Li, Zhou Yi Guo, Zheng Fei Zhuang, and Song Hao Liu are with the MOE Key Laboratory of Laser Life Science & Laboratory of Photonic Chinese Medicine, at the College of Biophotonics, South China Normal University in Guangdong, China. Please direct correspondence to: zhuangzhf@yahoo.com.cn.

Ke Xiong and Si Jin Chen are with the Southern Hospital, at Southern Medical University in Guangdong, China.

References

(1) L.G. Rushing and M.C. Bowman, J. Chromatogr. Sci. 18, 224–232 (1980).

(2) D.J. White, E.M. Johnson, and D.W. Warnock, Genitourinary Med. 69, 112–114 (1993).

(3) S. Culp and F. Beland, J. Am. Coll. Toxicol. 15, 219–238 (1996).

(4) W.J. Zhang, H.P. Xu, S.Q. Wu, X.G. Chen, and Z.D. Hu, Analyst 126, 513–517 (2001).

(5) I. Safarik and M. Safarikova, Water Res. 36, 196–200 (2002).

(6) P.J. Aarnoutse and J.A. Westerhuis, Anal. Chem. 77, 1228–1236 (2005).

(7) Z.P. Chen, G. Fevotte, A. Caillet, D. Littlejohn, and J. Morris, Anal. Chem. 80, 6658–6665 (2008).

(8) J. Cheng, Y.S. Li, R.L. Roberts, and G. Walker, Talanta 44, 1807–1813 (1997).

(9) A.T.G. De Paepe, J.M. Dyke, P.J. Hendra, and F.W. Langkilde, Spectrochim. Acta A. 53, 2267–2273 (1997).

(10) S.G. Skoulika, C.A. Georgiou, and M.G. Polissiou, Talanta51, 599–604 (2001).

(11) M.J. Pelletier, Appl. Spectrosc. 57, 20A–42A (2003).

(12) R. Szostak and S. Mazurek, J. Mol. Struct. 704, 229–233 (2004).

(13) Y. Numata and H. Tanaka, Food Chem.126, 751–755 (2011).

(14) Z.M. Zhang, S. Chen, Y.Z. Liang, Z.X. Liu, Q.M. Zhang, L.X. Ding, F. Ye, and H. Zhou, J. Raman Spectrosc. 41, 659–669 (2009).

(15) Z.M. Zhang, S. Chen, and Y.Z. Liang, Analyst 135, 1138–1146 (2010).