The Big Review II: The Physical Mechanism of Infrared Absorbance and Peak Types

In the second installment of “The Big Review,” we discuss the physical mechanism behind how molecules absorb infrared (IR) radiation. Because light can be thought of as a wave or a particle, we have two equivalent pictures of IR absorbance. We also discuss the quantum mechanics behind IR absorbance, and how this leads to the different peak types observed in IR spectrum.

Years ago, when “lite” beer was introduced in the United States, the original marketing campaign featured two well-known, burly former football players who argued over the advantages of the product as being either “Tastes great” or “Less filling.” The announcer then resolves their conundrum by claiming it is, of course, both. Our concept of electromagnetic radiation, or “light,” as I shall call it from now on, suffers from the same problem as lite beer. For decades, physicists have argued over whether light was a wave or a particle. None other than Sir Isaac Newton advocated for the particle viewpoint (1). An overwhelming number of experiments in the 19th century showed the wave picture was correct, resulting in the discovery of the laws of electromagnetic radiation known as Maxwell’s equations (2). It took Einstein at the beginning of the 20th century to resurrect the idea that light was composed of particles, what we now call photons. It was his work in explaining the photoelectric effect that iced it for photons and won him a Nobel Prize (3).

In the end, like with lite beer, light can be thought of as being “Tastes Great…Less Filling,” or, in reality, both wave and particle. Frankly, I believe this dichotomy in the view of something as fundamental as light means our brains are too puny to really understand what is going on.

All this matters because the purpose of this column is to teach you the physical mechanism behind how infrared (IR) light interacts with molecules so that you can understand where all the features in an IR spectrum originate. Because of the duality of light, it means we have two different pictures of IR absorbance.

The Wave Picture of IR Absorbance

Recall (4) that the proper name for light is electromagnetic radiation, and that light is comprised of two waves propagating in mutually perpendicular planes. One of these waves is called the electric vector, which is shown in part of Figure 1.

FIGURE 1: The light as wave picture of IR absorbance. Note the dipole moment of HCl indicated by the arrow, the alternating polarity electric vector, and how the electric vector pushes and pulls the HCl bond, causing it to become vibrationally excited.

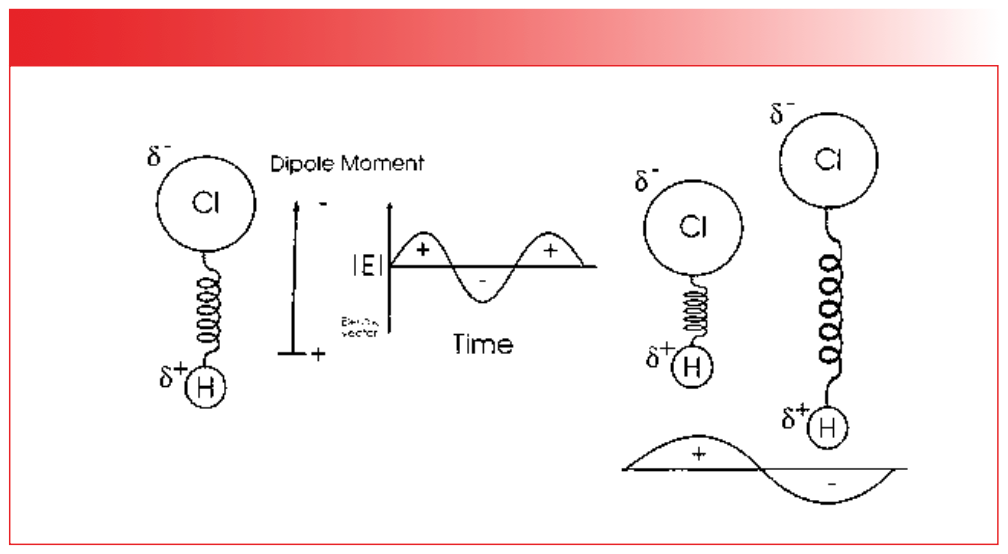
Recall that the electric vector alternates polarity over time from positive to negative to positive.

The molecule we choose to illustrate the wave picture of IR absorbance is hydrogen chloride, HCl, which is illustrated as a ball and spring model as seen in Figure 1. Because of the electronegativity difference between hydrogen and chlorine, the H-Cl bond is polar, with there being a partial negative charge on the chlorine and a partial positive charge on the hydrogen, also illustrated in Figure 1. What we then have is a chemical bond where there are two charges separated by a distance. This condition is called a dipole moment, and it is again a concept we have studied before (5). The dipole moment is a vector quantity, which means it has a magnitude and a direction. The arrow, or vector, in Figure 1 points from the positive to negative charge indicating the direction of the dipole moment, and the length of the arrow indicates its magnitude.

The question we seek to ask and answer here is what happens when an alternating polarity electric vector encounters a dipole moment. This is illustrated to the right in Figure 1. As an example, imagine the positive polarity part of the electric vector encounters the positive end of the H-Cl molecule (again, this is just an example; it could have just as easily encountered the negative end). Remembering the basic law of electromagnetism, that like charges repel and opposite charges attract, the positive polarity electric vector will repel the positive end of the H-Cl bond, causing the bond to shorten, as seen in Figure 1. Now, as we know, the polarity of the electric vector changes over time. So, a moment later when the polarity of the electric vector switches from positive to negative, we will have the opposite situation, a negative polarity electric vector interacting with a partial positive charge. Inevitably, opposites will attract; as a result, the electric vector pulls on the H-Cl bond, lengthening it. Over time, as the electric vector changes polarity, the H-Cl bond will be alternately stretched and contracted—that is, it will become vibrationally excited. Ultimately, energy is transferred from the light wave to the molecule during this process, resulting in a stretching vibration. The reduction in light reaching the detector in our spectrometer at this wavelength will result in a peak in the measured IR spectrum of the molecule. Now, this picture of IR absorbance applies to bonds and molecules with permanent dipole moments. However, molecules such as CO₂, which do not have a permanent dipole moment, can absorb IR light for reasons discussed previously (5). Thus, there you have it, the wave picture of how electromagnetic radiation can excite vibrations in molecules via IR absorbance.

The Particle Picture of Infrared Absorbance

A photon is a particle of light that has energy but no mass. A photon with energy E_p is illustrated to the left in Figure 2.

FIGURE 2: An illustration of a photon of light with energy E_p colliding with a with a methane molecule, represented as a sphere.

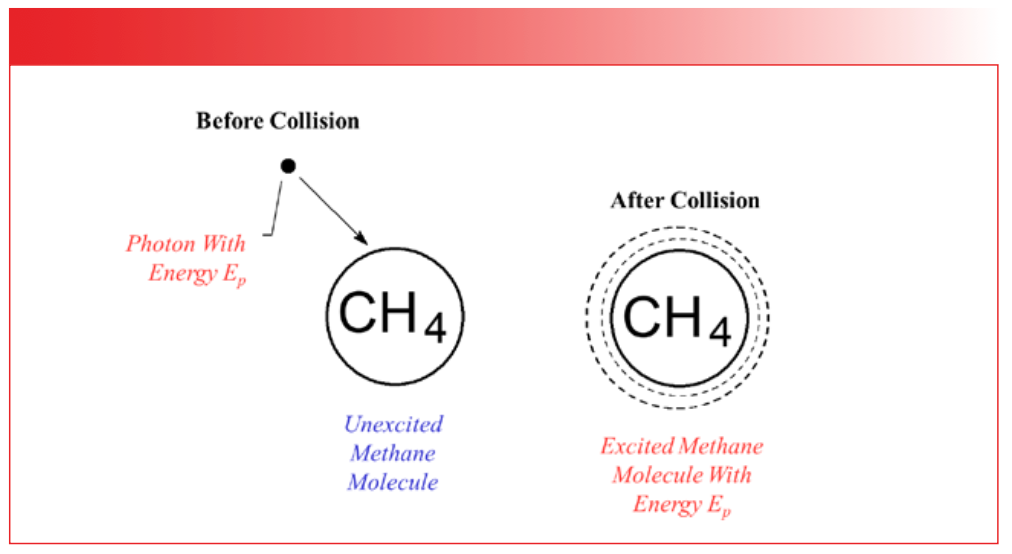
The arrow next to the photon shows it is about to collide with a methane molecule, which is illustrated as a sphere. As a chemist, I am fully aware that CH₄ is tetrahedral and not spherical, but physicists have trouble with this concept, and they find it easier when talking about collisions to treat molecules as spheres, like billiard balls. Note that, to the left in Figure 2, the methane molecule is unexcited, at rest, and has zero vibrational energy.

After the photon collides with the methane molecule, the situation to the right occurs. As a result of what is called a “totally inelastic collision” (6), all the energy in the photon is transferred to the methane molecule as vibrational energy, and the photon ceases to exist. The result of the collision as seen to the right in Figure 2 is that the methane molecule is now vibrationally excited with energy E_p. This is a requirement, since this process, like all physical processes, must obey the law of conservation of energy. As a result of this collision, the resultant reduction in energy at this wavenumber at the detector gives an IR peak in the spectrum of the molecule. You can think of a photon/molecule collision as being similar to that between a hammer and a bell, with the photon as the hammer and the methane molecule as the bell. In this case, the collision causes the methane molecule to vibrate at a characteristic frequency based on its structure, just like a bell would.

The Three Types of IR Peaks

At its root, IR absorbance is a quantum mechanical process. So far in this column series, we have had only hasty brushes with quantum mechanics. I am afraid that has to end for us to be able to address the topic of the three types of IR features. It is a property of microscopic bound systems that their energy levels are quantized. Examples of microscopic bound systems include atomic nuclei, atoms, and, most importantly for us, molecules. Quantized means that the microscopic bound systems cannot have any random amount of energy, but only specific allowed energies. Figure 3 shows the quantized vibrational energy levels for, let’s imagine, the O-H bond in water.

FIGURE 3: The potential energy diagram and vibrational energy levels for the O-H bond in water.

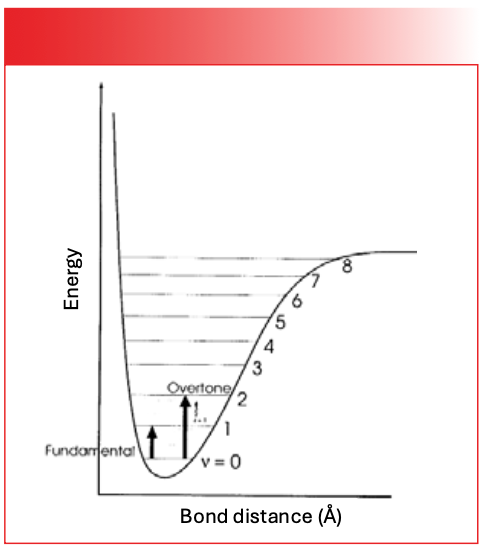
Note that the plot in Figure 3 has energy, which can be measured in wavenumbers, on the y-axis (4), versus bond length on the x-axis. The curve in Figure 3 also shows us the potential energy curve for the O-H bond, and it illustrates what happens when we stretch and contract an O-H bond. At the bottom of the curve, the O-H bond has minimal energy, and a bond length called the equilibrium bond length, which is the bond length when the molecule is vibrationally at rest. As we move to the right in Figure 3, we are lengthening the chemical bond and essentially trying to pull it apart. This, of course, takes energy, hence the plot curves up to the right in Figure 3. Ultimately, if we put enough energy into the bond, it will break apart, which is why the curve flattens out to the right in Figure 3. The difference in energy between the bottom of this plot, or well, and the flat part to the right is the bond’s energy. Breaking bonds in this way is how chemistry happens. To the left in Figure 3, we are contracting the O-H bond away from its preferred equilibrium bond length, which means we are trying to smash two positively charged atomic nuclei into each other. This takes a lot of energy, hence the steep slope to the left of the plot in Figure 3.

The horizontal lines in Figure 3 are the quantized vibrational energy levels for the O-H stretch of the water molecule. Let’s say, for the sake of argument, that the levels are 3400 cm^-1 apart. These vibrational energy levels are given numbers called vibrational quantum numbers, or V, as seen in Figure 3. Naturally, we give the lowest energy level the zero value or V = 0, and then number the levels V = 1,2,3… in ascending energy order. Diagrams like that in Figure 3 can be drawn in theory for any vibration of any molecule or functional group. We stick to the O-H stretch here for simplicity.

At room temperature, most of the molecules in a sample of water are in the ground, or V = 0 state and contain no O-H stretching vibrational energy (this is not strictly correct, as there exists a thing in quantum mechanics called the zero point energy, which we are ignoring to make our lives simpler). The spacing of the vibrational energy levels in Figure 3 is determined by the structure of the molecule. In fact, the spacing depends upon the force constant and reduced mass of the chemical bond, which relates to peak position as we saw last time (5), and is repeated here as equation 1.

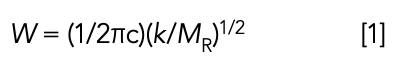
where W equals peak wavenumber position in cm^-1; c equals the speed of light; k equals force constant; and M_R equals reduced mass.

Thus, the ratio (k/M_R)^1/2 is what determines the energy level spacing of the quantized vibrational energy levels in molecules, and the spacing will be different for any two molecules or functional groups with different structures. Equation 1 is, in my opinion, the most important one in IR spectroscopy. It gives the correlation between peak position and molecular structure, it is the reason why if two molecules have different chemical structures they will have different IR spectra, and it is why we can use IR spectra as a chemical fingerprint.

Via one of the processes illustrated above, an O-H bond can absorb 3400 cm^-1 of energy, and be promoted from the V = 0 to the V = 1 vibrational energy level. This type of transition is called a fundamental transition, as seen in Figure 3, and the resultant peak in an infrared spectrum is called a fundamental band. In this case, the result of this transition is a peak near 3400 cm-1 in water, which we have labeled in the past as the “O-H stretching vibration” of water. Part of the IR spectrum of water is seen in Figure 4. The O-H stretching fundamental peak is clearly marked.

FIGURE 4: Part of the IR spectrum of liquid water. The scissors bend is fundamental at 1630 cm^-1, the O-H stretch is fundamental at ~3400 cm^-1, and the O-H stretch/scissors combination band at 5187 cm^-1 is marked.

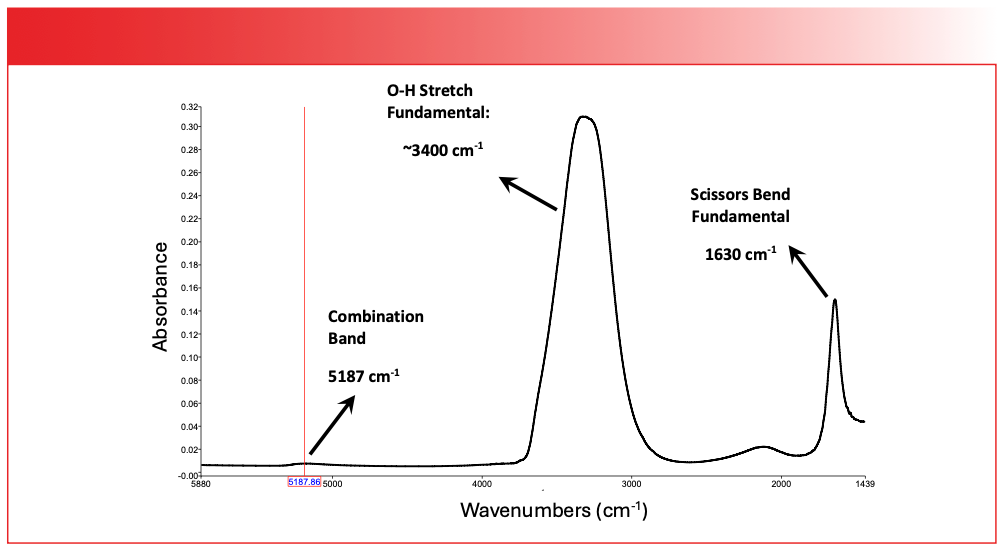
Fundamental peaks are the most common peaks seen in infrared spectra and make up the vast majority of the group wavenumbers we have studied in this course and I have written about (7). Fundamental bands are also the most intense of the three types of peaks that will be presented here, which is why fundamentals are such good group wavenumbers.

Note in Figure 3 that there is an arrow connecting the V = 0 to V = 2 levels. This is because, in this case, water can absorb light at an energy of approximately 6800 cm^-1, and make what is called an overtone transition giving rise to an overtone peak in its spectrum. The term “overtone” refers to any transition from V = 0 to any level greater than one, that is from V = 0 to v = 2,3,4… or any higher level. The V = 0 to V = 2 transition is called the “first overtone”, the V = 0 to V = 3 transition is called the “second overtone” and so on. Overtones are generally found at approximately twice the wavenumber of a fundamental peak because the energy levels in diagrams like Figure 3 are often approximately evenly spaced.

An good example of an overtone peak is shown in Figure 5, which is the IR spectrum of methyl ethyl ketone (MEK), which we have seen before (8).

FIGURE 5: The IR spectrum of methyl ethyl ketone, with examples of fundamental and overtone C=O stretching bands marked.

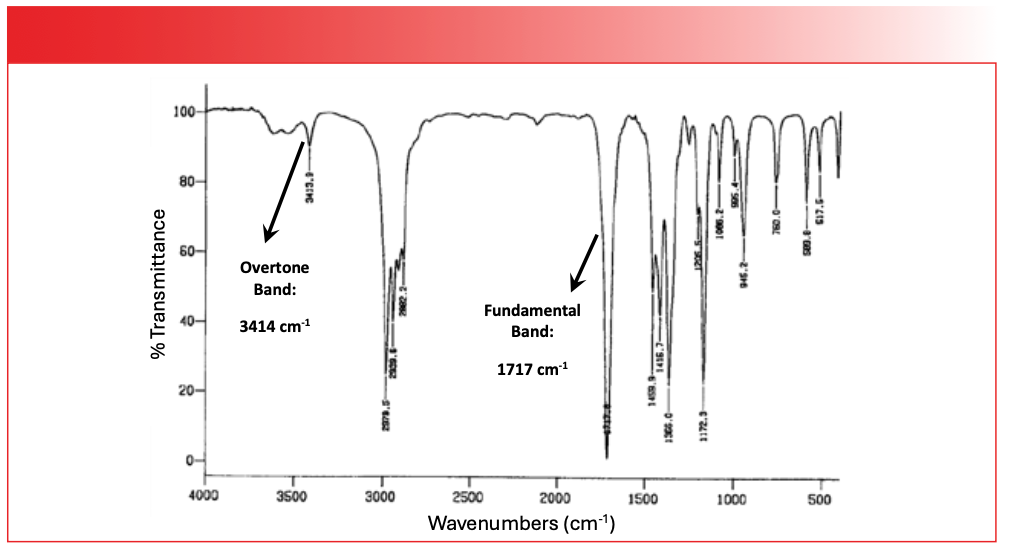
The fundamental C=O stretching peak, arising from the V = 0 to V = 1 transition, falls at 1717 cm^-1 in the spectrum of MEK. Its first overtone arising from the V = 0 to V = 2 transition falls at almost twice this value at 3414 cm^-1. Note how small the overtone peak is compared to the fundamental. For quantum mechanical reasons, I will not go into here, in general overtone peaks are 10–100 times weaker than fundamental bands. This is why they are not good group wavenumbers, and why we have rarely discussed them.

In addition to overtones, when a molecule absorbs infrared light more than one vibration can be excited at the same time. These types of IR features are called combination bands because they involve a combination of vibrations. The infrared spectrum of liquid water, seen in Figure 4, shows examples of how two fundamental bands combine to give a combination band. The water spectrum exhibits a scissors bending fundamental at 1630 cm^-1, and an O-H stretch fundamental at ~3400 cm^-1. The combination band because of the excitation of both these vibrations at the same time is at 5187 cm^-1, as marked in Figure 4. Note how small the combination band is compared to the fundamentals. Also, note that this combination band is in the near-infrared (NIR). Many NIR features are overtone or combination bands. Because they are not intense, combination bands are generally not diagnostically useful.

So, both overtone bands and combination bands are not diagnostically useful, because they are not very intense. In fact, these features make up a lot of the little wiggles and bumps in spectra that we generally ignore. One place where we did find overtone and combination bands to be useful was the Benzene Fingers (9), where we used them distinguish mono-, ortho-, meta-, and para-substituted benzene rings from each other. Also, when we studied aldehydes (8) we saw that via a mechanism called Fermi Resonance an overtone of the aldehydic C-H bend “stole intensity” from the C-H stretch fundamental peak and thus appeared with significant intensity.

Conclusion

Light can be thought of as a wave or particle, giving us two pictures of the physical process behind IR absorbance. In the wave picture, we imagine an alternating polarity electric vector pushing and pulling the partial charges on molecules, thus exciting vibrations. In the particle picture, we imagine a photon of light colliding with a molecule, the photon is absorbed, and the energy causes the molecule to vibrate—kind of like ringing a bell. We concluded by discussing the three types of IR features—fundamental, overtone, and combination bands—and presented their relative utility as group wavenumbers.

References

(1) Corpuscular theory of light. Wikipedia (accessed 2024-09-17)

(2) Maxwell’s equations. Wikipedia (accessed 2024-09-17)

(3) Photoelectric effect. Wikipedia (accessed 2024-09-17)

(4) Smith, B. C. Electromagnetic Radiation, Spectral Units, and Alkanes. Spectroscopy 2015, 30 (4), 18–23.

(5) Smith, B. C. Infrared Spectral Interpretation, In The Beginning I: The Meaning of Peak Positions, Heights, and Widths. Spectroscopy 2024, 39 (4), 18–24. DOI: 10.56530/spectroscopy.fi6379n1

(6) Smith, B. C. Quantitative Spectroscopy: Theory and Practice; Elsevier, 2002.

(7) Smith, B. C. Infrared Spectral Interpretation: A Systematic Approach; CRC Press, 1998.

(8) Smith, B. C. The C=O Bond, Part II: Aldehydes. Spectroscopy 2017, 32 (11), 28–34.

(9) Smith, B. C. The Benzene Fingers, Part II: Let Your Fingers Do the Walking Through the Benzene Fingers. Spectroscopy 2016, 31 (9), 30–33.

Brian C. Smith, PhD, is the founder and CEO of Big Sur Scientific, a maker of portable mid-infrared cannabis analyzers. He has over 30 years experience as an industrial infrared spectroscopist, has published numerous peer-reviewed papers, and has written three books on spectroscopy. As a trainer, he has helped thousands of people around the world improve their infrared analyses. In addition to writing for Spectroscopy, Dr. Smith writes a regular column for its sister publication Cannabis Science and Technology and sits on its editorial board. He earned his PhD in physical chemistry from Dartmouth College. He can be reached at: SpectroscopyEdit@MMHGroup.com●