An Optical Nose Approach to Explosive Detection: One Strategy for Optically Based Sensing

The ideal detection system for threat agents, whether these threats are explosives, chemical and biological agents, or illicit drugs, possesses a low detection limit (in other words, is capable of detecting threat signatures present at only parts-per-billion to parts-per-trillion by volume levels), is able to discern a threat amidst a real world background, and can operate from standoff distances in real time. This last operational criterion is critical whenever the sensor system is used at the front of a rapidly moving convoy, in an airport security or screening device, or in any other domestic application where impeding the flow of commerce is a concern. Unfortunately, a sensor meeting all of these criteria currently does not exist. This is particularly true in the explosive detection arena where the trained canine remains the gold standard.

One of the challenges associated with vapor detection of explosives is that energetic compounds such as 2,4,6-trinitrotoluene (TNT), cyclo-1,3,5-trimethylene-2,4,6-trinitramine (RDX), and pentaerythritol tetranitrate (PETN) have very low vapor pressures at ambient temperatures (3,4). For example, TNT is one of the most volatile energetic materials with a vapor pressure of 10^-5 Torr at 300 K (3–6). Vapor pressures for most other energetic materials are significantly lower. Figure 1 shows plots of the vapor pressures as a function of vapor pressure for a number of explosives, as well as several volatile organic compounds (VOCs). Nitroaromatics (including nitrobenzene [NB], dinitrobenzene [DNB], trinitrobenzene [TNB], mononitrotoluenes [MNT], and dinitrotoluenes [DNT]) are included here as they represent some of the impurities commonly found in TNT-based explosives. Parenthetically, several of these compounds have been identified in the vapor above TNT samples and, in the case of DNT, the initial gas chromatography–mass spectrometry (GC–MS) headspace measurements were reported more than 30 years ago (7).

Figure 1: Plot of vapor pressure (in torr) for several pure explosives, as well as several VOCs commonly found in TNT-based explosives, as a function of temperature. Data for this plot were taken, for the most part, from reference 4. At 300 K, note the vapor pressure range spans almost nine orders of magnitude.

In addition, explosive devices typically are packaged in a plastic or metal container, which further reduces the amount of vapor available for detection purposes. Some experts have suggested that such packaging can reduce the amount of vapor from a device by a factor of 10³ or greater (8). The canine limit of detection varies somewhat with the compound being detected, but it is nominally in the parts-per-billion to subpart-per-billion by volume range at atmospheric pressure conditions (2,5,6). Given the vapor pressures shown in Figure 1, the expected vapor concentration of TNT from a TNT-based device or a deployed landmine will be orders of magnitude less the canine limit of detection at 300 K. Given these low vapor concentrations, how is a trained canine able to successfully detect and locate the presence of a landmine? The answer lies in the concept of the explosive bouquet.

The Explosive Bouquet Concept

The trained canine has been deployed successfully for explosive demining purposes since World War II. However, the systematic selection of explosive compounds to train sniffer dogs and the standardization of canine testing and evaluation procedures were not fully developed until the Bureau of Alcohol, Tobacco, and Firearms initiated its Canine Explosive Detection program in the early 1990s. Over the past decade, researchers at Auburn University, the US Army Corps of Engineers, and Sandia National Laboratories have reported a number of interesting observations regarding not only how trained canines detect hidden explosives, but also the chemical makeup of the vapor signature from such devices (2,3). Based on research at centers such as the Institute for Biological Detection Systems at Auburn University, it appears that dogs do not rely on a vapor signature from the pure explosive material itself, but rather a characteristic combination of odors from solvents, synthetic remnants from the production–material processing steps, and degradation by-products (2,6). Canine researchers refer to this combination of odors as the explosive bouquet (2,6).

Certainly there has been a great deal of high-quality effort devoted to the explosive detection problem over the past decade. Much of this effort has focused on the development of strategies to detect pure energetic materials (9–14).

The explosive bouquet approach thus presents an alternative strategy, first advocated by the canine research community, to addressing the challenges involved in detecting explosives. The explosive bouquet approach involves developing sensors that rely on a set of odors or vapor signatures similar to those used by a trained canine (5,6,9–17). For optically based sensors, high-resolution gas-phase spectroscopic methods, similar to those developed to detect and monitor atmospheric species over the past four decades, represent a logical approach (18). Two particularly promising methods with standoff potential are infrared-enhanced laser-induced breakdown spectroscopy (LIBS) (19,20) and standoff super-radiant spectroscopy (SOS) (21). In the case of super-radiant spectroscopy, researchers at the Arkansas Center for Laser Applications and Science (ArCLAS) are evaluating the potential of an SOS variation known as stimulated Raman adiabatic passage (STIRAP) for standoff detection. It is worth noting that ArCLAS personnel have recently made STIRAP enhanced fluorescence measurements at atmospheric pressure in the picosecond time range (22).

To realize the full potential of laser-based sensing technologies such as STIRAP, an appropriate set of high-resolution spectral signatures must be available for the species or threat agents of interest. Because many of the molecules in the explosive bouquet are common organic compounds, there are high-quality gas-phase infrared spectra available for them through numerous database sources (23–37). However, high-resolution datasets, containing clearly defined rotational structure, are almost nonexistent for many of the highest priority explosive-related VOC compounds. One of the ongoing ArCLAS efforts is the development of such a database for threat agents of interest.

High-Resolution Infrared Spectroscopy and the Explosive Bouquet

High-resolution optical absorption methods have enjoyed considerable success in atmospheric sensing applications due not only to the high level of sensitivity afforded by laser-based techniques, but also the inherent selectivity achievable with rotationally resolved measurements (18). Whereas a low-to-moderate resolution infrared measurement allows a particular class of compound to be identified based on the presence of certain characteristic functional group vibrational modes, a rotationally resolved measurement provides a means to unambiguously identify a specific molecule and, in some cases, even a particular isotopic variation within a molecule. The detection and identification of molecular vapors by such methods is of course predicated upon the ability to obtain a rotationally resolved spectrum of the sample. For larger or even for less symmetrical molecular species, it can be difficult to rotationally resolve and analyze the spectral features. Consider, for example, the two triatomic molecules carbon dioxide and water. From a high-resolution spectroscopic perspective, carbon dioxide is a linear molecule whereas water is an asymmetric top. The consequences of these structural differences are manifested in the gas-phase rovibrational spectra shown in Figures 2a and 2b. For example, the carbon dioxide perpendicular bending mode shown in Figure 2a exhibits the classic P, Q, R rovibrational structure. The spacing between adjacent absorptions in the P and R branches is regular and, assuming one takes into account the nuclear spin (particularly that of ¹⁶ O), quite straightforward to analyze. The corresponding rovibrational band of water (Figure 2b) on the other hand, represents a bit more of a challenge for high-resolution spectroscopists. At first blush, there does not appear to be any sort of readily identifiable pattern. Even so, there are techniques, such as the method of combination differences, that can be used to analyze the complex spectra for this small asymmetric top (38). Indeed, existing databases such as HITRAN contains thousands of water absorptions that have been analyzed and assigned in just this manner (27,28,35,36). Although such techniques have been extended to larger molecules, the spacing between individual rovibrational transitions generally can be said to vary inversely with the molecular moments of inertia. The gas-phase infrared spectrum of acetone for example, a somewhat larger asymmetric top molecule, does not appear to exhibit any identifiable rotational structure, even under jet-cooled conditions (39). Acetone has the additional complicating feature of internal rotation (in the gas phase, the methyl groups are considered nearly free internal rotors), which contributes to the density of rovibrational states.

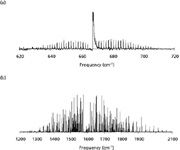
Figure 2: (a) FT-IR spectrum of the ν2 fundamental for carbon dioxide collected at a spectral resolution of 0.125 cm-1. (b) FT-IR spectrum of the ν2 fundamental for water collected at a spectral resolution of 0.125 cm-1.

The bad news, from a molecular spectroscopy perspective, is that many of the explosive bouquet molecules are not only asymmetric tops but also possess internal methyl rotors (for example, the nitrotoluenes). Nevertheless, many asymmetrical rotors exhibit less-complicated rovibrational structure in certain bands. In some cases, this spectral structure is almost symmetric top–like in appearance. Such vibrational bands may in fact be quite useful for atmospheric sensing applications (40).

Laboratory Detection and Infrared Spectral Signature for Isobutylene

The ArCLAS facility has an arsenal of spectroscopic methods for attacking atmospheric sensing problems including a Nicolet Model 8700 FT infrared spectrometer (Thermo Fisher Scientific, Waltham, Massachusetts) and several mid-IR high-resolution laser sources. The laser systems are schematically shown in Figure 3. The two large rectangular boxes defined by the bold black lines represent a 5 ft ×10 ft and a 4 ft × 6 ft optical table, respectively. There are four mid-IR laser sources on the 5 ft × 10 ft table, which is divided basically into two separate measurement channels. Liquid nitrogen–cooled Pb-salt diode lasers along with a recently acquired difference frequency generation laser (Novawave IRIS 1000, Thermo Fisher Scientific) are used to characterize and generate spectral signatures for threat agents primarily in the 3–7 µm range. CaF₂ optics are used exclusively in this channel and an assortment of cryogenically cooled InSb and mercury-cadmium-telluride (MCT) detectors are available depending upon the measurement task. At longer wavelengths, measurement options include either a set of He-cooled Pb-salt diode lasers (mounted in a Laser Components L5731 coldhead, Laser Components IG, Inc., Hudson, New Hampshire) or an external cavity quantum cascade laser (QCL) system from Daylight Solutions (San Diego, California). ZnSe optics and liquid nitrogen–cooled MCT detectors are used with these longer wavelength light sources.

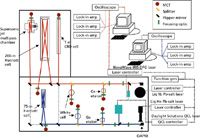
Figure 3: Schematic showing the ArCLAS mid IR laser spectrometers. The solid lines in the figure represent the optical path and the dotted lines represent electrical connections. While these spectrometers could be miniaturized and outfitted for field measurements, they are currently mounted on a 5 ft × 10 ft optical table and suitable for laboratory-based measurements only.

Optical beam paths for the two mid-IR channels were optimized with the following design constraint: They should provide each measurement channel access to as many gas sample cells as possible while ensuring that 3–7 µm and 7–14 µm measurements could be made simultaneously. For example, there are two astigmatic Herriott cells (from Aerodyne Research, Inc., Billerica, Massachusetts) shown in Figure 3. One is a low-volume 75-m absorption pathlength cell and the other is a 200-m absorption pathlength cell with a 5-L internal volume. These two cells are more or less dedicated to a specific measurement channel. Additionally, there is a pulsed supersonic molecular beam sample source and a 1-m cell equipped with electrodes as well as a McCarroll microwave cavity (mounted on a side-port) allowing plasma species to be produced in situ for spectroscopic study. These two additional sample sources can be accessed via strategically positioned flipper mirrors.

Each measurement channel has a dedicated PC that is interfaced to a number of devices including laser controllers, a LeCroy 6340 Waverunner DSO (LeCroy Corp. Chestnut Ridge, New York), three SRS 510 lock-in amplifiers (Stanford Research Systems, Inc., Sunnyvale, California), three SRS 560 preamps, a SRS DS 345 function generator, an MKS PR 400 Baratron gauge controller (MKS Instruments, Andover, Massachusetts), and an MKS 647 multigas flow controller. The last two devices are necessary to control the amount of sample gas input for analysis as well as for monitoring the overall pressure in the sample cell during measurement. The instrumental interface is accomplished using in-house routines developed on the LabVIEW platform (National Instruments Corp., Austin, Texas). Spectra are typically collected using a modulation detection scheme. Within this scheme, the laser is scanned, via an externally generated voltage ramp, through its free spectral range (for diode lasers this corresponds to a single longitudinal lasing mode 1–3 cm^-1 in length). Superimposed on this voltage ramp is a frequency modulation of 5–10 kHz. This frequency modulation can be input to the lock-in amplifiers as a reference, and the individual spectral lines are detected as either the first or second derivative of the absorption signal.

Given the available resources within ArCLAS, a spectroscopic strategy has been adopted that is similar to that described by Jennings (41). The central idea is to take advantage of the complementary nature of Fourier transform (FT)–based and mid-IR laser–based spectrometers. Moderate resolution Fourier-transform infrared (FT-IR) instruments (0.125 cm^-1 ), for example, possess a broadband scanning capability useful not only for obtaining the entire IR spectrum of a single gas-phase species or vapor mixture quickly, but they can also provide insight into specific spectral regions with potential for atmospheric sensing applications. One disadvantage of these broadband thermal source instruments, however, is an inability to resolve rotational structure, particularly for the molecules of interest here. The gas-phase infrared spectrum for isobutylene provides an explicit example of how FT-IR and laser-based spectrometers can be used to great advantage (41).

In addition to TNT-based materials, there are a number of priority targets within the context of the explosive detection problem. One such target is the military-grade high explosive known as composition C4 or simply C4. C4 is an RDX-based explosive with a detonation velocity 1.3 times that of TNT (4). A recent headspace solid-phase microextraction (SPME)–GC–electron capture detection (ECD) study suggests that cyclohexanone and 2-ethyl-1-hexanol are two components of the C4 vapor bouquet (42). However, the C4 formulation also contains plasticizer and binder materials. The binder material is often a synthetic rubber produced by polymerizing isobutylene with a small amount of isoprene. Polyisobutylene, or butyl rubber, is a gas-impermeable material capable of retaining air for long periods of time. In addition to endowing the explosive composition with a certain amount of water resistance, this property could trap any unreacted isobutylene left from the polymerization process and allow it to outgas slowly over time. Following the canine nose example that volatile impurities, and not the energetic material itself, are most effective for detecting the presence of explosives, isobutylene may prove useful for explosive sensing applications.

Isobutylene has a molecular formula of C₄H₈ and is structurally similar to acetone (in acetone the methylene group is replaced by an oxygen atom). As part of the explosive bouquet spectral signatures effort, we have begun examining the gas-phase infrared spectrum of this molecule at various sample cell pressures as well as different levels of spectral resolution. These two experimental parameters are linked, in that elevated sample pressures will tend to broaden the rovibrational structure. Given a nominal pressure broadening coefficient of ~10 MHz Torr^-1 for example, an observed linewidth of 0.2 cm^-1 or better would be expected for a gas sample at 1 atm total pressure (18). Experiments designed to yield rotational structure for larger molecules, which typically possess a significant density of states, are therefore not practical at sample pressures above a few torr. For the FT-IR measurements here, an isobutylene pressure of 175 mTorr was empirically determined to be optimal.

An IR spectrum for isobutylene, collected at this optimal gas sample pressure, is shown in Figure 4a. Based on integrated absorbance, the vibrational bands in the 3-µm region appear to represent some of the most intense spectral features. This region corresponds to the C-H stretching region (many of the volatile bouquet species exhibit resonances in this region) and although the absorption features do not appear to be overly complex, the low-resolution contour masks several interesting spectroscopic features (including several vibrational modes in the 2900–3000 cm^-1 region). Portions of this CH stretching region have been previously examined in some detail. For example, the a-type asymmetric top vibrational band at 3087 cm^-1 has been investigated both at room temperature (43) and by molecular beam methods (44). Based on the molecular beam measurements, a spectral density of ~100 states/cm^-1 was reported for the rotationless 0₀₀ vibrational state (44). In these previous studies, the large number of states was attributed to the torsional motion associated with the two methyl rotors, which essentially act to produce a fractionation of the rovibrational energy levels (39,44).

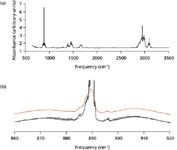
Figure 4: (a) FT-IR spectrum for a 175-mTorr isobutylene gas sample at an instrumental resolution of 0.125 cm-1. The broad intense resonances in the 3-µm region correspond to a C-H stretch. Isobutylene actually has two different types of C-H functionality: one associated with the two methyl groups and one associated with the CH2= portion of the molecule. The broader more intense red shifted structure arises from vibrations involving the two methyl groups. (b) The ν28 rovibrational band of isobutylene centered near 890 cm-1. The red trace was collected at an instrumental resolution of 0.5 cm-1, the black trace 0.125 cm-1. This band appears to have a rovibrational structure similar to a parallel band of an oblate symmetric top. Note all measurements were performed on an isobutylene gas sample taken from a Matheson lecture bottle and used without further purification.

We have performed a number of laser-based high-resolution scans in the 2900–3000 cm^-1 region and found that even a fairly dilute isobutylene gas mixture is sufficient to completely attenuate the entire longitudinal lasing mode from either a Pb-salt diode or a difference frequency generation laser. Lower isobutylene concentrations simply produce a proportionally lower attenuation of the mode. Combined with results from the previous studies (39,44), it appears the sheer spectral density acts to prohibit observation of rotational structure at room temperature. From an optical sensing perspective then, the utility of the 3-µm region for isobutylene detection appears quite limited.

While molecular beam methods are required to see rotational structure in the isobutylene signature near 3 µm, there are several vibrational bands for which rotational structure can be observed at room temperature. Consider, for example, the ν₂₈ mode centered near 890 cm^-1 shown in Figure 4b. As with the 3087-cm^-1 band, there is a well defined P, Q, and R branch rovibrational contour, although the P and R branch regions in Figure 4b appear to exhibit definite rotational structure at a spectral resolution of 0.125 cm^-1 and a sample pressure of 175 mTorr (given by the black trace in Figure 4b). The spacing between adjacent transitions here is approximately 0.5 cm^-1 and the overall rovibrational structure is somewhat reminiscent of a parallel band for a symmetric top (38,45). Given this spacing, it is not too surprising that rotational structure is not observed at a lower spectral resolution, even at this optimal sample pressure (the red trace in Figure 4b corresponds to a spectral resolution of 0.5 cm^-1 point^-1 ).

The selections rules for a parallel band of a symmetric top are ΔJ = 0 ± 1 and ΔK = 0 where J represents the quantum number associated with end-over-end rotation of the molecule and K is the quantum number for rotation about the internal top axis. Rovibrational spectra for asymmetric tops are typically quite different than those exhibited by symmetric tops. By definition, asymmetric tops have three unique molecular rotational axes conventionally labeled a, b, and c. Although all infrared selection rules require a change in dipole moment, in the case of an asymmetric top, this change can occur along the a-, b- or c-axis. Thus there is the possibility of an a-type, b-type, or c-type spectrum each with a slightly different set of rovibrational selection rules (38,45). Moreover, there is also the possibility of hybrid vibrational bands in which the transition moment lies in a plane defined by two of these axes. For example, a transition moment in the bc-plane could result in a condition where both b-type and c-type selection rules apply (38,45). An additional complicating factor is that K is no longer a good quantum number (38,45). Instead, the parameters K_obl and K_pro are introduced to describe how the asymmetric top rovibrational levels correlate to the value of K in the oblate and prolate symmetric top limits. For such molecules, the individual rovibrational levels are typically labeled using a J_KoblKpro scheme (38,45).

Highly irregular patterns in asymmetric top rovibrational spectra then are not unexpected and the vibrational band of water in Figure 2b undoubtedly represents such a case. However, it is also true that for certain (limiting) values of J, K_obl, and K_pro, asymmetric tops can exhibit symmetric top-like spectra (45). The rovibrational band in Figure 6 appears to represent an almost pedagogical example of this other extreme, particularly in the P and R branch regions. In fact, as a starting point, we have assigned and fit J quantum numbers to these transitions using the standard energy expression

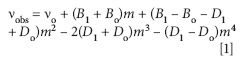
where ν_o is the vibrational band origin and B₁ and B_o are rotational constants for the ground and excited vibrational states (38,45). D₁ and D_o are the corresponding centrifugal distortion constants. The parameter m in equation 1 is a running number defined as m = J + 1 for an R branch line and m = –J for a P branch line. Although the application of equation 1 is normally reserved for the rovibrational spectrum of a simple diatomic molecule, the regular spacing between adjacent absorption lines in the P and R branches made a tempting target, at least for a preliminary evaluation. In any event, almost 40 rovibrational lines were included in the fit to equation 1 with most of the obs-calc values falling within the uncertainly of the measurement (±0.125 cm^-1 ).

At higher spectroscopic resolution, the absorptions, which appear to be individual peaks in Figure 4, take on a far more complicated appearance. Figure 5 shows a representative high-resolution (0.0003 cm^-1 /point) absorption trace, obtained with a 4215 ppm isobutylene–argon gas mixture at total sample pressure of 215 mTorr, is shown. Note, the individual transitions in Figure 5 are quite intense, so much so that frequency modulated detection schemes were unnecessary. A helium-cooled Pb-salt diode laser, temperature tuned to produce radiation at 888 cm^-1 , was used to obtain this scan. To date, we have collected a series of perhaps a dozen such scans across the frequency range 880–923 cm^-1 . As each individual trace can contain upwards of 20 absorption features, it is not surprising that over 200 isobutylene transitions have been measured and cataloged in this manner.

Figure 5: High-resolution trace from a helium-cooled Pb-salt diode laser centered near 888 cm-1. The absorptions in these traces are due to an isobutylene–nitrogen gas mixture (mixture contained 4215 ppm isobutylene at 2000 Torr) at a total sample cell pressure of 800 mTorr.

To be honest, attempting to rigorously assign quantum numbers to all of the structure in Figure 5 can be challenging even without the presence of freely rotating methyl groups. Parenthetically, the energy levels for methyl group rotation are typically labeled by introducing additional angular momentum quantum numbers to represent the amount of energy associated with torsional motion of the methyl rotors. One strategy for such molecules is to initially identify only those absorptions associated with the ground torsional state as these transitions would be expected to exhibit a rigid-rotor-like pattern (46). Although this approach does simplify the problem, we must still consider a rigid asymmetric rotor. Simulation programs (PGOPHER 6.0 and Jet Beam 95 v1.02.4) were used to generate rigid rotor stick spectra by systematically varying the A, B, and C rotational constants for the excited v.al state (47,48). As each set of simulated stick spectra is overlaid on the empirical ν₂₈ band data, potential matches between calculated and observed spectra are identified and quantum number assignments are made. This information (quantum number assignments and associated transition frequencies) can then be incorporated into a nonlinear least squares fitting program for further evaluation. Figure 6 shows representative plots illustrating this comparative spectroscopic analysis. Although these preliminary spectral assignments need to be solidified (using combination differences perhaps), we are now working to identify some of the many transitions, observed and cataloged via Pb-salt diode laser measurements, associated with the excited torsional states.

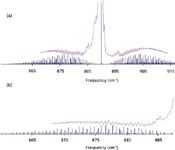
Figure 6: (a) The observed ν28 rovibrational band for isobutylene shown together with a stick spectrum generated assuming a rigid asymmetric oblate rotor (25). In generating this simulation, only a single value of Kpro, Kobl was considered and any centrifugal distortion or torsional effects are completely ignored. (b) An expanded view showing the comparison between the observed ν28 P branch and the simulated stick spectrum.

Assuming quantum numbers can be assigned to the transitions and other fundamental information such as transition moment and pressure broadening data is available, the absolute concentration of species producing the absorption signal can be determined. There are a number of other instrumental analytical techniques that also rely on some sort of optical measurement to quantify components in a sample. In many of these methods, a standard curve is first generated using a series of samples with well-known concentrations to gauge instrumental response. Provided the response function is linear with respect to concentration, the concentration for an unknown sample can be determined through interpolation with the curve. At this point, the pressure broadening coefficients and transition moments are not available so we have simply measured instrumental response at a number of different isobutylene gas pressures. These measurements are summarized in Figures 7a and 7b. Figure 7a shows two Pb-salt diode laser scans of the same spectral region with isobutylene gas concentrations representing the two extremes of a standard Beer's law curve. The absorptions shown in black correspond to a more concentrated gas sample (1.38 × 10¹⁴ isobutylene molecules/cm³ ). The isobutylene number density for the measurement shown in red was 2.19 × 10¹² molecules/cm³ . When plotted as a function of line center intensity (for the three main spectral absorptions), the resulting standard curve is fairly linear possessing an R² value of 0.992 (see Figure 7b). Note, the concentration range for this preliminary limit-of-detection (LOD) study varied from a high of 6 ppmv isobutylene to a low of 65 ppbv. Certainly, the LOD can be extended by incorporating a frequency modulation scheme and employing lock-in detection methods.

Figure 7: (a) Pb-salt diode laser traces showing a set of absorptions obtained with different isobutylene gas mixtures. The black trace represents a gas mixture with an isobutylene concentration of 16.9 ppm. The gas mixture corresponding to the red trace had an isobutylene concentration of 80.0 ppb. Center frequency for these measurements is 910 cm-1 based on a series of temperature tuned mode characterization studies. (b) A plot of measured line center intensity as a function of isobutylene number density. Over the 17–0.05 ppm concentration range, the measured line center intensity is fairly linear for the more dilute mixtures.

Conclusion

Extending the canine research community concept of focusing on the vapor signature used by explosive- trained dogs, we have begun developing a database of high-resolution spectral signatures for a series of VOCs that represent impurities commonly found in TNT-based explosives. In the IR, the broadband scanning capability of an FT-IR spectrometer is used to locate the band positions as well as to provide a preliminary means for evaluating the appropriateness of an individual rovibrational band for optical sensing measurements. Higher resolution measurements are then obtained with a combination of Pb-salt diode lasers, a DFG laser system, and an external cavity QCL.

For other military-grade explosives such as C4, the canine nose concept has led to similar spectroscopic investigations of other potential volatile impurities including isobutylene. For isobutylene, which is an asymmetric top structurally similar to acetone, the IR experiments not only proved interesting from a fundamental spectroscopic perspective, but also provide an excellent example of how high-resolution spectroscopic methods can be used to identify appropriate vibrational bands for sensing applications.

Acknowledgments

This work is supported by the U.S. Army Night Vision and Electronic Sensors Directorate under contract #W909MY-09-C-0001. We would like to thank Dr. Susan Allen and Dr. Bruce Johnson for useful technical discussions. In addition, we would like to thank the reviewers for not only the insightful comments, which only served to strengthen the manuscript, but also for pointing out an important oversight in the original draft.

References

(1) http://www.darpa.mil/dso/index.htm.

(2) A. Goth, I.G. McLean, and J. Trevelyan, in Mine Detection Dogs: Training, Operations, and Odour Detection, I.G. McLean (Ed) (GICHD, Geneva, 2003), pp. 195–208.

(3) D.S. Moore, Review of Scientific Instruments 75(8), 2499–2512 (2004).

(4) R. Meyer, J. Kohler, and A. Homberg, Explosives, 6th Edition (Wiley-VCH, Weinheim, 2007).

(5) M. Krausa, "Vapor Detection of Explosives for Counter-Terrorism," in Vapor and Trace Detection of Explosives for Anti-Terrorism Purposes, NATO Sciences Series II: Mathematics, Physics, and Chemistry (Kluwer Academic Publishers, Dordrecht, The Netherlands, 2004), pp. 1–9 and references therein.

(6) M. Stancl, "Detection of Traces of Explosives by Means of Sniffing Dogs," in Vapor and Trace Detection of Explosives for Anti-Terrorism Purposes, NATO Sciences Series II: Mathematics, Physics, and Chemistry (Kluwer Academic Publishers, Dordrecht, The Netherlands, 2004), pp. 66–77 and references therein.

(7) T.F. Jenkins, D.C. Leggett, and T.A. Ranney, Vapor Signatures from Military Explosives, US Army Corps of Engineers Special Report, 99-21 (1999).

(8) P. Kolla, Angew. Chem. Int. Ed. Engl. 36, 800 (1997).

(9) J. Wormhoudt, J.H. Shorter, J.B. McManus, P.L. Kababian, M.S. Zahniser, W.M. Davis, E.R. Cespedes, and C.E. Kolb, Appl. Optics 35, 3992–3997 (1996).

(10) J.I. Steinfeld and J. Wormhoudt, Ann. Rev. Phys. Chem. 49, 203-232 (1998).

(11) M.W. Todd, R.A. Provencal, T.G. Owano, B.A. Paldus, A. Kachanov, M. Hunter, S.L. Coy, J.I. Steinfeld, and J.T. Arnold, Appl Phys. B 75, 367–376 (2002).

(12) D. Heflinger, T. Arusi-Parpar, Y. Ron, and R. Levi, Optics Communications 204, 327–331 (2002).

(13) M.B. Pushkarsky, I.G. Dunayevskiy, M. Prasanna, A.G. Tsekoun, R. Go, and C.K.N. Patel, PNAS 103, 19630–19634 (2006).

(14) C.A. Munson, J.L. Gottfried, F.C. De Lucia, Jr., K.L. McNesby, and A.W. Miziolek, Laser-Based Detection Methods for Explosives, ARL-TR-4279 (2007).

(15) T.M. Swager, in Electronic Noses & Sensors for the Detection of Explosives,NATO Science Series II. Mathematics, Physics and Chemistry, J.W. Gardner and J. Yinon, Eds. (Kluwer Academic Publishers, The Netherlands, 2004), pp. 29–37.

(16) C. Cumming, M. Fisher, and J. Sikes, in Electronic Noses & Sensors for the Detection of Explosives,NATO Science Series II. Mathematics, Physics and Chemistry, J.W. Gardner and J. Yinon, Eds. (Kluwer Academic Publishers, The Netherlands, 2004), pp. 53–69.

(17) M. Fisher, and J. Sikes, in Electronic Noses & Sensors for the Detection of Explosives,NATO Science Series II. Mathematics, Physics and Chemistry, J.W. Gardner and J. Yinon, Eds. (Kluwer Academic Publishers, The Netherlands, 2004), pp. 117–130.

(18) Air Monitoring by Spectroscopic Techniques, M. Sigrist, Ed. (John Wiley and Sons, New York, 1994).

(19) D.K. Killinger, S.D. Allen, R.D. Waterbury, C. Stefano, and E.L. Dottery, Optics Express 15, 12905–12915 (2007).

(20) S. Wallin, A. Pettersson, H. Ostmark, and A. Hobro, Anal. Bioanal. Chem. 395, 259–274 (2009).

(21) V. Kocharovsky, S. Cameron, K. Lehmann, R. Lucht, R. Miles, Y. Rostovtsev, W. Warren, G.R. Welch, and M.O. Scully, PNAS 102(22), 7806–7811 (2005).

(22) J. Bruce Johnson, S. Allen, D.R. Britton, J. Burdin, J.L. Hicks, K. Lyon, and W.D. Murray, Chemical, Biological, Radiological, Nuclear and Explosives (CBRNE) Sensing X, SPIE Proceedings 7304, 73040U1-9 (2009).

(23) Compendium of Methods for the Determination of Toxic Organic Compounds in Ambient Air, Second Edition, EPA/625/R-96/010b (1999).

(24) U.S. Environmental Protection Agency (EPA) Method-320, Measurement of Vapor Phase Organic and Inorganic Emissions by Extractive Fourier Transform Infrared Spectroscopy (1999).

(25) L.R. Brown, M.R. Gunson, R.A. Toth, F.W. Irion, C.P. Rinsland, and A. Goldman, Appl. Opt. 35, 2828 (1996).

(26) N. Jacquinet-Husson et al., J. Quant. Spectrosc. Radiat. Transfer 62, 205 (1999).

(27) N. Husson, B. Bonnet, N.A. Scott, and A. Chedin, J. Quant. Spectrosc. Radiat. Transfer 48, 509 (1992).

(28) L. S. Rothman et al., J. Quant. Spectrosc. Radiat. Transfer 60, 665 (1998).

(29) Midac Corporation, Standards: Gas Phase Infrared Spectral Standards, A collection of approximately 150, vapor phase, infrared spectra supplied in digital format. Midac Corporation, Irvine, California; available at http://www.midac.com.

(30) P. Hanst, Infrared Spectra for Quantitative Analysis of Gases, Infrared Analysis Inc., A collection of 296 vapor phase infrared spectra supplied in digital format. Infrared Analysis Corporation, Anaheim, California; available at http://www.infrared-analysis.com.

(31) NIST/EPA Gas-Phase Infrared Database; available at http://www.nist.gov/srd/nist35.htm

(32) The Aldrich Library of FT-IR Spectra Vapor Phase, Edition 1, Vol. 3, C.J. Pouchert, Ed. (The Aldrich Chemical Comp. Inc., Milwaukee, Wisconsin, 1989).

(33) S.W. Sharpe, T.J. Johnson, R.L. Sams, P.M. Chu, G.C. Rhoderick, and P.A. Johnson, Appl. Spectrosc. 58, 1452–1459 (2004). Available on the web at: http://nwir.pnl.gov

(34) L.S. Rothman et. al., J. Quant. Spect. and Rad. Transfer 96, 139–204 (2004).

(35) L.S. Rothman et al., J. Quant. Spectrosc. Radiat. Transfer 110, 533–572 (2009).

(36) N. Jacquinet-Husson et al., J. Quant. Spectrosc. Radiat. Transfer 109, 1043–1059 (2008).

(37) T.J. Johnson, R.L. Sams, S.D. Burton, and T.A. Blake, Anal. Chem. and Biochem. 395, 377–386 (2009).

(38) J.I. Steinfeld, Molecules and Radiation, 2nd Edition (Dover Publications, New York, 2005).

(39) V.A. Walters, S.D. Colson, D.L. Snavely, and K.B. Wiberg, J. Phys.Chem. 89, 3857–3861 (1985).

(40) M.S. Zahniser, D.D. Nelson, Jr., J.B. McManus, and P.L. Kebabian, Phil. Trans. Royal Society London A 351, 371–382 (1995).

(41) D.E. Jennings, J. Quant. Spectrosc. Radiat. Transfer 40, 221–238 (1988).

(42) K.G. Furton and L.J. Meyers, Talanta 54, 487–500 (2001).

(43) C.M. Pathak and W.H. Fletcher, J. Mol. Spect. 33, 32–53 (1969).

(44) D.A. McWhorter and B.H. Pate, J. Mol. Spect. 193, 159–165 (1999).

(45) J.M. Hollas, High Resolution Spectroscopy, 2nd Edition (Wiley, New York, 1998).

(46) A. Hazra, P.N. Ghosh, and R.J. Kshirsagar, J. Mol. Spec. 164, 20–26 (1994).

(47) PGOPHER, a Program for Simulating Rotational Structure, C.M. Western, University of Bristol, http://pgopher.chm.bris.ac.uk

(48) Jet Beam 95 Spectral Fitting Program v1.02.4, D.F. Plusquellic, http://physics.nist.gov/Divisions/Div844/staff/Gp6/plusquellic.html.

Tabetha Osborn, William A. Burns, Joshua, Green, and Scott W. Reeve are with the Department of Chemistry and Physics and the Arkansas Center for Laser Applications and Science (ArCLAS), Arkansas State University, Jonesboro, AR 72401. The author of correspondence (Scott Reeve) can be reached at SREEVE@astate.edu